As per my question i don't understand why during the computation of the field between the inner and outer cylinder we just take into consideration the inner cylinder charges and neglect those of the outer shell?
some people argue that it's due to gauss theorem, but we also have the superposition principle so what'sthe point here?
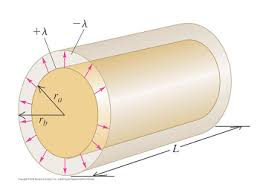
As you pointed in your question, this is because of Gauss theorem. From the definition of this theorem, once you have a closed surface the flux of the electric field through it is proportional to the charge in the volume in that surface. From the symmetry of this problem, you can easily solve it using a cylindrical surface inside which you have only one cylinder of the capacitor.
From my perspective, the charge on the outer shell of the capacitor is a consequence of the presence of charge on the inner shell (and vice versa), therefore you just need to take into account the effects of one of them. In fact, the charge on one surface induces the charge on the other.
