0
$\begingroup$

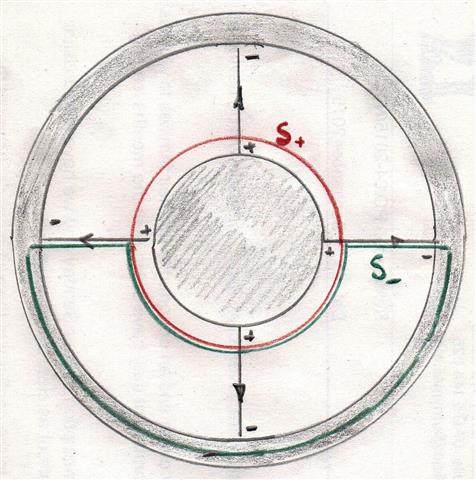
When getting the electric field between the outer and inner cylinder. why is it that we are only concerned with the field generated by the positive charge distribution on the inner surface and not also with the induced negative distribution on the outer cylinder surface. Doesn't this induced negative charge also create a field ? that is, shouldn't we multiply the field calculated using gauss on the inner cylinder by 2 ? (whereas negative charges would exert a field of the same direction and magnitude of the positive inner cahrges).

Is it because when you take the outer cylinder by itself without the inner with the same negative charge distribution on its inner surface (applying superposition) you get a cylinder with symmetrical charges distributed on its surface that cancel each other's effect? Although it seems that charges effect is only symmetrical at the center of the cylinder.

Improve this question
$\endgroup$

2 Answers 2

Reset to default
2
$\begingroup$

The electric field between the conductors is due to both sets of charges. however when finding a value for the electric field using Gauss's law it is only the charges inside the surface which are of interest and it is easier to choose the charge on the centre conductor and the red Gaussian surface $S_+$ which would be a cylider.

You could find the electric field by using the green Gaussian surface $S_-$ but it is perhaps just a little less convenient to do that?
The surface $S-$ could have been bounded by two circles in the diagram but I thought it might be easier to visualise the surface as drawn?

enter image description here

Improve this answer
$\endgroup$
2
  • $\begingroup$ Appreciate your answer, but many references state that the electric field is only that of the positive charge distribution on the inner cylinder. I agree with getting the negative charges contribution to the electric filed by gauss as you stated, but it seems that the negative charges do no exert an electric field. Isit possible that it's because when you take the outer cylinder by itself without the inner with the same negative charge distribution on its inner surface (applying superposition) you get a sphere with symmetrical charges distributed on its surface that cancel each other's effect? $\endgroup$
    – Slango
    Commented May 7, 2016 at 15:03
  • $\begingroup$ It is true that with no outer conductor you would get radial field lines and spherical equipotential surfaces. So putting a spherical conductor where there is an equipotential surface does not change the electric field pattern. The outer conductor is usually grounded to shield the inner conductor from stray fields. $\endgroup$
    – Farcher
    Commented May 7, 2016 at 15:49
1
$\begingroup$

The electric field due to the outer cylinder has no contribution inside. One way to view it using Gauss's law, the other way is that if you took a slice from that cylinder, and considered a point inside it other than the center, you'll find a point producing electric field in the near side of the point (small charge, small distance) and a corresponding arc of the circle that produces an electric field at that point (more charges, bigger distance) and they exactly cancel each other.

E = 0 inside a sphere.

The same applies for a sphere or other shapes as well.

Improve this answer
$\endgroup$
2
  • $\begingroup$ great ! just to make sure i got it right, so adding a point charge inside the cylinder would result in a charge distribution on the surface of the cylinder that would cancel each other?? because the outer cylinder is grounded it can supply charges that would always balance the system inside the cylinder and result in a zero electric field?! thanks a lot. $\endgroup$
    – Slango
    Commented May 7, 2016 at 15:33
  • $\begingroup$ Yes, even if there are some charges distributed on the surface without a point charge inside. but it has to be a conductor because this is not the case for the insulators. $\endgroup$
    – Parity Violator
    Commented May 7, 2016 at 15:51

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.