Electric Field from charged sphere within another charged sphere does not reinforce?

Question

Lets say I have a positively charged conducting sphere of Q. The electric field outside the sphere is

$$E = \frac Q{4\epsilon\pi r^2}.$$

Now suppose this sphere is enclosed inside another hollow conducting sphere of -Q charge. By Gauss law, the electric field is still the same?

Won't the negatively charged outer sphere reinforce the electric field, causing it it be larger?

In another example,

The field from a infinite sheet of charge is

$$ E = \frac \sigma{2\epsilon}. $$

But the field between 2 oppositely charged infinite sheets is

$$ E = \frac \sigma{\epsilon}. $$

Why is this not the case above?

Answer 1

It sounds like the math is not the problem. It is visualizing the geometry.

A point charge fills all space with an electric field. If you have multiple charges, they all create electric fields. To find the electric field at a point, add them up.

Do this with a sphere. If you are outside as shown above, all the E field contributions are more or less to the right. It works out just the same as a point charge E field. If you are inside, contributions from the left and right cancel perfectly. The total is 0. This property of the inverse square law has always struck me as one of the coolest things in physics.

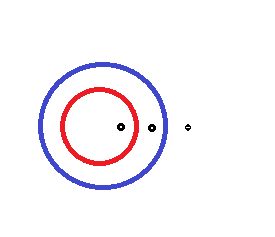
With two spheres, the inner point has 0 field because contributions from the red sphere cancel and so do contributions from the blue sphere.

For the middle point, the red sphere adds up to the same as a point charge, but the blue sphere contributions add to 0.

For the outer point, each sphere adds up to the equivalent of a point charge. In this case two opposite point charges at the same point would cancel.

For a plane, by symmetry the contributions add up to an E field perpendicular to the plane. The plane is infinite, and so the charge is infinite. But some is infinitely far away. The E field works out to be finite and uniform.

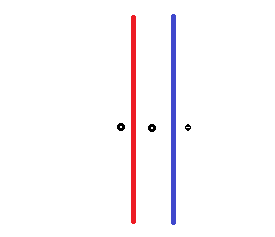
For two oppositely charged planes you get 0 on the outside and reinforcement in between. This is indeed similar to the two spheres, even though there is no charge off to the left.

---

Imagine the two spheres getting larger and larger, so the geometry looks more and more like two planes. The E field should approach the same solution as the two planes.

The left hand point is always inside two spheres. The right hand point is outside of two large spheres that always cancel. So the field is always 0 for them.

For the middle point, the red sphere matters. For the spheres to become like the planes, we must keep the charge density constant as they grow. This means the total charge grows larger. The total red E field is equivalent to a larger, but more distant point charge.

If the radius is r, the total charge, Q, is proportional to $r^2$, and the field is proportional to $Q/r^2$. So the field doesn't change. The E field becomes more uniform, and more like the plane.

Answer 2

The field inside a conducting sphere due to the charge on that sphere is zero. You are left with only the charge on the inner sphere determining the electric field between the spheres. This is a direct consequence of Gauss' Law - it is only the charge enclosed inside the surface over which you integrate that contributes to the field across the surface. The rest follows from superposition.