Why does a surface always exert force normal to it?

Question

In whichever angle an object is thrown at a surface, the surface always exert force normal to it. But why? According to Newton's third law, if an object hits a surface at an angle, the reaction force provided by the surface must be equal and opposite to the applied force by the object. But why does the surface always exert force normal to it?

Answer 1

Force of contact is often called the normal force. It's really the force of electrostatic charges in each body acting on the other, but if we model the field around the ground as a plate made of particles each of some charge, they make an electric field that nets only directly away (i.e. normal, hence "normal" force) to the surface. The reason they push away is these are largely electric charge forces from electrons and like charges repel.

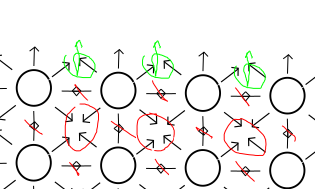
The reason the force is only normal is if we assume the particles are all the same in the body and they they are equally distributed across the surface, then some particle in a body has a field that pushes outward in every direction, but the particles all around it push back in every direction they come from, and none of the particles come from the normal direction, or else that wouldn't be the surface. I made a poor diagram, but it gets the job done. It has red lines to show how forces cancel to $0$ and the green lines show how they cancel to make a new total force in the direction of the green arrow. Notice that it only exists on the surface and points the direction that points least in the direction of every point on the plane, which is a property of the normal direction. This is why it pushes in a normal direction.

$$ % I wanted the spacing, if you are capable of making better diagrams please do, and also tell me what software you use to diagram, mine are always awful $$

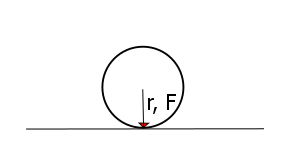
If we look at a ball, we just say that for a small of region/zoomed in enough, it is locally linear and the same as the ground, which is a plane. So a ball has a field that pushes directly away from the surface, which means it must pass through the center of the ball. And if the ball is a perfect sphere, then contact between the plane and the ball is only at one point and the plane is tangent to the ball. It is a property of tangent to be normal to the radius. So if the plane is tangent, and the force coming off of it is normal, then the force of contact (the normal force) acts along the radius of the ball. This can be seen in the following diagram.

$$ % Again, I wanted the spacing, also again, if you can make better diagrams, please do and let me know what you use to make them. $$ So when the ball comes into contact, the plane pushes on the ball completely away from the plane. Since the ground is a horizontal plane, it's normal force is completely upward so the ball must interact with the ground going completely upward. Interestingly, the plane feels the opposite, a ball comes along and pushes it down, but it's in the exact opposite direction. This is known as Newtown's Third Law, or the Weak Law of Action and Reaction. It states, "Every action has an equal and opposite reaction." It is weak as in it does not hold up in all cases, you could also research the strong law if you like, it covers some magnetic forces, which do not behave how Newton predicted.

---

However, all of this ignores friction. Friction is what happens when we realize that those electric fields are not perfect, there's little regions where the forces can't cancel perfectly to only go upward because the surface isn't perfectly flat. When we include friction we get some neat things happening, most interesting is that we can get things to spin around when they strike the ground, really useful on the basketball court if you want to show off. Push the ball off your hand with a lot of spin and you can get it to change spinning direction after it comes into contact with the court, if you do it right you can get the ball to return to you if you push it away spinning toward you, but there's a limit on how hard you push it away from you, the ball will eventually need to spin too quickly to get back to you and will end up sliding. But sliding friction is smaller than static friction and that means the ball won't get be pushed back to you as hard and might not be able to change direction.

For a better description of friction, the first thing to point out is that in the old scenario we had a perfect ball, and a perfect ball wouldn't deform at all, which you won't find in the universe, but let's say that we can approximate the atomic structure to not change position. When the surfaces come near contact, the electric fields cause the force of contact to increase, slowing the ball's fall until it pushes it back up. This would take at least some period of time. And if we go with our frictionless model, then that means the ball must have traveled sideways as it pushed into the surface because nothing stopped it from going sideways, therefore it must have continued going on its way for that period of time. So it needed to slide on the frictionless surface. This alone is enough to continue on and describe how friction works, but we can add a little better explanation of it if we let the ball and/or plane bend a little bit or deform when they come into contact. The contact between a perfect ball and plane is a point, this is how we define tangency, but if it squishes, then we'll get a small area of a likely irregular shape. This area can have defects of its own, unlike the perfect ball. So here's a picture of one surface coming into contact with another surface. The big one is the overall force felt by the ball.

Notice how we get multiple little forces pushing in non specific directions? These are what cause friction. Friction is essentially the amount of force that describes the overall effect of these little forces. For the most part, the forces on the ball will come directly off the plane in the big vector, but these small surface defects will also have an effect, the former is the contact force, or normal force, the other is called friction. From this image, we can see that both of these are contact forces, but it's easy for us to say that the ball comes into point contact with the plane, but there will be also be a force that wants to make the ball both slow down and rotate. It's more difficult to describe the normal force as a function of the two surfaces, I have to define these two surfaces and how they bend when they come into contact. At the end of the day, the second case will need to be put into a coordinate system and if you choose a pair of axes that lie on the surface normal and the surface tangent, you'll get those two forces, normal and friction.

Answer 2

The force is normal to the surface only if no friction is present. If we have some sort of sticking between the thrown object (e.g. ball) and the surface (e.g. earth), there will be a non-normal component of the force.

Just think of an ball which slides (w/o friction) on the surface of the earth. As there is no friction, there can not be a force.

Now go back to the original question. Let's consider the motion of the ball as a superposition of two motions:

• The vertical motion, which is in normal direction (say y-direction), and
• the horizontal motion (say in x-direction).

If we consider the horizontal motion to be frictionless, there can not be a force in x-direction. Hence, we are left with a normal force, which reflects the ball.

Once you include friction to the problem, you will get a superposition of two forces. From that you can derive the direction of the total force. In general it will not be along the moving direction of the ball.

Answer 3

A reaction force can surely be angled.

And it can then be split into a tangential part and a perpendicular part. The tangential part is called friction, and the perpendicular part normal force.

So, the reaction force is not at all always perpendicular - but there always is a perpendicular component (the one called normal force). This arises because, since the wall doesn't break, it must be holding back. A normal force is a "holding back" force that objects create when being pushed upon to avoid breaking.

Answer 4

From a purely Newtonian-dynamics perspective the origin of the normal force (or any force) is not explained. Instead, we infer the existence and direction of the normal force by observations of acceleration: if you see two solid objects not sinking into one another despite the influence of gravity, they must be exerting a relative force upon one another that counteracts gravity - otherwise the net force would be nonzero, so there would be a relative acceleration.

What generates that force microscopically? Well, it's a bit complicated, depending on the details of the structure of the matter in question. At the end of the day this question requires a detailed explanation of the structure of matter, since for example liquids, solids, and gasses will exert different "normal forces". But roughly, it is a combination of electrostatic repulsion and Pauli exclusion between electrons in matter.

Answer 5

The word "normal" in normal force is not referring to ordinary or commonplace. The "normal" here refers to perpendicular. This is because the normal force, usually represented with $F_n$ or just $N$, is a force that is directed perpendicular to the two surfaces in contact. It makes sense that the force is perpendicular to the surface since the normal force is what prevents solid objects from passing through each other. Surfaces can also exert contact forces in the direction parallel to the surfaces, but we would typically call those forces frictional forces (since they work to prevent the surfaces from sliding across each other) instead of calling them normal forces.

Example of this: when you stand still on the ground, you are pushing the floor down. This is a force due to gravity. Some may think that's the only force acting on you, but if that were true, you would be free falling through the floor. Why are you not falling? Because the floor is pushing you up! When you are standing still, the floor is pushing you up just as much as you are pushing down on it, which gives a net force of zero.

Normal force happens when 2 bodies with mass (usually one is a wall or floor but can be any body with mass) come to contact and have a force towards each other.

Also, friction is dependent on the normal force. Won't go into detail but friction always opposed the motion of a body of mass.

Answer 6

Let us put some symbols. We will call the velocity of the particle $\vec{v} = (v_x, v_y)$ and momentum $\vec{p} = (p_x, p_y) = (mv_x, mv_y)$. Let us supose that the collision is frictionless, to simplify, but losing some generality (not much, your satement would be false if instead of the particle we would have a rigid body with rotation and friction).

Then the question is: ¿Why $\Delta \vec p = (0, \Delta p_y) $? and $\Delta p_x =0$

We will formulate a theory for collision of a particle with a surface. What parameters descrive the system before the collision. This migh be for example the momentum $\vec p$ (or velocity $\vec v$) of the particle.

The angle of collision, that is, the angle between the normal vector to the surface $\vec n = (0,1)$ and the trajectory of the particle is guiven by the volocity on the moment of impact, so it is not an independent parameter. The angle of collision will be given by $\tan(\theta) = v_x/v_y$.

Knowing this, we will change our inertial frame, to one comoving in the $X$ axis with the particle, that is with velocity $(v_x,0)$, so in the new reference frame the momentum will be $(0,p_y)$ and the angle of collision will be $\theta =0$. The situation is completly symmetric (initial conditions and forces), thus the solution must be also symmetric (think this argument, is important in physics), so there can't be change in momentum in the $X$ axis, so $\Delta p_x = 0$.

But as Newtonian physics is independent of the inertial frame, we have that this is true in our original frame also, so all the change is in the $Y$ axis.

Change of frame and symmetry arguments are comom in physics, and simplify the arguments, nice to use them.

EDIT: Added an explanation for the angle