Which is the difference between entangled spins and classical coins?

Question

I'm trying to understand why entanglement has no classical analog. I imagine the usual well known example: Charlie prepares two spins in the entangled states. Alice and Bob measure them using their own detectors.

Why the results cannot be explained as for a classical couple of coins (head/tail)?

My understanding so far is that - if Alice and Bob measured only the z components, the two situations (quantum one and classical analog) would be really indistinguishable: when Alice measures -1 then Bob gets +1 and viceversa.

What is the difference if they measure the x components of their spins instead? They will still find they have opposite values, will they? So why couldn't this be explained again with the classical coin analog? What rules out the hypothesis that the results may have been produced in the very first moment of the entanglement creation?

Answer 1

You need to reproduce the rest of a typical experimental test of Bell inequalities and consider the component of the spin in different directions. The gist of it could be obtained as follow with your coins.

Charlie has boxes with two compartments, a red one and a blue one, with a special mechanism that locks a compartment as soon as the other one is opened. He will send one box to Bob and the other one to Alice, who will therefore only be able to look at one compartment each. Charlie randomly puts one coin in each compartment of the box for Alice: 1/2 chance of head or tail, for each compartment. Then he puts the complementary side in the box for Bob. So for example if Alice's box has head (resp. tail) in the red (resp. blue) compartment, the box for Bob will have tail (resp. head) in the red (resp. blue) compartment. He then closes the boxes and sends the to his fellow physicists without telling them what he did.

First, Alice and Bob are told to both open the red compartment. After a while, they see that Alice gets tail half of the time and Bob too but that every time Alice gets tail, Bot gets head.

Then they do the same experiment but opening the blue compartment. Same results.

If this was all classical, after guessing that Charlie has done, Alice and Bob can make a prediction at this point: if Alice opens the blue compartment and Bob opens the red compartment, on average, every other time the coins will have the same side. It is easily seen by considering all possible cases:

• Bob: head-head and Alice: tail-tail
• Bob: head-tail and Alice: tail-head
• Bob: tail-head and Alice: head-tail
• Bob: tail-tail and Alice: head-head

But then in the Quantum world, the equivalent of that prediction would be falsified.

Answer 2

Actually both quantum and classical correlations are zero when the measurements of Alice and Bob detectors involve z and x components, respectively. The above is clearly shown in the beginning of the naive view of an experimentalist and in the image below.

More surprising results arise at smaller angles. I've found the easiest explanation to understand from wikipedia:

Start with one setting exactly opposite to the other. All the pairs of particles give the same outcome (each pair is either both spin up or both spin down). Now shift Alice's setting by one degree relative to Bob's. They are now one degree off being exactly opposite to one another. A small fraction of the pairs, say f, now give different outcomes. If instead we had left Alice's setting unchanged but shifted Bob's by one degree (in the opposite direction), then again a fraction f of the pairs of particles turns out to give different outcomes. Finally consider what happens when both shifts are implemented at the same time: the two settings are now exactly two degrees away from being opposite to one another. By the mismatch argument, the chance of a mismatch at two degrees can't be more than twice the chance of a mismatch at one degree: it cannot be more than 2f.

So the measurements in my original question (along x and z axis) are not resulting in non-classical predictions.

However it has been noted that my "description applies to the singlet state, not to a general entangled state, for which the details of the correlations can be different".

Indeed, taking into account that the singlet state-vector |sing> is a superposition of two vectors |ud> and |du> which is maximally entangled, more exactly defined as

$|sing⟩ = \frac{1}{\sqrt 2} (|{↑↓}⟩-|{↓↑}⟩)$

then the x components anticorrelate. In that case the paradox would consist in the fact that

if both spins are measured along the same axis, they are found to be anti-correlated. This means that the random outcome of the measurement made on one particle seems to have been transmitted to the other, so that it can make the "right choice" when it too is measured

Anyway, the measurements only along axis can't be used to rule out a possible classical explanation (local realism) - in other words initial conditions (hidden variables distributions) exist such that measurements along same axis are anticorrelated, while along different ones have zero correlation - so, once more, Bell inequality is meaningful at smaller angles (as already discussed in the first part of this answer) between Alice's and Bob's detectors.

Answer 3

"Why the results cannot be explained as for a classical couple of coins (head/tail)?"

They can be explained as classical coins:

To start with, consider three types of classical objects, a pair of balls (white and black) a pair of gloves (right and left handed) and a pair of coins. If one of the balls, gloves and coins is given to Alice and the other, from each pair, is given to Bob, then both Alice and Bob will instantly know the color of the other’s ball and the handedness of the other’s glove (assuming they were told beforehand, that the paired balls are always white and black, and that the paired gloves are always right and left handed), regardless of how far apart they are; no “spooky action at a distance.”

But they will not know the other’s coin state (or even their own!), because that state, unlike the color of the balls and the handedness of the gloves, is not an attribute of the object itself. Rather, it is an attribute of the relative, geometric relationship, between the observer and the coin; when they look at the coin from one aspect angle, they observe it as being in the state “heads”, but when they look at it from the opposite angle, they observe the state “tails”.

So before they can even determine the state of their own coin, they have to make a decision, about which angle to observe the coin from. Making this decision and observing the result, is what is mistakenly called a “collapse of the wave-function”. But there is no wave-function, there is only a decision-making process, for determining which state, of several possible, states, the object is in, relative to the observer. Unlike the balls and gloves, objects like coins are not in ANY state, until an observer “makes it so.” Note also that even after the decision is made, the two-sided coin did not mysteriously “collapse” into a one-side coin. There is no physical collapse, there is only an interpretational collapse - a decision.

But suppose that the coins were so tiny and delicate, that the mere act of observing one, totally altered its state - it gets “flipped” every time you observe it. Now it becomes impossible to ever repeat any observation of any coin’s relative state. So it is impossible to make a second measurement of such a coin’s original state.

This brings us to the EPR paradox. Since it is now impossible to remeasure any coin, as when attempting to measure a second “component”, EPR suggested, in effect, to create pairs of coins that were “entangled”, such that they are always known, a priori, to be either parallel or anti-parallel. Hence, a measurement of one coin, should not perturb the measurement of the other. The relative orientation of the coins, relative to any observer, is assumed to be completely random. But relative to each other, the coins are either parallel or anti-parallel.

It turns out that for small particles like electrons, it is much easier to create entangled-pairs that are anti-parallel, than parallel, so we will restrict the following discussion to the anti-parallel case.

Now, whenever Alice and Bob measure each coin (one from each anti-parallel, entangled-pair) in a sequence of coins, they obtain a random sequence of “heads” or “tails”, since, regardless of what angles they decide to observe a coin from, all the coins are in different, random orientations.

But what happens if they record both their individually, decided measurement angles and the resulting, observed states of their respective coins, and subsequently get together and compare their results?

As expected, whenever they had both, by chance, decided to observe their entangled-coins from the same direction, they observe that their results are always anti-parallel; if one observed “heads”, then the other observed “tails.” And if they had both, by chance, decided to observe their entangled-coins from exactly opposite directions, they observe that their results are always parallel.

But what happens when they, by chance, happened to observe their coins at other angles? This is where things start to get interesting - and subject to mis-interpretation! Because what happens, is critically dependent upon how accurately Alice and Bob can actually decide upon the observed state of their respective coins.

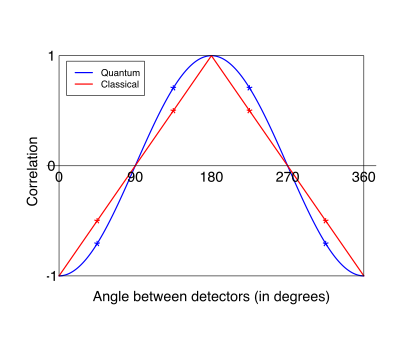
If both observers can clearly observe their coins, and make no errors in their decisions, even when the coins are perfectly “edge-on”, then you get one result (Figure 1, in this paper: http://vixra.org/pdf/1609.0129v1.pdf ) , when the observers get together and compute the correlations between their observations.

But if the coins are worn-down, dirty and bent-out of shape, and can only be observed, for a brief instant, far away, and in the dark, through a telescope (AKA with limited bandwidth, duration, and signal-to-noise ratio). Then a completely different type of correlation will appear, due to the fact that many of the observer’s decisions are erroneous; they mistakenly decided to call the coin a “head” when it was really a “tail” or vice-versa. Or they may even totally fail to detect the existence of some of the coins (fail to make any decision), as the coins whiz past them, never to be seen again.

And if they attempt to mitigate these “bit-errors”, by attempting to assess the quality of their observations, and eliminating all those measurements of the worst quality, then they will get yet another correlation - one that perfectly matches the so-called “quantum correlations”, when analogous, Bell-type experiments, are performed on subatomic particles, like photons or electrons.

So, should quantum correlations be interpreted as a “spooky action at a distance”, or just a misunderstood classical phenomenon? Given the (little known) fact that the limiting case of the Heisenberg Uncertainty Principle can be shown to (just an amazing coincidence !!!???) correspond to a single-bit-of-information being present, which thereby guarantees that every set of multiple observations must be “strangely correlated”, “spooky action at a distance” seems to be an implausible interpretation, at best.

It is worth pointing-out, that a classical coin, as described above, is simultaneously BOTH a heads and a tails - that is what a superposition of two states looks like - a coin - until an observer makes a decision, and "calls it" - either a heads or a tails. Perhaps it should be called Schrödinger's coin.