Bounds of Integration (with respect to something that is not time)

Question

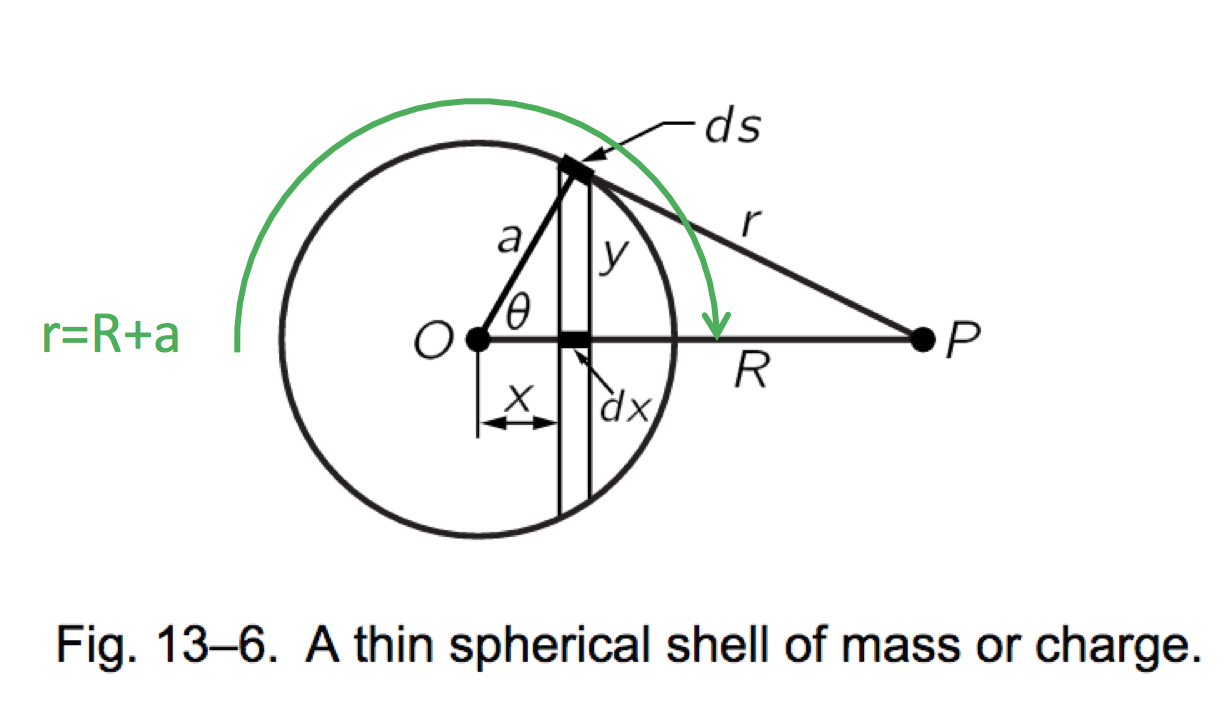
I have been reading Richard Feynman's lectures and came across an interesting proof regarding the Earth's gravitational force. At one point in the proof, Feynman uses the following the integral:

$\int_{R+a}^{R-a} dr$ (13.18 on http://www.feynmanlectures.caltech.edu/I_13.html)

In this integral, r is the distance between a point in space and the surface of the Earth, R is the distance between that point and the center of the Earth, and a is the radius of the Earth. I interpret this integral as summing up all of the dr's going around the Earth. The proof itself makes sense to me, I am just confused about the bounds of integration.

As $\int_{R+a}^{R-a} dr$, I interpret the integral as summing up the dr's starting on the right side of the Earth and going to the left side. However, in this sense, $\int_{R-a}^{R+a} dr$ should be the sum of all the dr's starting from the left side and going to the right side. Conceptually, I feel as if these should be the same, but mathematically $\int_{R+a}^{R-a} dr = -\int_{R-a}^{R+a} dr$. My question is, how did Feynman choose the ordering of his bounds of integration? It does not appear arbitrary, but I am not sure how the decision was made. Thank you!

Answer 1

In this integral, r is the distance between a point in space and the surface of the Earth, ...

Almost. Actually, based on the diagram in the derivation, $r$ is the distance from the point in space to the ring of mass that is part of the spherical shell. It $is$ a distance like you describe, but it is the distance to a very specific geometrical piece of mass.

I interpret this integral as summing up all of the dr's going around the Earth.

Maybe, depending on what you mean by "going around the earth." I would describe the integral as summing all the rings of mass, starting at the distance $R+a$ (on the far left end of the shell) and accumulating ring by ring all the way to $R-a$ (on the far right end of the shell). The integral around the earth which creates each ring in the shell was done implicitly and results in the $2\pi$ factor.

Answer 2

Reading through the proof, it seems like he actually starts by working in $x$, then proves the relationship between $x$ and $r$ (which includes the negative sign): $2r~dr = -2R~dx$.

Keeping the direction of the integration the same, you can see that the natural order for $r$ is from larger ($r=R+a$) to smaller ($r=R-a$):

The sign change (going from x positive to r negative) is absorbed by removing the negative sign that was in front of the original expression for W.

---

^{Image source: http://www.feynmanlectures.caltech.edu/I_13.html modified to include direction of integration}

Answer 3

The problem has nothing to do with change of variable. It is just about orientation, where you are choosing to put your zero potential energy. He is putting it at $r=\infty$. This is just a convention, no math. You can put the zero anywhere you like. The integral is then from $r=\infty$ to $0$. So, the integral is really $\int_{\infty}^{0}dW$. There is no mass everywhere in that interval. The mass only counts in the interval from $R+a$ to $R−a$. That is why you end up computing $\int_{R+a}^{R-a}dW$. That's all. Put your zero potential energy at $r=0$ and the order reverses. The sign of the resulting potential energy accounts for the different choice in zero level.

See Why choose a convention where gravitational energy is negative?