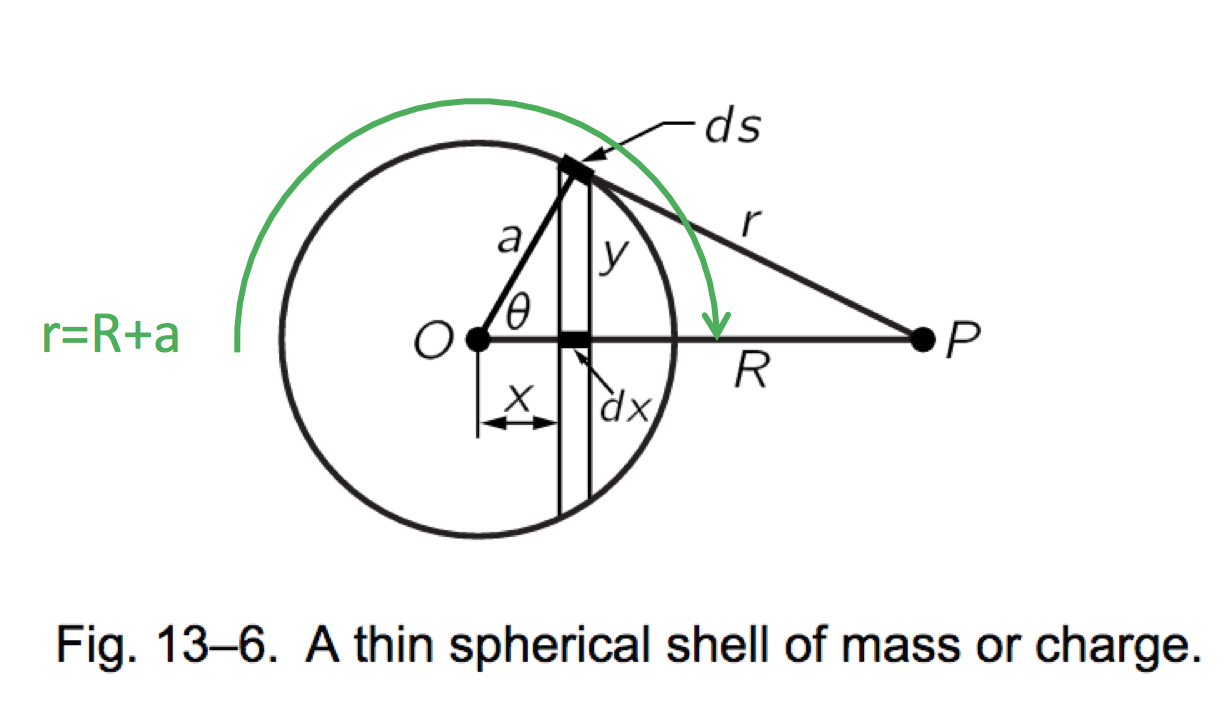
Reading through the proof, it seems like he actually starts by working in $x$, then proves the relationship between $x$ and $r$ (which includes the negative sign): $2r~dr = -2R~dx$. Rather than making the negative sign explicit, he flips the order of the integration bounds (which has the same effect).

Reading through the proof, it seems like he actually starts by working in $x$, then proves the relationship between $x$ and $r$ (which includes the negative sign): $2r~dr = -2R~dx$.

Keeping the direction of the integration the same, you can see that the natural order for $r$ is from larger ($r=R+a$) to smaller ($r=R-a$):

The sign change (going from x positive to r negative) is absorbed by removing the negative sign that was in front of the original expression for W.

^{Image source: http://www.feynmanlectures.caltech.edu/I_13.html modified to include direction of integration}