When the Young double slit experiment is performed with 2 separate slits..and interference is observed does Diffraction not take place in the 2 separate slits and thus affect the interference of 2 waves from the slits?
-
$\begingroup$ Possible Duplicate of : physics.stackexchange.com/questions/219594/… (Couldn't flag the question) $\endgroup$– SumantCommented Apr 16, 2017 at 12:53
-
$\begingroup$ whenever an EM wave, any wave, encounters any finite obstacle, say a screen with a slit, then there is always diffraction. $\endgroup$– hyportnexCommented Apr 16, 2017 at 12:55
-
$\begingroup$ So how does that diffraction affect the double slit interference pattern? $\endgroup$– user153175Commented Apr 16, 2017 at 13:07
-
$\begingroup$ There would be no interference on the screen unless there is diffraction at the slits ... right? $\endgroup$– garypCommented Apr 16, 2017 at 13:20
-
$\begingroup$ Perhaps this ishelpful to draw the full picture: What is the explanation for the phenomenon that single photons produce fringes even behind single edges?) and If I put one of the slits from the Young's double slit experiment apparatus into a different medium what happens to the fringe width? $\endgroup$– HolgerFiedlerCommented Apr 16, 2017 at 15:13
2 Answers
Diffraction
Wikipedia states that diffraction
is defined as the bending of light around the corners of an obstacle or aperture into the region of geometrical shadow of the obstacle.
Due to the first meaning coming from Francesco Maria Grimaldi diffraction refers also to the phenomenon of fringes behind obstacles:
light break up; that is, that parts of the compound [i.e., the beam of light], separated by division
Deflection
Experiments with point-like sources and the observation of fringes (intensity distributions) behind obstacles are done with electrons too (Bi-prism experiment by Möllenstedt and Jönnson, see Zeitschrift für Naturforschung 10 a S. 256 a). In contrast to the definition of diffraction as bending radiation into the region of geometrical shadow the results of this experiments have shown that the electrons get deflected only away from the geometrical shadow:
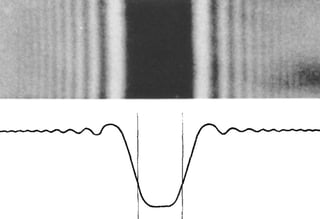
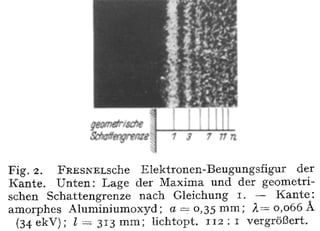
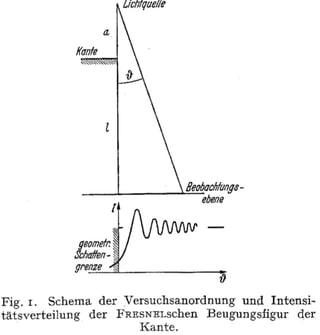
Some years before this biprizm experiment Hans Boersch published Fresnel'sche Elektronenbeugung (an article from 1940 behind a paywall :-0). It was shown that electrons behind an edge get deflected (Beugung = deflection):
Interference
To your question
does diffraction not take place in the 2 separate slits and thus affect the interference of 2 waves from the slits?
and according to the above mentioned experiments is given the following answer:
- Not only behind a separate slit diffraction occurs but deflection occurs behind every (sharp or thin) edge.
- The intensity distribution (fringes) starts for EM radiation inside the geometrical shadow and for electrons at potential-free edges outside the geometrical shadow. So for photons and for electrons the diffraction happens in different ways.
- Yes you are right with the implication in your question in the sense that somehow the intensity distribution of the four edges of a double slit is the sum of the distribution behind every of the edges. You could call it interference or simply intensity distribution.
Which one is primary, diffraction or interference, is an ancient and rather sterile question because one does not exist without the other. Yes, one can imagine non-physical but purely mathematical notions of two idealized plane or spherical waves that interfere with each other without anything diffracting but there are no idealized plane or spherical waves. Those are idealized. Real waves that are generated from real antennas do diffract at their sources, in fact that diffraction combined with interference will produce the radiation pattern, never one without the other. (One can even say that an ideal hemi-spherical wave source is really just the ideal diffraction of a pure plane wave source from a point - see Huygens' principle, so even there is diffraction). When an idealized plane wave hits one or two or many slits, the waves passing through the slits will suffer diffraction (see comment above by @garyp ) and the sum of those diffracted waves interfere with each other the result of which when collimated produces the interference pattern on the screen. This is all formulated mathematically in the Huygens-Fresnel principle and in the corresponding Kirchhoff integral, see https://en.wikipedia.org/wiki/Huygens%E2%80%93Fresnel_principle
-
$\begingroup$ Ok.. So is it like the interfering waves in a slit again interfere to produce a wave pattern on the screen? As, interference should take place individually in those slits as in the single slit expt? $\endgroup$ Commented Apr 16, 2017 at 14:28
-
$\begingroup$ It's hard to understand what you're asking..., but I think the answer is yes. In the real world, the double slit experiment requires you to consider both single-slit interference effects and double-slit effects at the same time. Most treatments oversimplify the situation by assuming the slits are like "point sources", but that's only an approximation. $\endgroup$ Commented Apr 16, 2017 at 15:15