We don't yet, but we will soon.
When this question was asked, in 2012, the process of redefinition of the SI unit system had only just begun: it was officially kicked off by the CGPM resolution in 2011 and the current timeline (pdf) is on track for an official re-definition in 2018. The Wikipedia page on the proposed redefinitions has a good introduction, and the question What are the proposed realizations in the New SI for the kilogram, ampere, kelvin and mole? has much of the detail on what the redefinitions will actually mean in practice.
In particular, the kilogram will be defined as the unit of mass such that Planck's constant has an exact value of $h=6.626\,069\,\mathrm{X} \times 10^{-34}\:\mathrm{J\:m^2s^{-2}}$ (where the final digits, $\mathrm{X}$, will be fixed at the time of the redefinition in 2018). This leaves it open to experimentalists to implement their own mass standards (and in fact it frees other quantities from a strict dependence on the mass standard itself, so you could in principle make a standard for torque or pressure, say, that didn't directly depend on a mass standard), but the main proposed implementation is the watt balance, which looks sort of like this,
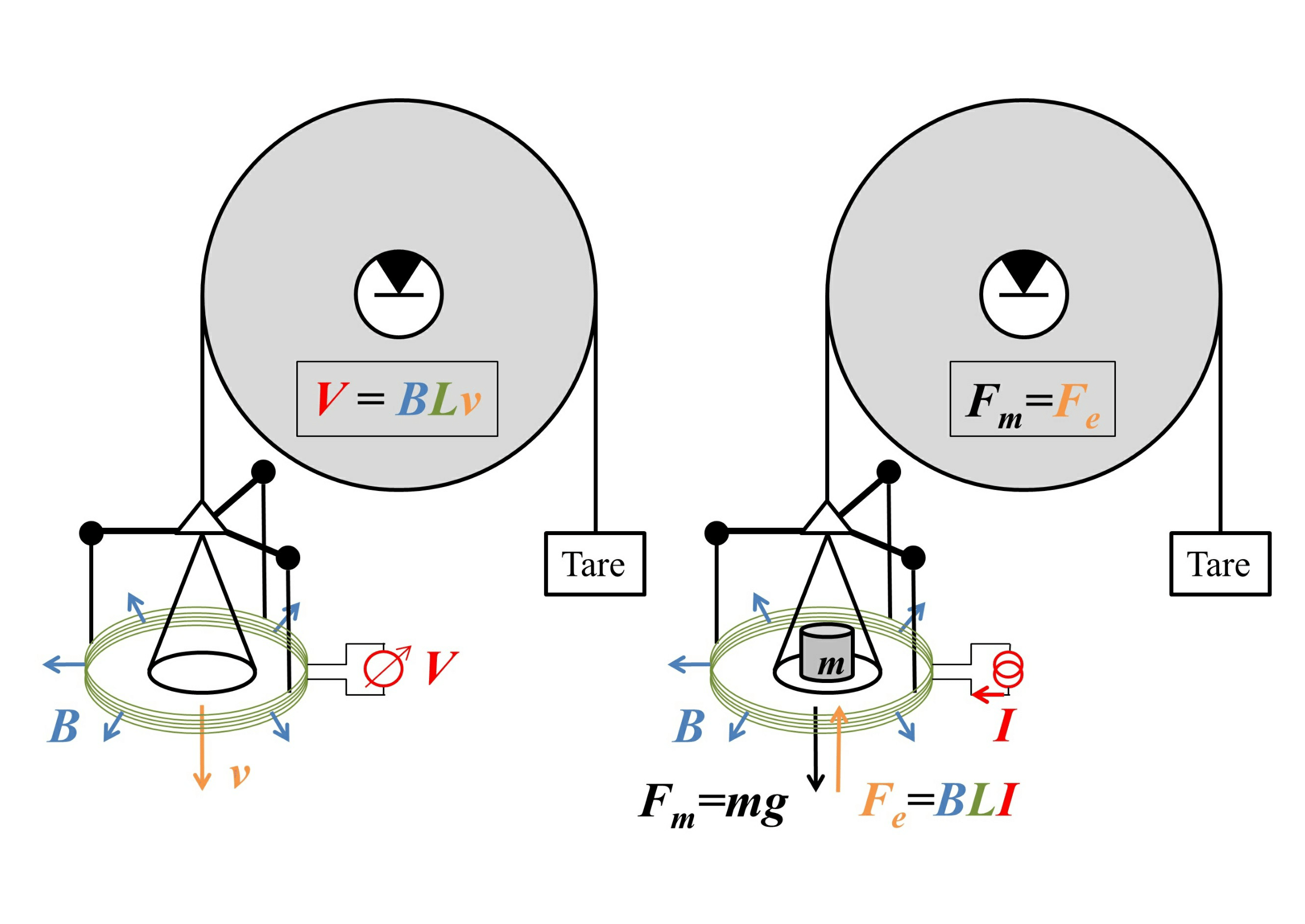
Image source: arXiv:1412.1699, which also describes how to build one of these out of LEGO. Fun video on how it works.
and which is discussed in more depth in the implementations question and references therein.
There was an alternative approach, the Avogadro Project, based on making a big shiny sphere made of a precisely known number of silicon atoms, and it would have gone through fixed values of the Avogadro constant and the mass of the 28Si atom. However, that project was set aside for a redefinition of the mole, and the watt balance is the main proposed mise en pratique for the kilogram standard.
As for the question itself, the issue of why it took so long is... mostly a bit of a non-issue for me. Metrologists were busy doing other things, and having an artifact-based definition is not, by itself, that problematic, at least conceptually. Metrology is a very pragmatic science, and the goal is to have clean, reproducible, accurate measurements for as many quantities as are needed in modern science, and conceptual cleanliness takes a bit of a back seat there. There are, really, two valid driving reasons for a change in the standards to a constant-based definition.
One is when the standard drifts, as happened with the solar second, which is a wrecking ball for reproducibility of experiments at accuracies past the drift rate.
The other is when the constant-based definitions are significantly more accurate and stable than the artifact-based metrological chain, as happened with the metre in 1960, when the limiting uncertainty in the distance to the moon, say, was the uncertainty in the metre standard.
Both of these factors are relevant for the kilogram today, but they've only become relevant recently.
The current SI kilogram does indeed (probably!) drift, as explained in the wikipedia article on the stability of the IPK mass. This means that a well-calibrated measurement of a 1kg mass today is not reproducibly comparable to the exact same measurement performed fifty years ago, or at least not past the ${\sim}10\:\mathrm{\mu g}$ scale. Moreover, this impacts all measurements that depend metrologically on the mass standard, i.e. all measurements whose bare SI expression involves the kilogram. That's... not great.
However, this is a problem but it's not a huge problem. The kilogram has drifted in the scale of roughly ${\sim}10\:\mathrm{\mu g}$ over a century, which means that it has a stability on the order of $10^{-10}\textrm{ yr}^{-1}$, which is actually not that bad. It's probably time to change it, but there aren't that many kg-dependent measurements down to ten significant figures that are much older than one or two decades.
There are now more accurate and stable ways of determining mass, but these are very recent. It turns out to be hard to design measurements of Planck's constant to that degree of accuracy and stability, and it is only with 21st-century technology that we've managed to close the gap.
Moreover, old-school balances turn out to be surprisingly accurate. As dmckee mentioned in a comment, even in the 19th century it was possible to build analytic balances that measured ${\sim}100\:\mathrm{\mu g}$ differences between ${\sim}2 \:\mathrm{ kg}$ masses, i.e. down to about seven significant figures. Metrology spent much of the twentieth century improving this technology, and porting it to other mechanical quantities. IPK-based metrology is a formidable edifice to replace (and, in fact, much of it may well remain in use, with only the primary standard changing with the redefinition, but secondary and tertiary layers unaffected).
Now, these reasons sort of tip the balance (har, har) towards the change, but it also explains why it isn't a huge priority. Metrology labs invest in research on things like watt balances because they want more accurate and stable standards, not because it's "fairly important", conceptually, to have a constants-based definition. An investment on the scale of the LHC (or even a minor fraction of that) has not yet been warranted because metrology was doing fine as is. Changes are now being made, but they're slow because metrology is a slow science, requiring agreement between multiple different approaches and labs before anything is set in stone.

