Suppose I have an intense laser light beam and an electron beam. What happens when they meet at a point in space? Is it possible for the electron to get deflected by the photon? Will they affect each other?
$\begingroup$
$\endgroup$
1
-
$\begingroup$ see the answer here quora.com/… $\endgroup$– anna vCommented Mar 22, 2017 at 17:10
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
0
This is the operating principle behind the Compton polarimeters which are used to measure the polarization of the electron beams into two of the four experiment halls at Jefferson Lab in the USA. The electrons, which have energy 1--10 GeV, pass through a laser cavity that's filled with a kilowatt or so of green laser light, nearly parallel to the electron beam. In the rest frame of the electron, however, it's the photon that has high energy, and scattering is allowed. The result (a nice homework problem) is that the electron gives up a small fraction its energy and momentum to turn that boring green photon into a kind of amazing 0.1--1 GeV gamma ray.
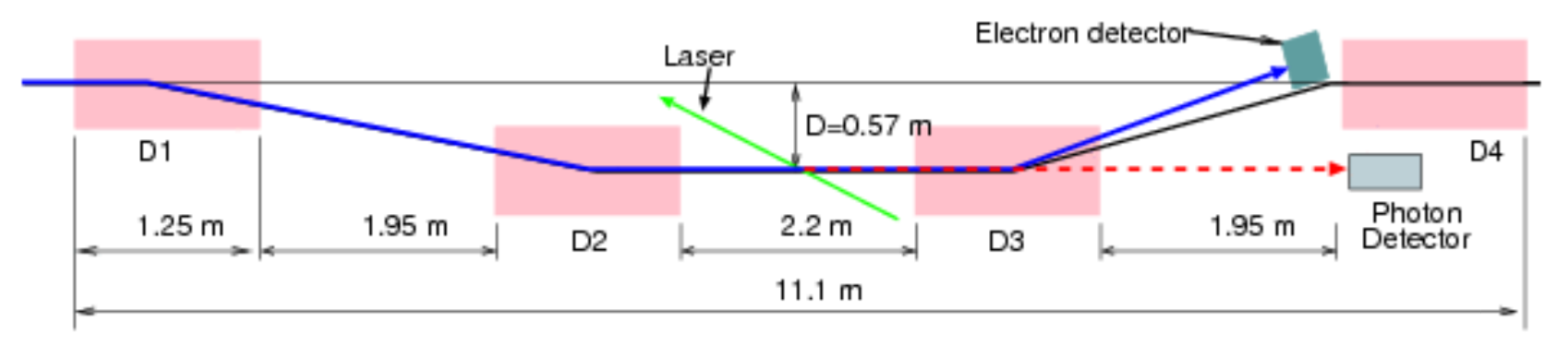
Here's a cartoon of the Hall C Compton polarimeter, which I found in some slides by D. Gaskell:
The beam (in blue) comes in from the left. Because of relativistic beaming, the slowed-down electron and the upscattered photon both have their momentum quite parallel to the initial electron beam. In the JLab polarimeters, the laser interaction region is offset from the "straight-through" beam path by a chicane, with four bending dipole magnets (in pink). The hard photons aren't affected by the bending magnets, while the electrons which interact with the laser are more bent than their companions who passed through the laser cavity without scattering. So the third bending magnet separates the beam into three components: the unscattered electrons (which are straightened out by D4 and continue to an experiment in the hall), the unbent photon beam, and a smear of scattered electrons. Thanks to the Compton edge, the scattered beam is well-separated from the primary beam. There's lots of neat experimental phase space here.
(Why is it a "polarimeter"? Scattering between an electron and a photon requires a spin flip. If the laser light in the cavity is polarized, then the cross-section for scattering depends on the electron polarization.)
