LCAO - Constructing a molecular wavefunction

Question

I am a theoretical physics student and have been studying the LCAO (Linear Combination of Atomic Orbitals) technique from the perspective of a physicist. I would like to get some explicit clarification on the fine details of the LCAO technique, as I have picked up some confusion over some of the terms used, and, to be honest, exactly what the final wavefunction expression should look like. I will use a (very abstract) example to highlight some of my confusion. Please note that my concerns and interests here are entirely in the quantum mechanics and the mathematics of constructing a molecular wavefunction (not a discussion on the shapes of molecules).

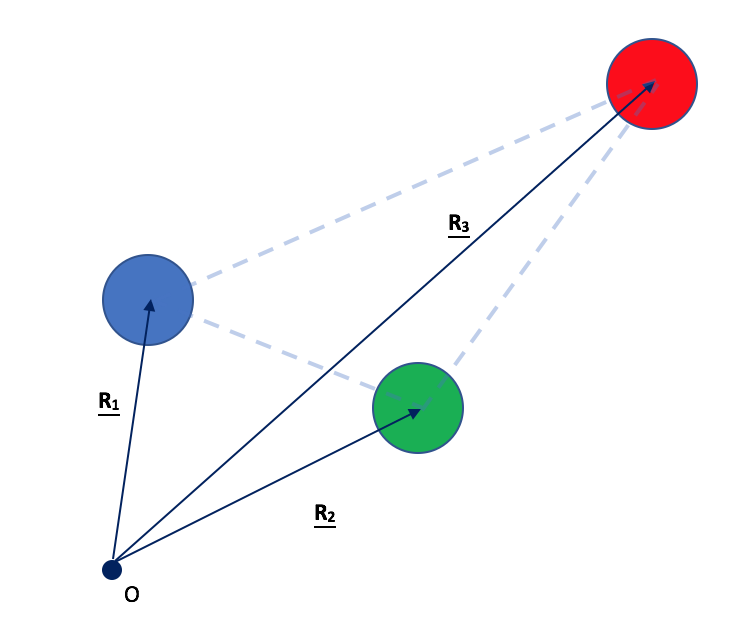
Let's consider the following strange, highly asymmetric tri-atomic planar molecule that I came up with, as an example, which allows me to treat a more general case):

For the moment, imagine there are no electrons in the system; just the three (different) nuclei which I have given different colours (blue, green and red for sites 1, 2 and 3 respcetively) which have charges $Z_1$, $Z_2$ and $Z_3$ respectively.

Now, it is my understanding that an 'atomic orbital' (a hydrogenic eigenstate) centered on, say, nucleus 1 would look like:

$$\psi^{1}_{nlm}(\vec{r}) = \psi_{nlm}(\vec{r}-\vec{R_1}) $$

Where $\psi_{nlm}$ denotes a hydrogen wavefunction with quantum numbers $n, l, m$ and using nuclear charge $Z_1$. This is just a hydrogen wavefunction with shifted origin. Likewise, we could also write down atomic orbitals centered on nuclei 2 and 3:

$$\psi^{2}_{nlm}(\vec{r}) = \psi_{nlm}(\vec{r}-\vec{R_2}) $$ $$\psi^{3}_{nlm}(\vec{r}) = \psi_{nlm}(\vec{r}-\vec{R_3}) $$

Where we again note that $Z_2$ and $Z_3$ replace the standard hydrogenic $Z=1$ charge in the wavefunction expressions.

Now that we have these atomic oribtals written down, it is my understanding that we can start constructing 'molecular orbitals' from a linear combination of these atomic orbitals. For example, lets take a 1s orbital on each nucleus. Neglecting normalisation for now, one possible 'molecular orbital' could be (I'll use $\phi$ to denote molecular orbitals), in my understanding:

$$\phi^{a}(\vec{r}) = \psi^{1}_{100}(\vec{r}) + \psi^{2}_{100}(\vec{r}) + \psi^{3}_{100}(\vec{r})$$

Where I have used the notation for $\psi^{1}$, $\psi^{2}$ and $\psi^{3}$ defined above. Another possible combination could be (lets call this one $\phi^b$):

$$\phi^{b}(\vec{r}) = \psi^{1}_{100}(\vec{r}) + \psi^{2}_{100}(\vec{r}) - \psi^{3}_{100}(\vec{r})$$

A more complicated molecular orbital could look like:

$$\phi^{c}(\vec{r}) = \psi^{1}_{320}(\vec{r}) + \psi^{2}_{421}(\vec{r}) + \psi^{3}_{211}(\vec{r})$$

And so on. There are many possible combinations we could come up with. Each of the above is a 'molecular orbital' and can be occupied by an electron when we decide to put some into the system (see below). I would like to confirm that the examples I have given above are indeed 'molecular orbitals'. Is this correct?

So far, I've not put any electrons into the system. Lets say, for the sake of argument, I decide to put two electrons into the system (with position vectors $\vec{r_1}$ and $\vec{r_2}$). Lets say that one occupies the $\phi^{a}$ defined state above and the other occupies $\phi^{c}$. We could choose a symmetric combination (again neglecting normalisation):

$$\chi^{symm}(\vec{r_1}, \vec{r_2}) = \phi^{a}(\vec{r_1}) \phi^{c}(\vec{r_2}) + \phi^{c}(\vec{r_1}) \phi^{a}(\vec{r_2})$$

Or the corresponding antisymmetric one. There are, of course, many many possible different $\chi$'s depending on which molecular orbitals we choose to occupy.

It is my understanding that as electrons are added to the system, they'll start filling the molecular orbitals from the lowest energy one up. So, in principle, you could find the energy of every single molecular orbital, rank them from lowest to highest, and fill them from the bottom up in order to get the ground state of the molecule. Is this correct?

I appreciate LCAO is highly approximative, especially for more complicated molecules. It's the basics of the method I want to get nailed.

Answer 1

I would like to confirm that the examples I have given above are indeed 'molecular orbitals'. Is this correct?

Yes, those are molecular orbitals, but they're not great at their job. For starters, you should really allow the orbitals from different centres to contribute with different weights: $$\phi^{a}(\vec{r}) = c_1\,\psi^{1}_{100}(\vec{r}) + c_2\, \psi^{2}_{100}(\vec{r}) + c_3\, \psi^{3}_{100}(\vec{r}).$$ Similarly, there's nothing stopping you from having multiple single-center orbitals contributing to the same molecular orbital, such as e.g. $$\phi^{a}(\vec{r}) = \left(c_1\,\psi^{1}_{100}(\vec{r}) + b_1\,\psi^{1}_{200}(\vec r)\right) + c\, \psi^{2}_{100}(\vec{r}) + c_3\, \psi^{3}_{100}(\vec{r}),$$ or in its more general form $$ \phi^{a}(\vec{r}) = \sum_{k,n,l,m}c^k_{nlm}\,\psi^{k}_{nlm}(\vec{r}). $$ In general, moreover, you really want to use this opportunity to add in spin into the system now, rather than later, so you form what's known as spin orbitals: you add a label $i$ for spin into your coordinates $\mathbf x=(\vec r,i)$, and you choose a basis of spin states $\sigma=\{\alpha, \beta\}$, getting you to the form $$ \phi^{a}(\mathbf{x}) = \sum_{k,n,l,m,\sigma}c^k_{nlm\sigma}\,\psi^{k}_{nlm}(\vec{r})\sigma(i). $$

After this, then yeah, you've got most of how it works:

It is my understanding that as electrons are added to the system, they'll start filling the molecular orbitals from the lowest energy one up. So, in principle, you could find the energy of every single molecular orbital, rank them from lowest to highest, and fill them from the bottom up in order to get the ground state of the molecule. Is this correct?

That's essentially correct. The problem, though, is how do you find out what energies the orbitals have, and more importantly how do you shape the orbitals by finding the correct $c^k_{nlmi}$'s to describe the system? Ideally, you would want your molecular orbitals to obey some form of Schrödinger equation, a la $$ \hat H |\phi^a\rangle = E_a|\phi^a\rangle, $$ but the problem is that $\hat H$ includes the electron-electron repulsion, and the average of that repulsion depends on the shape of the clouds occupied by the electrons and those depend on the $\phi^a$, so you've got a problem there.

The usual resolution is to resolve this via the Hartree-Fock method, essentially by putting in reasonable guesses, diagonalizing (numerically) for $\hat H$, then updating $\hat H$ with the new eigenorbitals, diagonalizing again, putting in the new orbitals, and so on and so forth until you hopefully converge onto a self-consistent set of orbitals.

After that, then yes, you get a bunch of orbitals associated with energies, and you fill them up from the bottom up until you run out of electrons.

That said, though, at this stage you should also be aware that whenever we say "fill in the electrons on these orbitals", what we're really saying is "the global $N$-electron state is given by a Slater determinant composed of the indicated orbitals", and nothing less. I should also mention that orbitals are a tricky concept in a multi-electron setting, but we can usually decide on a set that makes for good tools for understanding any given molecule.

There's plenty of additional subtleties to be had, but I'll stop here.