You're slightly conflating MO formation with hybridisation.
MO's form as an interaction between two Atomic Orbitals (AOs) and mathematically can be seen as the linear combination of two AOs.
MOs can be formed when some conditions are met:
1. Symmetry and sufficient overlap:
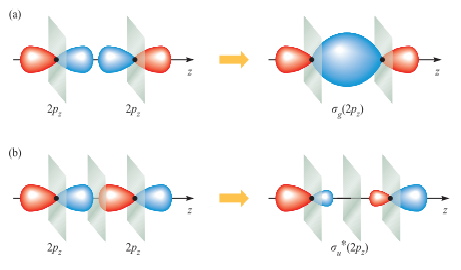
(a) formation of a $\sigma_{pp}$ bond.
(b) formation of a $\sigma^*_{pp}$ anti-bond.

(c) formation of a $\pi_{pp}$ bond.
(d) formation of a $\pi^*_{pp}$ anti-bond.

Lack of symmetry in (a) and (b) (above) prevents bonding (no overlap). (c) forms a $\sigma_{sp}$ bond.
2. Orbital lobes interacting must have same wave function sign (gerade/ungerade):
The cases (b) and (d) above lead to anti-bonds because the orbital lobes are of opposite sign (as indicated by colour).
3. AOs must be of comparable energy levels.
4. MOs can contain a maximum of 2 electrons (with differing spin quantum number, acc. Pauli).
Hybridisation of AOs:
Hybridisation features heavily in Valence Shell Electron Pair Repulsion theory. Hybridisation minimises the potential energy of many molecules by minimising the electrostatic repulsion between the MOs.
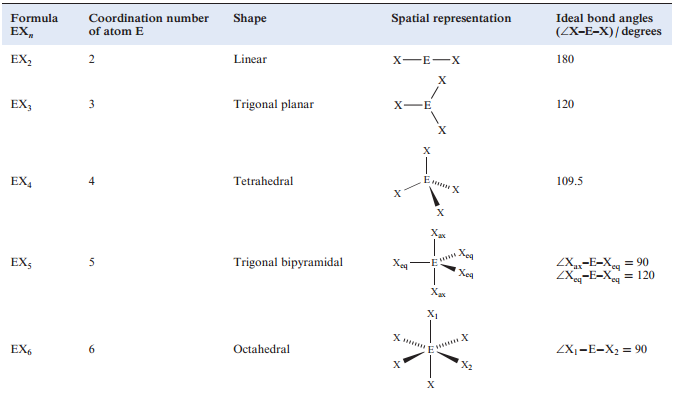
It explains the bond angles for compounds of the $EX_n$ ($X$ is a halogen or hydrogen, $E$ is some element) group of binary compounds. For example, methane's ($CH_4$) tetrahedral structure is explained by it, because that bond angle minimises electrostatic repulsion between the MOs (four $\sigma$ MOs from linear combination of (hydrogen) $1s$ AOs and hybridised $sp^3$ carbon AOs).
The hybridisation process can be schematised as electron configurations as follows:
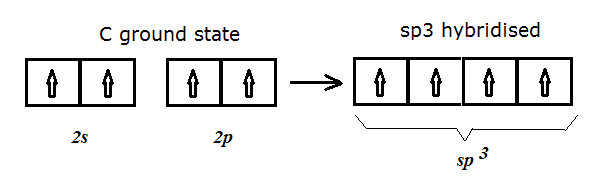
For carbon the $sp^3$ hybridised AOs look like below. For clarity the AOs have been presented separately, with white dots representing the C-nucleus:
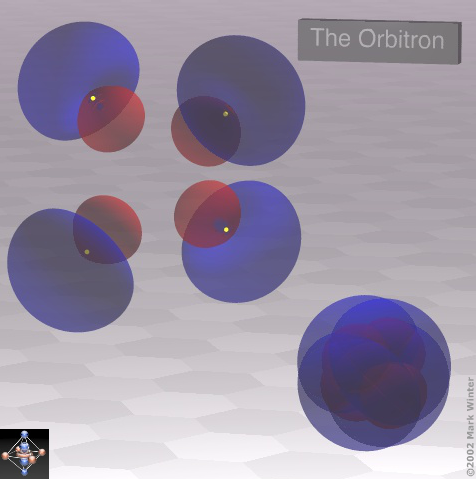
([Image source.])6
The molecular shapes and bond angles for $EX_n$ compounds, due to hybridisation of MOs:
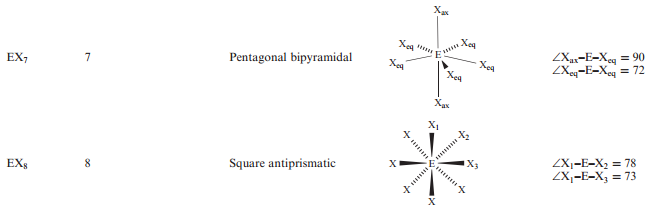
Edit: in response to OP's question in the comments.
$s$ and $p_{x,y,z}$ orbitals can combine to bonding $\sigma$ bonds (MOs), as long as one of the $p$ lobes lies on the inter nuclear axis (the axis connecting the nuclei of the bonding atoms) and provided the energy levels of the $s$ and $p$ are not too far apart:
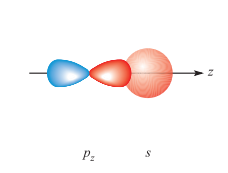
What we call that $p$ orbital ($x$,$y$ or $z$) is a matter of convention, as we can choose the coordinate system to our convenience. Conventionally, the one interacting with an $s$ is set to $z$.
Now have a look at this, bonding and anti-bonding between two $1s$ AOs:
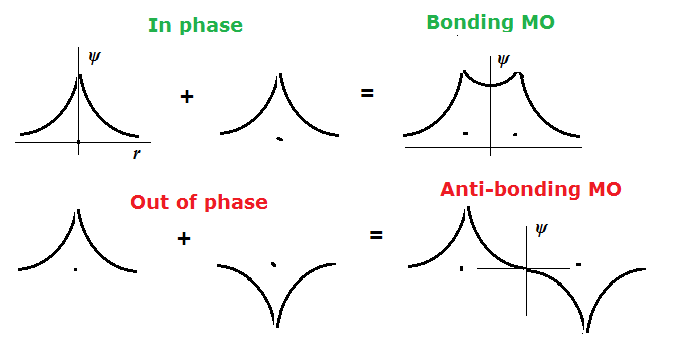
Remember that both wave functions $+ ψ$ and $– ψ$ satisfy the Schrödinger equation. In the top part of the diagram the wave functions have the same sign (either +,+ or -,-) and are said to be ‘in phase’. In the bottom part the wave functions have the opposite sign (either +,- or -,+) and are said to be ‘out of phase’.
Like actual waves, the wave functions can now show reinforcing interference when they are in phase (top) or negative interference when they out of phase (bottom).
In the top situation a bonding molecular orbital has formed. See how in the top part the value for $ψ^2$ between the nuclei (represented as dots) is positive. This means there is considerable probability of finding the electron(s) in that region and this is where they reduce the electrostatic repulsion between the (both positively charged) nuclei. A $σ_{ss}$ ($σ$ for short) has formed and a $H_{2}$ is born.
But in the bottom part for $ψ^2$ between the nuclei is zero and with no electron presence in that region there is nothing to prevent the electrostatic repulsion between the nuclei from ripping the ensemble apart. This orbital is called an anti-bonding molecular orbital, noted as $σ^*$.
The same principle holds for other types of bonds: the interacting lobes must be in phase (of the same sign - i.e. colour in the figures higher up).
Note that $\pi$ bonds don't offer much inter-nuclear electron density and for that reason never occur on their own but always as part of a double bond (one $\sigma$ plus one $\pi$) or triple bond (one $\sigma$ plus two $\pi$).









