It is known that protons are held together inside the nucleus by nuclear forces which are short range forces. Now my question is how are the protons brought together when they are far apart despite their repulsion?
$\begingroup$
$\endgroup$
1
-
$\begingroup$ Two protons ('diproton') isn't actually a stable arrangement. But fusion of other proton containing nuclei can be achieved (e.g.) in the interior of stars, where the core temperature is near a billion Kelvin. In these conditions, the nuclei have such high kinetic energy that the work needed to overcome the electrostatic repulsion can be done. Once close enough together the short-range nuclear forces do the 'binding' of the nuclei. $\endgroup$– GertCommented Oct 27, 2016 at 17:24
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
3
Protons with high enough kinetic energy can approach each other so much, in spite of the Coulomb repulsion, that they can tunnel through the remaining energy barrier and come into the reach of the strong nuclear force. The resulting diproton nucleus is unstable and one proton converts into a neutron so that a deuterium nucleus (deuteron) results. This deuterium continues in fusion reactions that fuel our sun.
answered Oct 27, 2016 at 17:29
-
1$\begingroup$ From where do they gain such high kinetic energy?? $\endgroup$ Commented Oct 27, 2016 at 17:54
-
1$\begingroup$ +1 for mentioning tunneling! Extremely important phenomenon. $\endgroup$– Bill NCommented Oct 27, 2016 at 18:02
-
$\begingroup$ @SaujatyaKole From the original Big Bang source, as the expansion cooled the universe there were regions where during the inflation period the seeds of galaxies , high density regions, had much higher kinetic energies than what became finally the void between clusters of galaxies and galaxies. Also gravitational attraction and accumulation of matter raises kinetic energies , when a cloud of matter condenses into a star like the sun, for example, changing potential energy (from original BB ) to kinetic. $\endgroup$– anna vCommented Oct 29, 2016 at 4:00
$\begingroup$
$\endgroup$
As @freecharly mentioned and @Bill N remarked, it is about tunneling and kinetic energies.
A clear example emerge in the so-called stellar nucleosynthesis. Here, we have two main protagonists: the gravitational force, which tends to contract the star, and the nuclear fusion reaction, which compensate the previous one and keep the star stable. The gravitational attraction also causes the temperature, say the kinetic energy of the protons (or the other nuclei), to raise, and this is what makes the fusion process start.
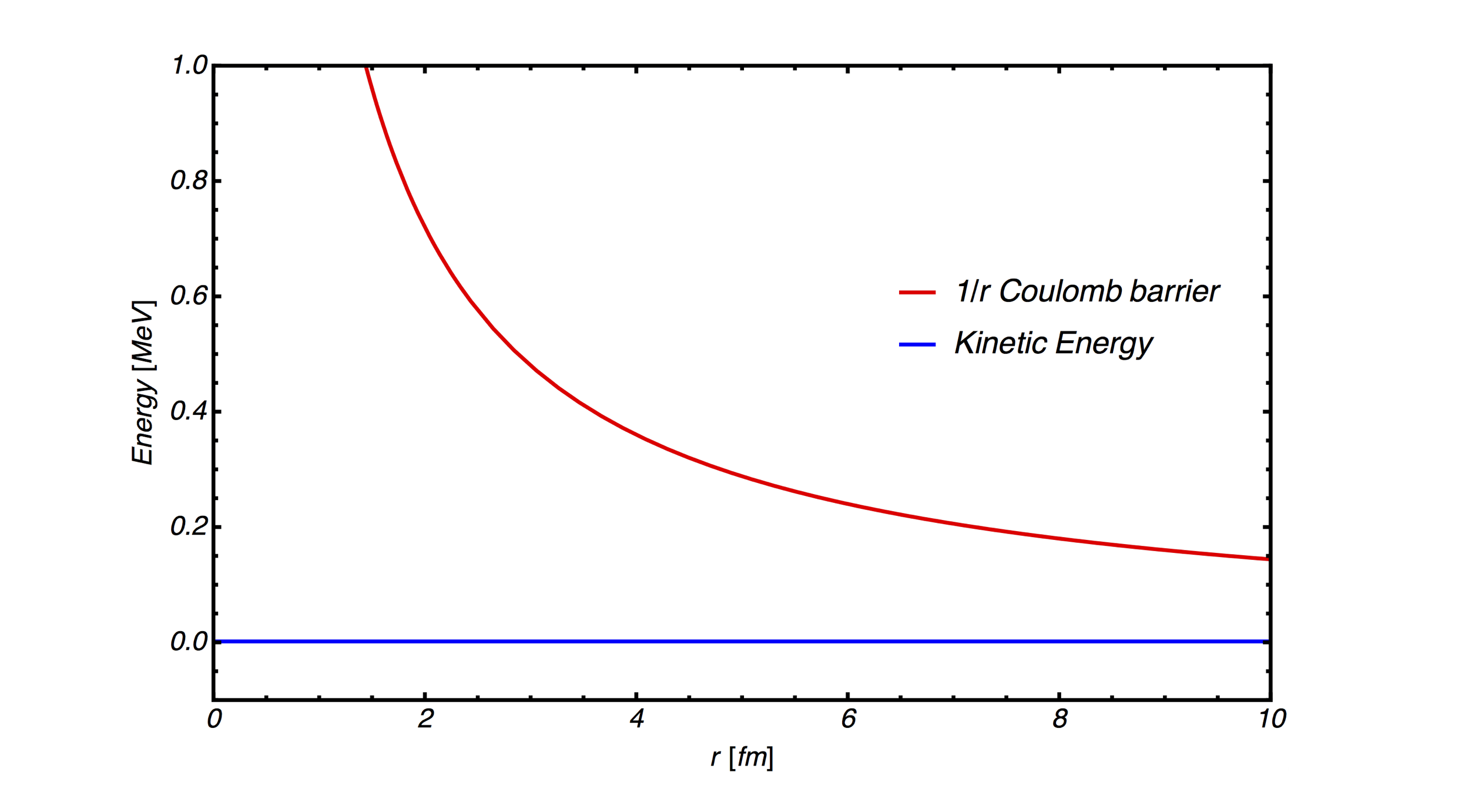
Take the Sun. Roughly speaking it has a core temperature of about $1.6\times 10^7$ K. Then, we can get the kinetic energy by
$$ E=k_B T_{Sun}\sim1.6\times10^{-3}\text{ MeV}. $$
This is quite a small energy and leads to a very long tunneling (hence very small cross sections). To get a graphical idea of the comparison between the coulomb barrier and the average kinetic energy take a look at the image below (Coulomb repulsion $=\frac{Z_1Z_2e^2}{r}\sim\frac{1.44\text{ MeVfm}}{r}$).
At some point the temperature will be high enough to make this tunneling possible. In particular, in the sun we have the proton-proton chain reaction (I), which starts with the synthesis of deuteron: $$ p+p\to \text{d}+e^++\nu_e, $$ followed by $$ \text{d}+p\to {^3\text{He}}+\gamma\;\;\;\text{ and }\;\;\;{^3\text{He}}+{^3\text{He}}\to {^4\text{He}}+2p. $$ One can compute that synthesis of helium starts, thanks to this reaction, for temperatures higher than $\sim10^7$ K, which corresponds to a certain bound for the average kinetic energy.
This kind of argument holds in general when you are talking about nuclear processes involving nuclei subject to the Coulomb repulsion, therefore all the further stages of the stellar nucleosynthesis or the various processes of the Big Bang nucleosynthesis (the formation of nuclei in the firsts minutes of our universe).
In the end, the answer to your question is kinetic energy, which can be gained from anything that can make the two protons (or any particle/nucleus) overtake their Coulomb barrier, whether it is electromagnetic fields, such as in particle accelerators (LHC at CERN, etc.), or gravitational force, as in astrophysical environments.
