If the extent of our observable universe at a point in time is determined by the particle horizon, and the future horizon tells us the point where we will never be able to see anything beyond is there a way to calculate when these two horizons will meet and thus the greatest extent of the particle horizon? Will the particle horizon shrink before it reaches the future horizon?
1 Answer
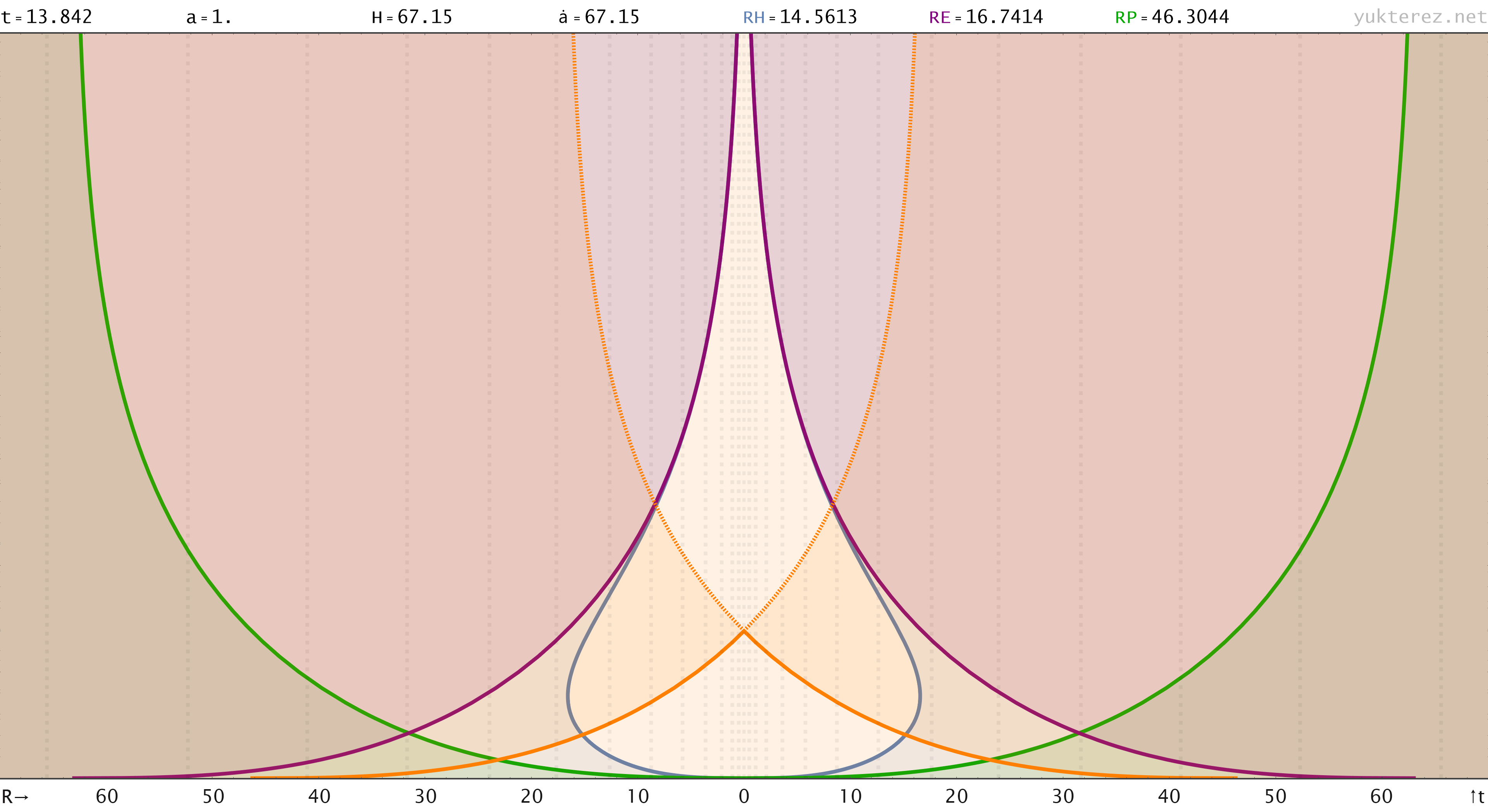
Henry Smith asked: "What is the greatest extent of the particle horizon?"
The maximum comoving distance from which any information could ever reach us is the particle horizon $\rm R_p=r_p/a$ at infinite future
$$\rm R_p(\infty)=63.046 \ Gigalightyears$$
That is assuming that the relevant cosmological parameters are
$$ \rm H_0=67.150 \ km/s/Mpc, \ \Omega_r =9.2136 \cdot 10^{-5}, \ \Omega_m=0.315, \ \Omega_{\Lambda}=1-\Omega_r-\Omega_m$$
If you assume different cosmological parameters this number of course changes, then you need
$$ {\rm r_p(t) = a(t) \ \int_0^t} \ \frac{\rm c}{{\rm a}(\bar{t})} \ {\rm d} \bar{t} \ \to \ {\rm r_p(a) = a \ \int_0^a} \ \frac{\rm c}{\bar{a}^2 \ {\rm H}(\bar{a})} \ {\rm d} \bar{a} $$
for the particle horizon $\rm r_p$ in proper distances, and the comoving one is $\rm R_p=r_p/a$ (see the green curve in the comoving spacetime diagram below)
Green: particle horizon, purple: event horizon, blue: hubble radius, orange: past & future lightcone
Henry Smith asked: "Will the particle horizon shrink before it reaches the future horizon?"
That will not happen, the particle horizon will expand forever, going to infinity in proper distances and converging to the aforementioned value in comoving distances.
Henry Smith asked: "Is there a way to calculate when these two horizons will meet?"
The particle horizon and the event horizon (these two are mirror images of each other in comoving coordinates) at
$$ {\rm r_e(t) = a(t) \ \int_t^{\infty}} \ \frac{\rm c}{{\rm a}(\bar{t})} \ {\rm d} \bar{t} \ \to \ {\rm r_e(a) = a \ \int_a^{\infty}} \ \frac{\rm c}{\bar{a}^2 \ {\rm H}(\bar{a})} \ {\rm d} \bar{a} $$
(and $\rm R_e=r_e/a$) if you mean that, had the same distance at
$$\rm t=4.2298 \ Gyr, \ a=0.39702$$
which was at a proper and comoving radius of
$$\rm r_p=r_e=12.513 \ Glyr, \ \ R_p=R_e=31.518 \ Glyr$$
see the diagram where the green and purple curves cross each other (the present time $\rm t=13.842 \ Gyr$ is where the past and future lightcone emerges).