Movement of the point $A$ on the cylinder [closed]

Question

We have a point $A$ on the top of a cylinder with radii $1$.After $2.5$ rolls find the movement of point $A$.(Take $\pi=3$ if needed)

For finding the movement of point $A$ we should use pytagoras to find the movement but I had a disscustion with my teacher about the value of $A'B$ I strongly belive that $A'B=2.5*permiter=2.5*2*\pi=15m$ but our teacher tells that $A'B=2.5*2radii=10m$ I don't know why but I strongly belive my answer.So we decided to ask it here to get an answer .A video will be helpful a lot but I don't know how to make one.Please make for me one or tell me how to make one.Thanks.

Answer 1

When a circle (or a cylinder) is rolled, it completes one full cycle when point $A$ gets back to its original position relative to the center of the circle, That means it has rotated $2\pi$ radians from its original position relative to the center of the circle. When this one cycle is completed circle travels a distance that is equal to its circumference. Formula of the circumference of the circle is defined as following:

$$C = 2\pi r$$

Now to calculate the distance the circle has traveled $(D)$ we have to multiply the number of cycles $(n)$ the circle has completed with the circumference of the circle, Thus we get a following formula:

$$D = Cn \rightarrow D=2n\pi r$$

Now to find out what is the distance from $B\rightarrow A`$: We know that Number of cycles $(n)$ is equal to $2.5$ and radius is equal to $r = 1 $, We plug in this parameters into the formula we derived above:

$$B\rightarrow A` = 2 \pi n =5\pi \approx 15.7 $$

We can derive $d$ from Pythagorean theorem:

$$d=\sqrt{(2r)^2+(B\rightarrow A`)^2} \rightarrow d = \sqrt{250.49} \approx 15.8$$

I do not know why your teacher multiplied the radius with the number of cycles to get the distance the circle traveled, Maybe your teacher was trying to calculate something else.

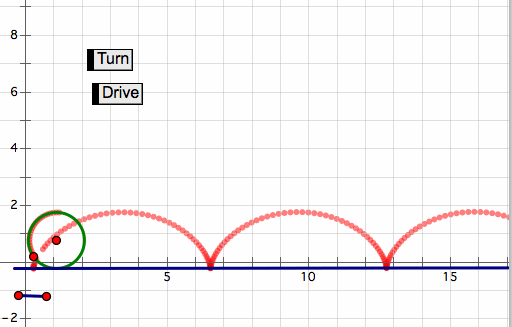
When this circle is rolled point A's trajectory looks like the following image which is called cycloid:

If you are interested, I suggest you watch this video about Brachistochrone Problem

Answer 2

As you've written down, $d = \sqrt{|AB|^2 + |BA'|^2}$ holds. Obviously $|AB| = 2$m, that means radius of the cylinder base is $r = 1$m. Perimeter is $2\pi$ then.

Now the problem could be in the fact, that generaly $O = 2\pi r$. Correct answer is $|BA'| = \frac 52O = 5\pi r$ and the final answer to the problems is $d = \sqrt{4r^2+25\pi^2r^2} = \sqrt{4+25\pi^2}r$. If we set $\pi = 3$, then $|BA'| = 15$m and finally $d \doteq 15.13$m. Using the correct definition of $\pi$ we'd get $d\doteq 15.8$m.

I think it should have been noticed that generaly we've got formula $$ d = r\sqrt{4+25\pi^2} \doteq 15.8\cdot r, $$ what means that ratio $\frac dr$ is conserved. We can generalize it by considering rolling about $\frac l2$ instead of $\frac 52$, than we'd get $d = r\sqrt{4 + l^2\pi^2}$.

I've found this nice picture where it can be seen, that the distance in $x$-axis can't be only $2r$, but $2\pi r$, in this case $r=1$m just like in given problem.

If still not enough, I'm adding these youtube video about cycloid https://www.youtube.com/watch?v=ohP0clzK5rc. It's complicated, but the start answers to your question, one "roll" is $2\pi$s could be seen.