Why do headphones tangle themselves whereas shoe laces untie themselves?

Question

Why do headphones tangle themselves whereas shoe laces untangle or untie themselves? Why aren't we using the phenomenon to create headphones that tangle less and shoe laces that untie less?

Answer 1

The basic problem is that headphones are heavy and asymmetric, whereas there's nothing attached to the shoelace.

What this means is that the headphone - cord system can get stuck in stationary, locally but not globally minimum energy configurations: the twisting of a cord raises the energy of the cord, but the torsion resulting from the twist is not enough to flip the headphones over when they are on your head or put down on the table; otherwise put, to find the lowest energy state, the headphone - cord system's gravitational potential energy would need to be raised enough so that the headphones could flip. There would be much less tendency to tangle if we had a cord attached to a heavy sphere: the torsion would tend to roll the sphere around on a flat surface to relax and dissipate the elastic potential energy stored in the twisting. The shoelace has a much smaller energy threshold to undo it.

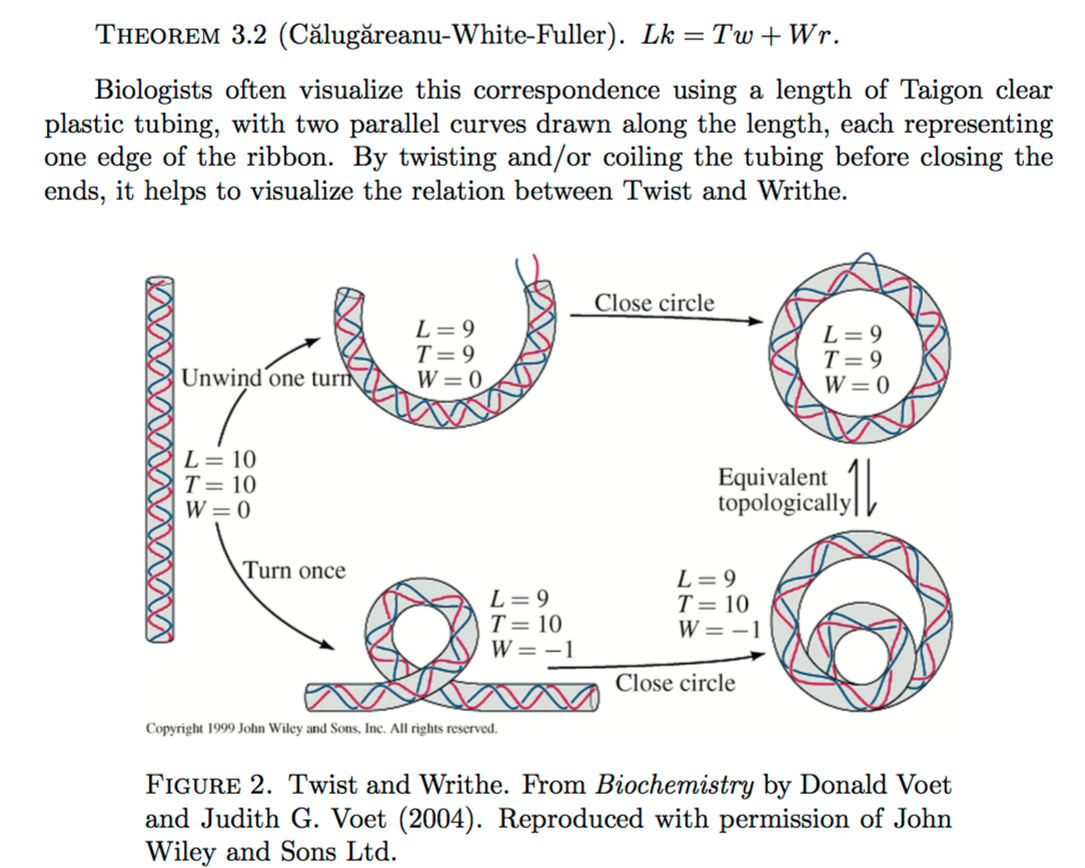
The tangling is a manifestation of supercoiling or writhing. The following is a topological invariant in a fibered loop (Calugareanu-White-Fuller Theorem):

$$T + W - L$$

To understand this invariant, imagine taking two long straight fibers, or a ribbon. Now twist the straight entity $T$ half turns, bring the ends of the composite entity together and glue the corresponding fibers / ribbon edge. E.g. you do this procedure with $T=1$ to get a Möbius strip. However, the number of times each strand crosses the other (the "Linking Number" $L$ can be traded off between the quantities twist and writhe, whose intuitive understanding is best given by the following:

taken from:

Dorothy Buck, "DNA Topology" ,Proceedings of Symposia in Applied Mathematics Volume 66, 2009.

Often in elastic physical systems, writhes represent lower energy than twist, so not all members of $T+W-L$ topological equivalence classes are equivalent from an elastic potential energy standpoint. So the tethered cord (as with the asymmetric headphones or a landline telephone receiver) relaxes by converting the links into writhes.

See Dorothy Buck's paper and also her website: she has done quite extensive research on twisting / writing / linking topology.

Answer 2

My proposed explanation is in the thickness. We wish the headphone wires thin and shoelaces thick. The former favors fixing sharp bends by entangling them randomly, the latter favors straightening them. If we tolerated 5 mm thick nylon yarn around our headphones, they would certainly tangle much less.