Radioactive decay of uranium-238

Question

Problem

We have a cubic room of side $10\rm\ m$, into which no fresh air has been allowed to flow for a week. We register a specific activity of radon $(^{222}\rm Rn)$ of $50\ \rm Bq\,m^{-3}$. Knowing that $^{222}\rm Rn$ is a product in the $^{238}\rm U$ chain, we would like to find the concentration of $^{238}\rm U$ in the walls of this room. We take for granted that the radon diffuses through a $3\rm\ cm$ thick layer of wall.

My attempt

I assume that the activity concentration of the radon is the same as that of the uranium from which it's coming. I compute the activity of the radon using $A = (50\rm\ Bq\,m^{-3})(10^3\ m^3)= 50 000\ Bq$. Then taking one of the four walls, the concentration of uranium is given by the activity per unit volume in the thin layer through which the radon diffuses, that is, $C = (50 000\ \rm Bq)/(10 \times 10 \times 0.03\ m^3)$.

I think this approach is flawed. Any hint towards a more reasonable solution will be appreciated.

Answer 1

One more assumption must be made regarding the $^{222}Rn$ concentration at time zero (week ago). If you assume radioactive equilibrium between $^{222}Rn$ and the parent $^{238}U$ you can proceed like you did. But instead of taking one wall, you should take into account all the walls made of the $^{238}U$ contaminated material.

On the other hand, if you assume that the room was well ventilated until week ago, i.e. the $^{222}Rn$ concentration in the air was zero at the beginning, you must take into account the activity build-up. Starting with zero activity, $^{222}Rn$ will gradually grow until the equilibrium with parent radionuclide is reached (it takes approximately one month in this case). Let's assume the radioactive equilibrium between the $^{238}U$ and its decay products exists until $^{226}Ra$ (which decays to the $^{222}Rn$). The decay-growth equations describing such situation are:

$ \frac{dN_1}{dt}=-\lambda_1N_1 $

$ \frac{dN_2}{dt}=-\lambda_2N_2+\lambda_1N_1 $

Where $N_1$, $N_2$ are numbers of atoms of $^{238}U$ and $^{222}Rn$ respectively (as a functions of time) and $\lambda_1$, $\lambda_2$ are decay constants of the $^{238}U$ and $^{222}Rn$ respectively.

Solution to this system of equations is

$ N_2=\frac{N_0 \lambda_1}{\lambda_2-\lambda_1} (e^{-\lambda_1 t}-e^{-\lambda_2 t}) \Rightarrow A_2=A_1 \frac{\lambda_2}{\lambda_2-\lambda_1} (e^{-\lambda_1 t}-e^{-\lambda_2 t}) $

Since $\lambda_2= 2.1 \times 10^{-6} \ s^{-1} \gg \lambda_1= 4.9 \times 10^{-18} \ s^{-1}$ and $1 \ week \doteq 6 \times 10^{5} \ s$ we can approximate this solution as

$ A_2 \doteq A_1(1-e^{-\lambda_2 t}) $

where $t$ is a time of $^{222}Rn$ "building up" in seconds and $A_1$, $A_2$ are activities of $^{238}U$ and $^{222}Rn$ respectively and $N_0$ is the initial number of $^{238}U$ atoms. It means, that after a week, the $^{222}Rn$ will grow to the ~ 70 % of its equilibrium activity, see the figure:

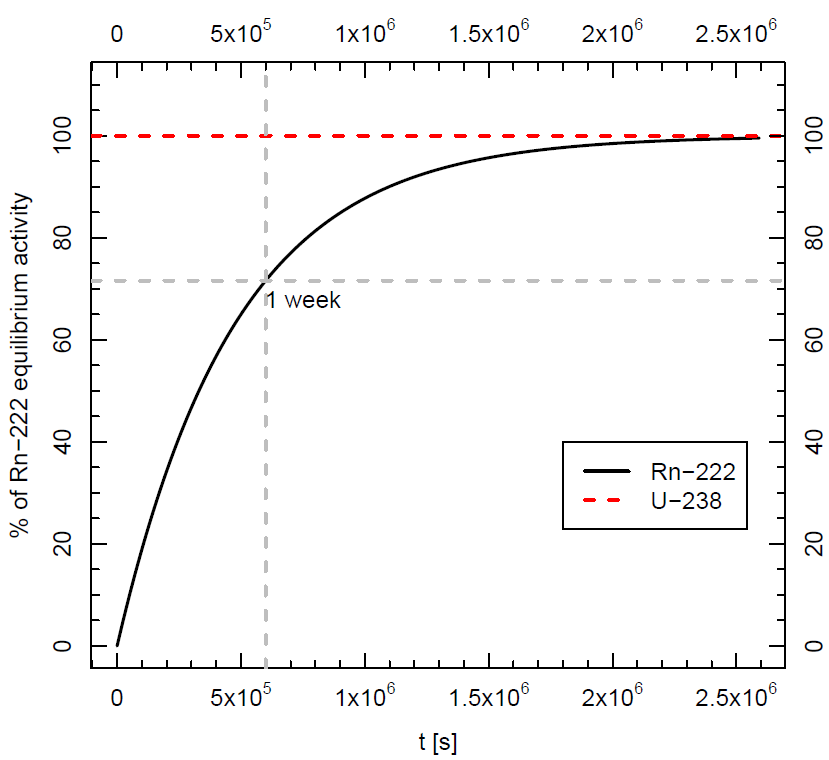
Assuming, that $^{222}Rn$ emanates from all of the walls including the floor and the ceiling, we obtain the activity $A_1$ of $^{238}U$ per unit volume of a wall material as

$ A_1 =\frac{A_2 V_{air}}{V_{material}(1-e^{-\lambda_2 t})}=\frac{50 \times 10^3}{6 \times 10^2 \times 0.03 \times (1-e^{2.1 \times 10^{-6} \times 6 \times 10^5})} \doteq 3900 \ Bq/m^3 $