According to Wikipedia lift in an aircraft is due to an area of low pressure formed above the wings of an aircraft due to the fast moving air there. So why exactly is an area of low pressure created due to fast moving air?
-
3$\begingroup$ You can simply Google it. Do some prior research $\endgroup$– N.S.JOHNCommented Dec 30, 2015 at 15:03
-
$\begingroup$ Have a look at en.wikipedia.org/wiki/Bernoulli%27s_principle $\endgroup$– Gyro GearlooseCommented Dec 30, 2015 at 15:28
-
$\begingroup$ Bernoulli only applies in a contained environment, like a tube. It does not apply significantly in open air. $\endgroup$– Carl WitthoftCommented Dec 30, 2015 at 20:59
-
$\begingroup$ @CarlWitthoft I admit that I understood Bernoulli ever only in the sense of "any monkey could do the computation", never had a deeper understanding, and whenever I thought about it, I had more questions/doubts than answers/proofs/explanations. $\endgroup$– Gyro GearlooseCommented Dec 30, 2015 at 21:49
-
$\begingroup$ Related: physics.stackexchange.com/q/290/59023 $\endgroup$– honeste_vivereCommented Feb 24, 2021 at 0:05
11 Answers
It's all about conservation of momentum, $F=ma$. Fluid can only change velocity by experiencing a force, and the only force it can feel is a pressure difference. So if there's a velocity difference, there's a pressure difference, and vice-versa (neglecting other things, like gravity). Read this beautiful exposition.
If wikipedia says that, it's wrong. There is no physical requirement for the air to move faster or slower on either side of the wing. The best analogy I've found so far is to think of two layers of cotton batting, or two blankets, of infinite length. The aircraft wing separates them, but there is no need for the blankets to "line up" after the wing has passed.
If you read any half-decent text on aerodynamics, you will find that lift is almost 100% due to the angle of attack. The air below the wing is forced downwards, so momentum conservation forces the wing upwards. Aircraft can and have flown just find upside down.
The shape of the wing, with camber and trailing edge taper, etc., is almost entirely designed to minimize drag, eliminate stall vortices, and all sorts of other messy mathematical problems in turbulent flow. Lift is absolutely NOT due to reduced air density on top of the wing.
-
3
-
1$\begingroup$ @MikeDunlavey, yes, but if you read that carefully it points out that Bernoulli does not apply there. Of course there's a pressure difference, but not because the air is forced to move faster. Further, the lift comes from the air below the foil being forced downwards (yes this force does increase the pressure on the air below the wing). Read the rest of the page, which says quite clearly what I said above, plus the details on streamline analysis to avoid flow tear, stagnation stall, and other undesirable turbulent effects. $\endgroup$ Commented Jan 2, 2016 at 19:56
-
2$\begingroup$ Here it's more explicit. The air being deflected downward is not just the air below the wing, but also the air above the wing. In particular, anything that disturbs the flow on top of the wing, like a thin layer of frost, kills lift badly. Big cause of accidents. Also this. Note emphasis on top of the wing. $\endgroup$ Commented Jan 2, 2016 at 23:53
-
$\begingroup$ A counter experiment is blowing pin-pon ball on a tube. The harder you blow, the ball tend to get tighter to the tube open. The thery of attack angle seems fails to explain this simple demo. $\endgroup$– ytluCommented Feb 21, 2021 at 17:25
-
$\begingroup$ I think it is worth mentioning that a change in momentum of air is not absolutely required in order to get lift. Think of a balloon for example. But I admit the momentum change may be the biggest contribution for many aircraft. $\endgroup$ Commented Feb 22, 2021 at 11:28
There are two main explanations, since I don't know your education level, first I will explain it in layman's terms even though this is not fully correct but you can encounter this explanation in some maybe most of the undergraduate physics textbooks. Then I will explain why this picture is wrong and how to fully understand the physics behind aircrafts.
- Airplane wings are designed in such a way that they are more bulgy at the top of an airplane's wing compared to the bottom, this plays a significant role. Have a look at the illustration:
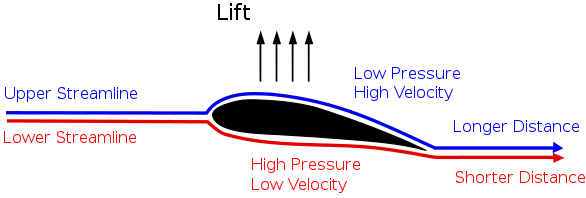
Air molecules at the top of the airplane's wing should move faster than those at the bottom to meet at the back edge of the wing. Bernoulli's principle written as
$$P_1 +\rho g h_1 +\frac{1}{2} \rho v_{1}^2 = P_2 +\rho g h_2 +\frac{1}{2} \rho v_{2}^2$$
where subscript $2$ refers the bottom and subscript $1$ refers the top of the wing. Since the thickness of the wing thus the difference $h_2-h_1 \approx 0$ one can cancel those terms out and obtain
$$P_1 +\frac{1}{2} \rho v_{1}^2 = P_2 +\frac{1}{2} \rho v_{2}^2 $$
$$P_2-P_1 = \Delta P = \frac{1}{2} \rho (v_{1}^2-v_{2}^2)$$
Then (lifting) force on the aircraft
$$F=\Delta P \cdot A = \frac{1}{2} \rho (v_{1}^2-v_{2}^2)\cdot A$$
This also gives a good intuition why aircrafts malfunction when they reach a certain altitude since $\lim_{\Delta P \to 0} F =0$ so there will be no lift.
- $\textbf{1.}$ This above explanation is not fully correct because even when the length of the airplanes' wings were the same at the top and at the bottom, there would be a lift (consider paper-planes where you don't have bump on top of the wing).
- $\textbf{2.}$ Experiments have shown that the air molecules at the leading edge (from the top and bottom of the of the wing) do not meet at the end of the wing.
To have a complete understanding of the physics behind the working mechanism of aircrafts one should solve the Navier-Stokes equations. Since Navier-Stokes equations are generally too complex to solve, one can use an approximation by solving Euler equations which doesn't take the viscosity into account.
They then further simplify the Euler equations by assuming that compressibility is negligible. Thus in incompressible form, density $\rho$ is constant thus it oversimplifies to
$$\frac{\partial u}{\partial x}+\frac{\partial v}{\partial y}=0$$
$$u\frac{\partial u}{\partial x}+v\frac{\partial u}{\partial y}=-\frac{1}{\rho}\frac{\partial p}{\partial x}$$
$$u\frac{\partial v}{\partial x}+v\frac{\partial v}{\partial y}=-\frac{1}{\rho}\frac{\partial p}{\partial y}$$
But even these oversimplified set of differential equations are difficult to solve therefore one solves them numerically, write a program to simulate the behavior of air molecules at the top and bottom of the wings.
-
1$\begingroup$ The diagram in this answer is wrong, because in fact the air above and below does not cross the wing in the same time and then rejoin. $\endgroup$ Commented Feb 22, 2021 at 11:24
-
2$\begingroup$ I already stated this fact multiple times in my answer and once separately in 2. $\endgroup$– MonopoleCommented Feb 22, 2021 at 17:20
-
3$\begingroup$ Oh yes I see, but surely it is much better not to display an incorrect diagram than to display it and then say it is incorrect. $\endgroup$ Commented Feb 22, 2021 at 17:53
-
$\begingroup$ @AndrewSteane, it is a good guess that the streamlines below and above the airfoil do not intersect, so that applying Bernoulli's equation to points on the two streams directly may not be technically accurate. (1/2) $\endgroup$ Commented Mar 17, 2021 at 7:14
-
$\begingroup$ @AndrewSteane, however, from a practical viewpoint, consider a point designated $3$ on a streamline upstream of the airfoil which divides into the lower and upper flows. In that case Bernoulli's equation (neglecting the static head due to gravity) applied to the two streamlines (3-to-1 and 3-to-2 separately would yield $P_3 + \frac{1}{2}v_3^2 = P_1 + \frac{1}{2}v_1^2 = P_2 + \frac{1}{2}v_2^2,$ thus making the following analysis accurate. (2/2) $\endgroup$ Commented Mar 17, 2021 at 7:14
There are two different issues here:
(1) how do wings generate lift?
(2) what is the physics underlying a connection between flow velocity and pressure in a fluid?
I will answer just (2). The connection is conservation of energy.
To state the connection correctly, you must not look at different places across the fluid; you must pick a flow line and follow it along (in conditions of laminar, i.e. smooth, flow). As you move along this flow line, if the pressure goes up then the velocity goes down, and if the pressure goes down then the velocity goes up. Put like this, it becomes, I think, reasonably intuitive: when moving from a lower to a higher pressure, there is a pressure gradient opposing the flow, so the flow will naturally slow down. To calculate this one calculates the work done by the pressure forces on either side of any particular parcel of fluid. The parcel of fluid gains or loses kinetic energy equal to the difference between the two amounts of work.
This connection goes by the name Bernoulli's principle. I think the reason why it puzzles people is because it is often presented the other way round: it is said that when the fluid speeds up its pressure falls, or it is said that the fall in pressure is owing to the faster speed. I think this way of putting it introduces a muddle between cause and effect. It is clearer to say that if there is a pressure difference then the fluid must respond accordingly. But the emphasis on velocity can be useful when one is doing detective-work to find out what a given fluid is doing. When one observes laminar flow and one finds that the fluid is accelerating along some of its flow lines, then one can deduce that there is a pressure gradient along those flow lines.
All this does make a modest contribution to the physics of flight, but in that case there are other issues to think about as well. In particular, it is debatable whether the laminar flow approximation is ever good enough to describe an aerofoil, even to first approximation.
Moving air can have any pressure. However if air is moving away from a surface without enough new air flowing in to replace it, then the pressure at that surface drops. For a wing with lift the cause is inertia / momentum conservation. Since the air collides at higher rate with other parts of the wing there is a net force, hopefully up.
First, we need to talk define some terms in a way that is useful to this particular discussion. These are not the most common general definitions.
Temperature is a description of the amount of kinetic energy a substance has. It's the average speed (not velocity) of the particles of air. In "still" air, these particles are moving in totally random directions. Some of them are going really fast, some of them are going slow, some of them are around the average. Average speed is some number proportional to temperature, average velocity is 0 (because the particles are moving in random directions).
Pressure is a force exerted by a substance's particles on a surface. In this case, it's a result of air particles that happen to traveling towards a surface striking it and bouncing off.
Consider a cube of air. For simplicity, let's pretend all particles have exactly the average speed, "T".
When air is still, particles are moving in random directions, and each wall gets hit by particles with about the same frequency and same speed. Each wall experiences the same pressure.
Now let's make the air all move to the right, but keep the temperature the same. Now the average speed of particles is the same, but the direction they're traveling is no longer completely random. They're all moving a little to the right in addition to the direction they were going. Particles that were moving directly left are moving left a little slower. Particles moving to the right are moving to the right a little faster. Particles that were moving down are now moving down and to the right, at an angle. It's that angle that's really important here. A particle that would have hit the bottom wall with "T" speed is now hitting it with speed=sin^-1(speed to the right/T)*T, which is less than T.
The left, side, top, and bottom walls are getting hit with less force (less perpendicular particle speed), while the right wall is getting hit harder. In a closed box, this can't last, but in the case of the airplane wing, most of the walls are open.
I don't know if it helps or not but give it a try
For this to understand let us consider a room in place of a hose say of length l in which a ball is bouncing up and down and colliding elastically with the ceiling and the floor continuously. Now if we increase the speed of the ball in this upward or downward direction using some techniques, the ball will strike the ceiling and the floor with a greater momentum and thus the change in momentum will also increase and hence will apply a greater force and hence pressure on the ceiling and the floor will increase.
But what if we push the ball in the horizontal direction ?
If we give it a appreciable horizontal speed , it will rarely strike both the walls before coming out of the room and hence we can conclude that the pressure on the ceiling and the floor has decreased with increase in kinetic energy.
The above statements are taken from this answer of mine.
And I think that because of more hitting of molecules (present below the paper) than that above it, the paper goes up.
As with most "why" questions, there are multiple possible answers, and the one that's most satisfying depends on the person. The following is one that appeals to me.
First, we are talking about the Bernoulli principle, which strictly applies only to a very limited class of flows: steady (unchanging in time), inviscid (no viscosity, so no dissipation and no transfer of momentum across streamlines), and incompressible.
What the principle says is that the total energy (kinetic plus potential) of a fluid parcel remains constant as it moves along a streamline (a parcel is just a little box of fluid--you could mark it with dye to keep track of it). To keep things as simple as possible, we will say there is no gravity, so that the potential energy of a bit of fluid is just its pressure ($P$) times its volume ($V$); the kinetic energy meanwhile is $E_k=\frac{1}{2}\rho V v^2$, where $\rho$ is the density and $v$ the velocity. [To see that $PV$ is the potential energy, just imagine using the pressure to launch a plug of fluid with cross sectional area $A$ and length $L$ such that $V=AL$, starting from rest; the force is $PA$ and the distance through which it acts is $L$, so the work done (force times distance) during the launch is $PA\cdot L=PV.$]
So, what we would like to understand is why the total energy of a fluid parcel remains constant as the parcel moves through the flow field.
We (or at any rate I) are very accustomed to the idea that the total energy of a particle moving under the influence of only gravity (no drag) is constant. If the particle goes up, it gains potential energy and loses kinetic energy; if it falls back down, that potential energy is converted back to kinetic. This property is true for a particle moving through any static, conservative force field (if it isn't static, you can't apply conservation of energy to the particle on its own; conservative just means that the curl of the force field is zero--otherwise you could gain energy by going in a loop). If you buy all this, it's time to get back to our fluid parcel.
The force that causes the parcel to change its velocity is the pressure difference across it, which, for a sufficiently small parcel, is just the pressure gradient force, $\nabla P$--it pushes the parcel in the direction that pressure decreases most rapidly. Since the flow is stipulated to be steady, the pressure gradient force is unchanging in time. Since it's the gradient of a scalar, it is curl free. As we just established, that means that the thing that it acts on (the fluid parcel) always keeps the same total energy.
We have shown that the total energy of the fluid parcel $$E_{tot}=E_k+E_p=\frac{1}{2}\rho V v^2 + PV$$ remains constant. As we are assuming the fluid is incompressible, $V$ is a constant, and any change in $P$ is balanced by an opposite change in $v^2$: along a streamline (that path along which the parcel travels), higher pressure means lower velocity, and vice versa.
Since I have made several programs for lift and drug force acting on airfoil computation, I can explain my suggestion about how lift force generates. First of all, there is wrong explanation in Wikipedia about it. When we compute force we put some boundary condition for air on the top and bottom surface of airfoil. This condition is very simple and it turns all suggestion about air velocity on top and bottom surface of airfoil to this one $\vec {v}=0$ in the aircraft coordinate system. Therefore there is no difference in velocity at all and it breaks the theory about Bernoulli principle and energy conservation. Nevertheless we have some pressure difference on the top and bottom surface, but this difference is mostly due to flow structure around airfoil. It depends on angle of attack and mostly looks like on pictures presented on
Drag and lift force acting on an airfoil
How can we improve transonic flow visualization?
Plotting Joukowski Airfoil Streamlines using conformal maps
On the last picture from the last post we can see red zone past to airfoil and this is main reason for the pressure difference.
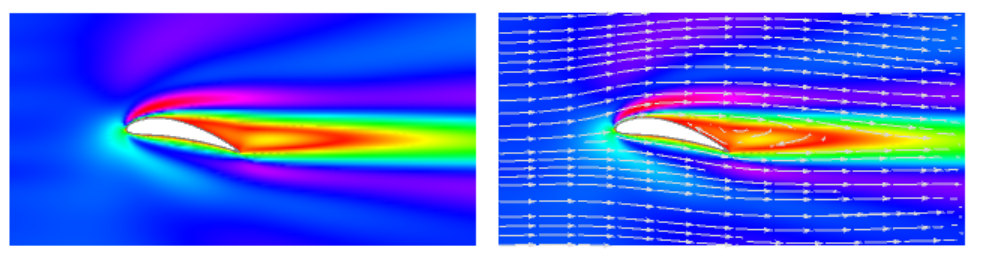
When an aircraft starts from the ground it has very higher angle of attack and flow around wings looks like on the left picture below, while on the right picture there is pressure distribution shown. Apparently we have low pressure on top and high pressure on bottom surface, but velocity distribution contradicts to Bernoulli principle.
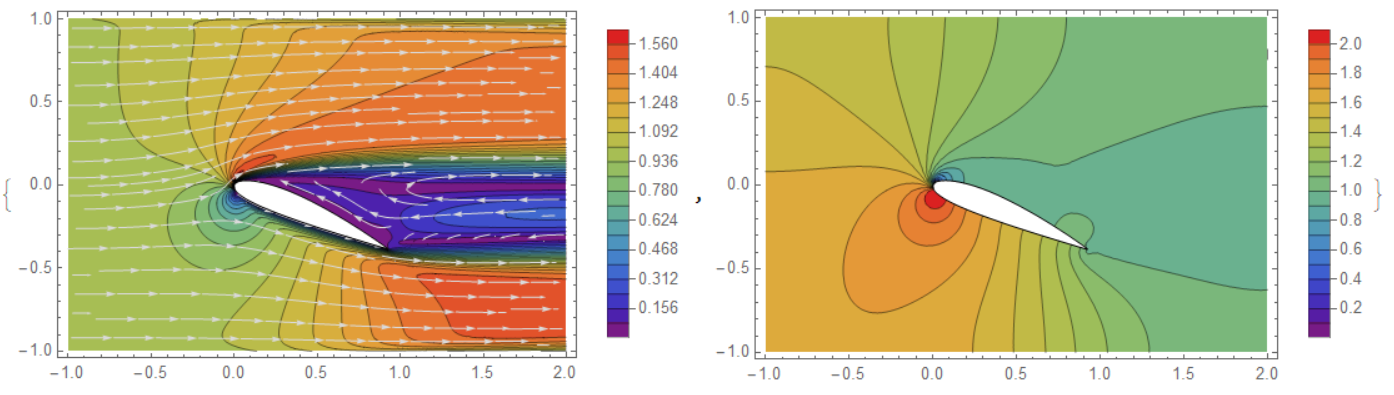
-
$\begingroup$ The reason for the pressure distribution in the lower right-hand image is because ram pressure acts normal to the surface with which it is incident, not transverse. That is, the air incident on the airfoil hits just below the leading edge and is deflected downward. This creates a large ram pressure gradient, which is ultimately what gives much of the lift here (as I think you are implying in your answer). $\endgroup$ Commented Mar 17, 2021 at 15:15
-
$\begingroup$ @honeste_vivere You are absolutely right. We don't need Bernoulli principle to explain lift force. Due to angel of attack the air flow in the low part pushing airfoil up, and due to viscous effect in the upper part there is the recirculation zone with relatively low pressure. But for some airfoil we need other explanation like in this case on physics.stackexchange.com/questions/617962/… $\endgroup$ Commented Mar 17, 2021 at 15:56
-
$\begingroup$ The "lift" in that bad wedge example you linked to would be due entirely to the Kutta–Joukowski theorem. I put lift in quotes because I very much doubt there would be net lift, as my answer to that question states ;) $\endgroup$ Commented Mar 17, 2021 at 16:42
-
$\begingroup$ @honeste_vivere I have computed viscous flow around triangle airfoil (we don't need Kytta-Jukowski theorem in this case) . There is the lift force acting on this airfoil at low Reynolds numbers of about 100-1000, but lift force decreases with Reynolds number increasing. $\endgroup$ Commented Mar 17, 2021 at 17:07
-
$\begingroup$ I am not sure what you mean by "we don't need Kytta-Jukowski theorem in this case..." If the simulation is self-consistent, then the KJ theorem will apply, will it not? That is, it's not something you "turn on" or "turn off" in a self-consistent fluid simulation. It results due to rotational flow around an object in a fluid, kind of like the Magnus effect but without the object rotating. $\endgroup$ Commented Mar 17, 2021 at 18:16
Because when a stream of moving air attached to a curved wing is made to change direction, it sucks. The air wants to move in a straight line, but the surface underneath it is curving away from the flow. So it tends to create a partial vacuum.
-
$\begingroup$ Could you please explain why this effect should have anything to do with the air changing direction? $\endgroup$ Commented Dec 30, 2015 at 16:09
-
$\begingroup$ I get it Gyro. If air was thick and syrupy it would leave the surface of the wing behind. Only it isn't, so it's only a partial vacuum. $\endgroup$ Commented Dec 30, 2015 at 18:17
Here is something to help us imagine the reason from first principles. We can assume that the air above the paper and the air below have equal temperatures. This can happen if the velocity of the 'blow' isn't too high. Then by the time the moving air reaches the area above the paper, its temperature has equalized with the surroundings.
The kinetic energy of each molecule $KE=(3/2)kT$ where $k$ is the Boltzmann constant and $T$ is temperature. Then $(1/2)mv^2 = (3/2)kT$ and the average speeds of molecules above and below the paper are equal.
However, since molecules in the air above the paper have a horizontal component of velocity, they'll have a lower vertical component, on average, than molecules under the paper (so that the combined speed, from Pythagoras theorem, is the same).
The ones above the paper then hit less hard on the paper vertically than the ones below and the paper is pushed upwards.
