There are two main explanations, since I don't know your education level, first I will explain it in layman's terms even though this is not fully correct but you can encounter this explanation in some maybe most of the undergraduate physics textbooks. Then I will explain why this picture is wrong and how to fully understand the physics behind aircrafts.
- Airplane wings are designed in such a way that they are more bulgy at the top of an airplane's wing compared to the bottom, this plays a significant role. Have a look at the illustration:
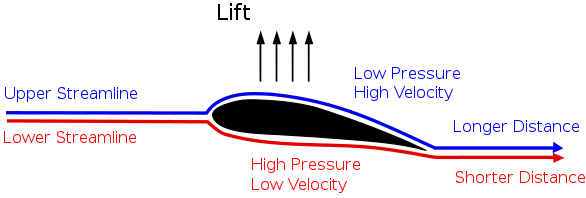
Air molecules at the top of the airplane's wing should move faster than those at the bottom to meet at the back edge of the wing. Bernoulli's principle written as
$$P_1 +\rho g h_1 +\frac{1}{2} \rho v_{1}^2 = P_2 +\rho g h_2 +\frac{1}{2} \rho v_{2}^2$$
where subscript $2$ refers the bottom and subscript $1$ refers the top of the wing. Since the thickness of the wing thus the difference $h_2-h_1 \approx 0$ one can cancel those terms out and obtain
$$P_1 +\frac{1}{2} \rho v_{1}^2 = P_2 +\frac{1}{2} \rho v_{2}^2 $$
$$P_2-P_1 = \Delta P = \frac{1}{2} \rho (v_{1}^2-v_{2}^2)$$
Then (lifting) force on the aircraft
$$F=\Delta P \cdot A = \frac{1}{2} \rho (v_{1}^2-v_{2}^2)\cdot A$$
This also gives a good intuition why aircrafts malfunction when they reach a certain altitude since $\lim_{\Delta P \to 0} F =0$ so there will be no lift.
- $\textbf{1.}$ This above explanation is not fully correct because even when the length of the airplanesairplanes' wings were the same at the top and at the bottom, there would be a lift (consider paper-planes where you don't have bump on top of the wing).
- $\textbf{2.}$ Experiments have shown that the air molecules at the leading edge (from the top and bottom of the of the wing) do not meet at the end of the wing.
To have a complete understanding of the physics behind the working mechanism of aircrafts one should solve the Navier-Stokes equations. Since Navier-Stokes equations are generally too complex to solve, one can use an approximation by solving Euler equations which doesn't take the viscosity into account.
They then further simplify the Euler equations by assuming that compressibility is negligible. Thus in incompressible form, density $\rho$ is constant thus it oversimplifies to
$$\frac{\partial u}{\partial x}+\frac{\partial v}{\partial y}=0$$
$$u\frac{\partial u}{\partial x}+v\frac{\partial u}{\partial y}=-\frac{1}{\rho}\frac{\partial p}{\partial x}$$
$$u\frac{\partial v}{\partial x}+v\frac{\partial v}{\partial y}=-\frac{1}{\rho}\frac{\partial p}{\partial y}$$
But even these oversimplified set of differential equations are difficult to solve therefore one solves them numerically, write a program to simulate the behavior of air molecules at the top and bottom of the wings.
