Why are waveguide fields not infinite?

Question

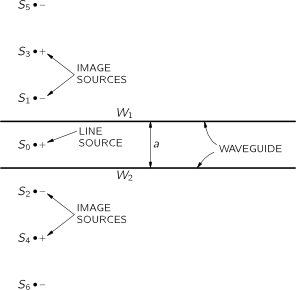
I was reading The Feynman Lectures on Physics, Volume II, when I got to the section on waveguides. Toward the end, Feynman uses the concept of an infinite number of alternating line sources to explain attenuation and propagation in the guide.

While I very much enjoyed this point of view, one problem did stick out in my mind. If we have an infinite number of sources, I agree the beam will zero out everywhere except at the special angles for which all sources constructively interfere (far from the sources). However, at this angle, wouldn't an infinite number of positively reinforcing waves produce an infinite field? Do the further sources produce weaker fields, making the sum finite?

Even if that's true, what if we consider the problem in terms of bouncing plane waves? Won't the transverse field components be infinite in extent and constant down the guide, setting up a standing wave pattern. But, since in an infinitely long guide there will be infinite in-phase reflections, shouldn't the resulting fields be infinite?

When we mathematically solve for the fields however, they seem to turn out perfectly finite, even in ideal conductors.

Where's the discrepancy? Any help would be greatly appreciated!

Answer 1

You're presumably referring to this construction:

Fig. 24–15.The line source $S_0$ between the conducting plane walls $W_1$ and $W_2$. The walls can be replaced by the infinite sequence of image sources

This does indeed produce a field which has infinite energy density, or more precisely, if you draw a plane parallel to the source wires then it has infinite power flowing through it. However, this is because you have an infinite number of copies of the waveguide you're trying to describe: each waveguide copy carries a finite amount of power (the same for all copies) and it becomes infinite when you count an infinite number of them.

There's nothing in the infinite-source case, though, which really requires that the fields themselves be infinite. (In fact, you know they will be regular inside each copy of the waveguide, because you can solve it for the actual waveguide (you've already done this) and show that the fields are finite and regular.)

Keep in mind that in the construction we're replacing the waveguide walls with the infinite collection of sources, so that there are no reflections in the infinite-sources case. This is the usual case with the method of images - we choose the image sources so that the fields will cancel out in the plates, and then we remove the plates. This is what seems to be tripping you up when you say things like

But, since in an infinitely long guide there will be infinite in-phase reflections, shouldn't the resulting fields be infinite?

There's no reflections - simply fields travelling through and interfering. The fields from $S_0$ will thin out (as they're no longer contained inside the waveguide) and their energy will be replaced by the fields from all the other sources.