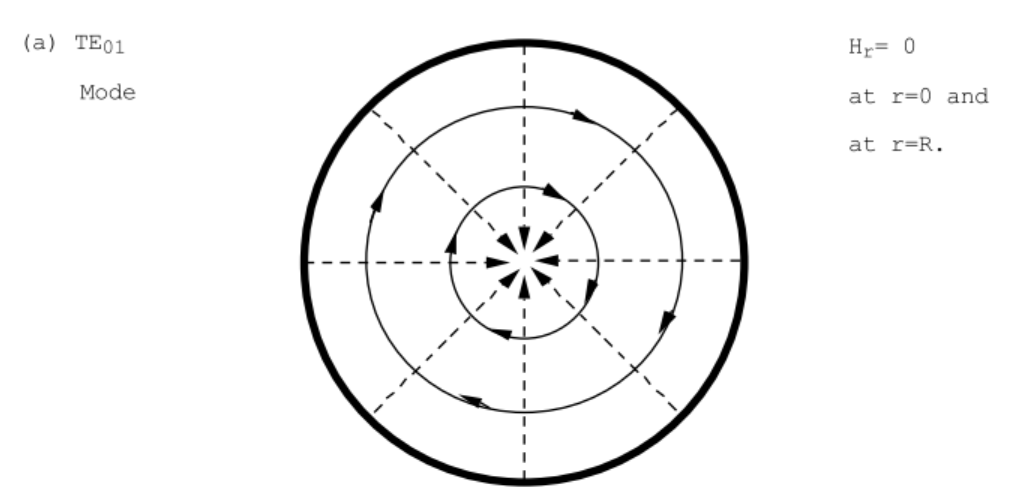
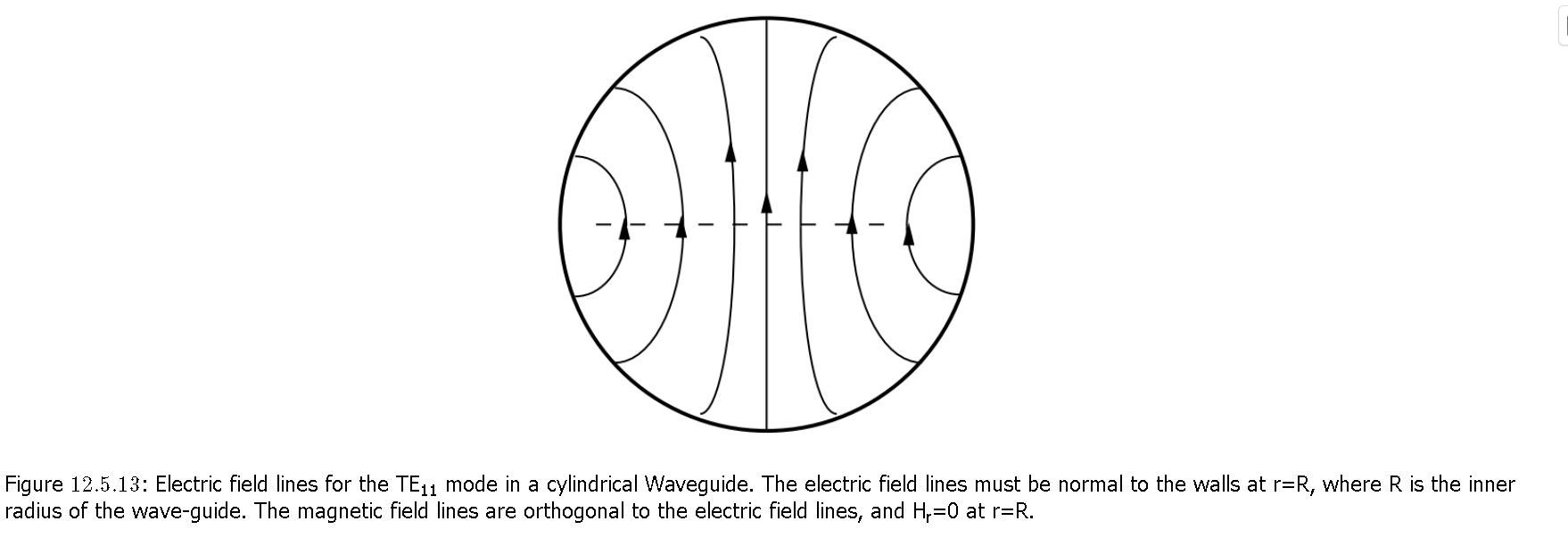
As we can see bellow TE01 and TE11 mode taken from the manual bellow. The fiirence between the two in the first index,but the shape is totally changed. Is there a logic regarding the shape of the mode with respect to the indexes?
$\begingroup$
$\endgroup$
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
2
In the reference you gave Equations 12.5.13 and 12.5.14 define the $TE_{mn}$ mode components. The index $m$ defines a series of modes having the same axial (angular) m-fold symmetry. The second index refers to the radial distribution of the field and is numbered by the roots of the $m^{th}$ Bessel function $J_m'(k_nR) = 0$ where $J_m'(x)=\frac{dJ_m(x)}{dx}$ and $R$ is the radius of the waveguide. This ensures the boundary condition on the inner metal surface $H_r(r=R)=0$.
answered Apr 27, 2023 at 14:13
-
$\begingroup$ Hello hyportnex so m is angular and n is radial. so for 01 there is no angular and radial is 1? i cant see it in the photo. also for TE 11 we have 1 angular and one radial,i cant see this data in the TE11 photo. could you please explain that? Thanks. $\endgroup$– lub2354Commented Apr 27, 2023 at 15:18
-
$\begingroup$ hint: the top plot for $TE_{01}$ is obviously circularly symmetric and thus $m=0$ and the 1st radial null is on the boundary with a single peak around the middle. There are better mode pictures than this, see, for example. I leave the other for you to think about. $\endgroup$ Commented Apr 27, 2023 at 15:44