We know photons have spin s=1. However, in Nuclear physics, the conservation of angular momentum in case of Gamma transitions is employed as follows: $$\vec J_i=\vec J_f+\vec L$$ where $J_i$ is the nuclear "spin" (total angular momentum spin+orbital contribution) of the parent nucleus, $J_i$ is the nuclear "spin" daughter nucleus and $\vec L$ is the orbital angular momentum of the photon. But we know that photons also carry spin angular momentum. Why we do not include the spin of the photon and write the conservation equation as $$\vec J_i=\vec J_f+\vec L+\vec S ?$$
$\begingroup$
$\endgroup$
4
-
1$\begingroup$ I believe $\vec L$ includes both spin and orbital angular momentum, which can't easily be decoupled for the photon. Photon spin is accounted for by the selection rule which says there is no transition matrix element for "monopole" ($L=0$) electronic transitions which preserve nuclear spin, only for dipole transitions and higher. $\endgroup$– rob ♦Commented Oct 5, 2015 at 19:04
-
$\begingroup$ @ rob- But it is also used to determine whether the photon is dipole type or quadrupole type etc using $2^L$, where $L$ is usually associated with $Y_{lm}$. $\endgroup$– SRSCommented Oct 6, 2015 at 8:38
-
$\begingroup$ Photon helicity (that is, spin) is at the heart of the Goldhaber et al. neutrino helicity measurement: $E1$ photons from polarized nuclei are circularly polarized. The spin argument in that paper is quite clear. $\endgroup$– rob ♦Commented Oct 6, 2015 at 15:37
-
$\begingroup$ Note that if you define $\vec J_\text{photon} = \vec L_\text{photon} + \vec S_\text{photon}$ it's still possible to have $J,L,S=1,1,1$. $\endgroup$– rob ♦Commented Oct 6, 2015 at 15:38
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
2
Massless particles aren't characterized by spin because square of Pauli-Lubanski operator (which is Casimir operator of Poincare group) for them is equal to zero. They are characterized by helicity.
Now, let's back to conservation law. We don't include spin of photon in conservation law, because we can't introduce it. Instead of it we require that quantity $\mathbf L$ may have values $1, 2, ...$
-
$\begingroup$ I don't understand this answer. Are you simply saying that $L$ is not only the orbital angular momentum but also includes the spin of the photon? (since photon is spin 1, adding its contribution to the orbital angular momentum would still give even numbers). $\endgroup$– PaganiniCommented Oct 5, 2015 at 18:19
-
$\begingroup$
$\endgroup$
In the reaction you write the photon takes away the angular momentum as its spin. Before the transition, the photon does not exist, so as to be included in the sum of quantum numbers. It appears at the transition as the carrier of the energy momentum and angular momentum .
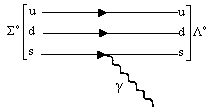
This is clear in simple Feynman diagrams , for example
the decay of a sigma_0 to a photon and a lamda_0
the quantum numbers are balanced at the vertex. The photon and the lamda spins , momentum and energy must add up to the sigma_0 mass and spin.
In the complex environment of nuclei this still holds.
