I think it might be a good idea to identify Thomson's 2009 lectures you are referring to. He actually explains the suppression of the remaining 12 combinations you appear to be worrying about, at high energies, $E\gg m_\mu\gg m_e$.
The QED action couples the electron to chiral combinations similar to the kinetic term, $$eA_\mu \bar{\psi} \gamma^\mu \psi= eA_\mu (\bar{\psi} L \gamma^\mu R\psi + \bar{\psi} R \gamma^\mu L\psi),$$
where L and R are the left and right projectors, respectively. That is, a photon couples L electrons to R positrons and R electrons to L positrons, only.
This is in sharp contrast to the mass terms for both electrons and muons, which
do the very opposite,
$$
m\bar{\psi} \psi = m(\bar{\psi}LL \psi +\bar{\psi}RR \psi),
$$
coupling L electrons to L positrons and R electrons to R positrons. So, the fermion propagators involving the masses introduce effective couplings upon radiative corrections (such as the vertex correction, magnetic moments, etc) which would couple all 4 initial with all 4 final state combinations. (You are already aware of this for spin triplet, J=1 orthopositronium, but also spin singlet, J=0 parapositronium.)
You also know that at low energies, an effective (nonrenormalizable) QED vertex is the Pauli moment, $\propto F_{\mu \nu} \bar{\psi}\sigma^{\mu\nu} \psi$, coupling R with R and L with L like the mass term. So the photon couples to these combinations too, at low energy.
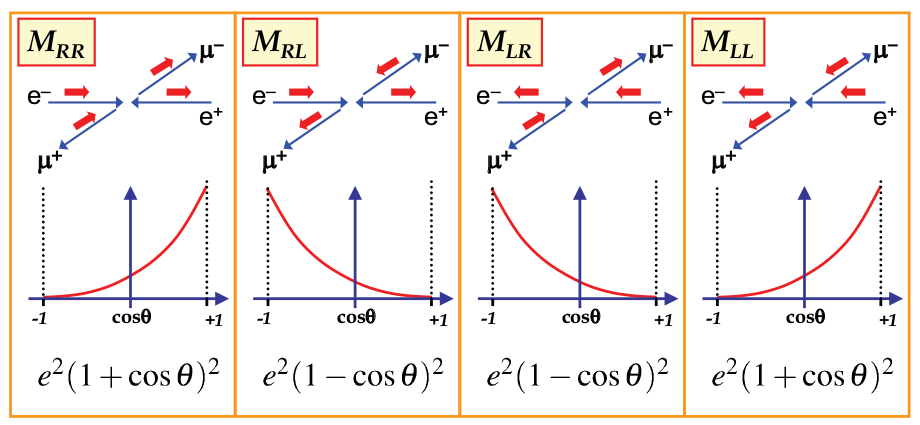
However, at high energies, one may imagine the masses dropping out, in which case there is no way to have these rogue RR and LL couplings--the systematic formal way is to see them suppressed in the amplitudes by powers of m/E. Also in this limit, chirality and helicity are the same, and they are good quantum numbers, preserved in the process. So the 4 amps you list survive, and the 12 rogue ones are suppressed, vanishing in such an idealized massless theory. You need no knowledge of the intermediate state, one or many photons, for that matter, once you know what the initial and final states can be.
It is easy to use the rotation matrices in your helicity amplitudes, $d^1_{1~1}(\theta)$=$(1+\cos \theta)/2$, etc., to rotate from the initial to the final state, square, and get the cross-sections provided--but I understand this is not your problem. Again, thinking about the spin of the photon is counterproductive (it is off shell, and 2 photons would introduce more mootness, etc.), and the moment the amps are restricted in the high-energy limit, you can get the angular distributions by inspection, which is the point here. A full quantum calculation would include everything, including the rogue stuff, with full energy suppression behavior... This is just a quick-and-dirty shortcut, but, at high energies, a good one.