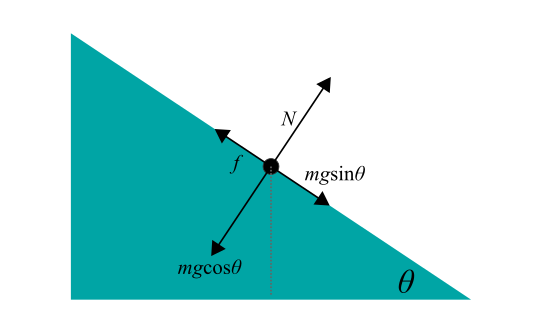
Five choices I made up:
a.
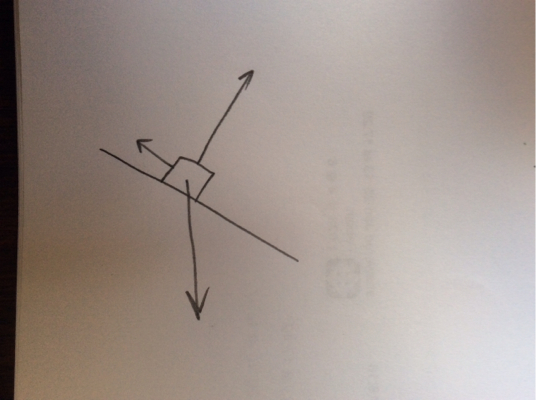
b.
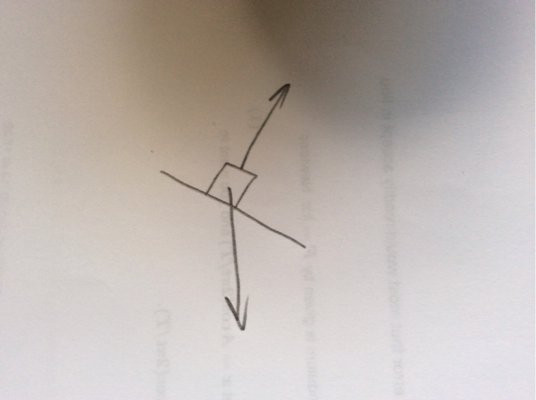
c.
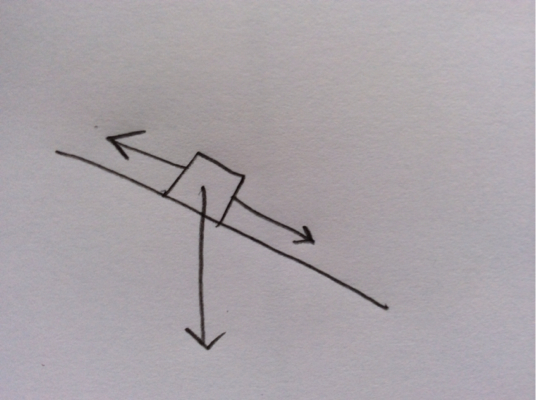
d.
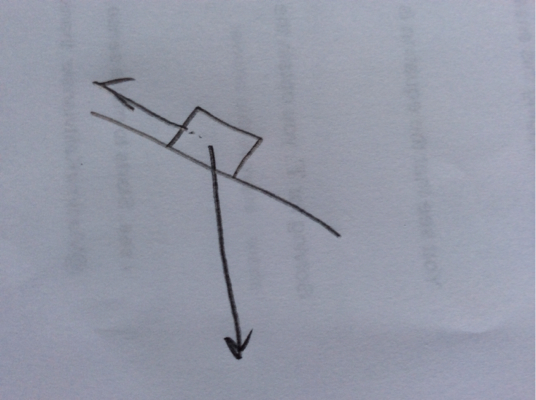
e.
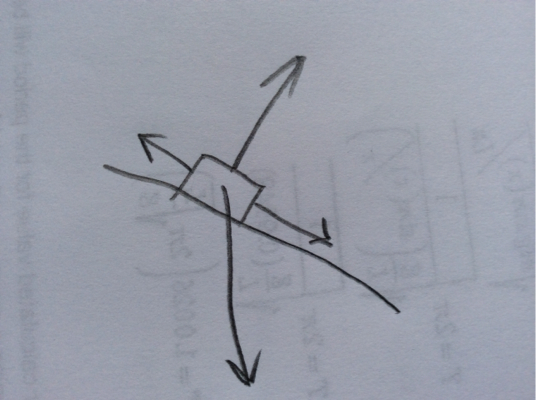
One thing I know immediately is that the vectors have to cancel each other at last so there is no net force acting on it.
a. certainly qualifies.
b. doesn't. If the components of the vertical arrow leave the object sliding down the incline.
c. has two vector forces directed down the incline, but only of which is is cancelled.
d. is tricky.
There is a unbalanced force going into the plane. But that vector won't change the fact that the object is at rest. One would automatically realized there is a normal force preventing the object from falling into the plane.
e is basically the same as c, except that the normal force isn't drawn.
If the cause of my confusion still isn't clear to you, it is that I can't settle between choices a and d, due to the lack of the normal force in d.