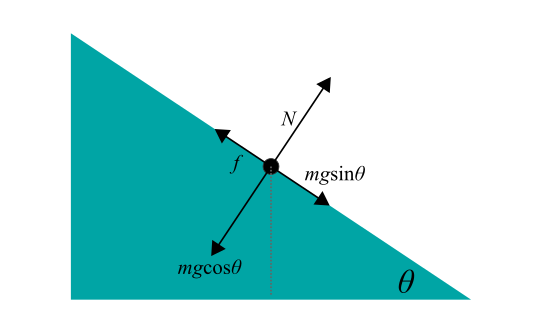
$A$ is correct, assuming the forces are the force of gravity $F_g$, the normal force $N$, and the friction force $f$. $D$ is not ideal because you should draw $N$ in this case.
You might be confusing yourself about $E$ because you are drawing $F_g$ as well as the component of $F_g$ in the direction parallel to the plane ($mg\sin\theta$).
One thing you can do is decompose $F_g$ into components that are parallel and perpendicular to the plane—as long as the component vectors sum to the original $F_g$ vector (theindicated by the dashed line in the following diagram).
Then, since the object is not sliding along the plane or "rising" or "falling through" it, you know that both the sum of the forces parallel to the plane and the sum of the forces perpendicular to the plane must be zero.
If you make this correction to $E$, you have basically found a FBD equivalent to $A$.