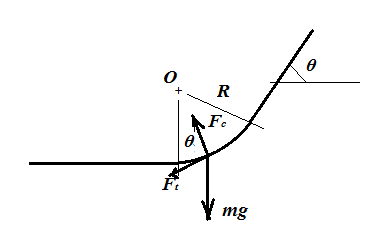
Let's consider this super-simple example. The ball (mass $m$) is moving from position 1 to position 2 at constant horizontal velocity $v$ until the ball reaches the red arrow (in position 1, it has vertical velocity $0$). Gravity force is equal to $mg$.
When the ball reaches the point marked with the red arrow, it starts moving up, therefore its vertical speed is not $0$ anymore.
Its vertical speed has changed = there was vertical acceleration, so there had to be a non-zero net force in the vertical direction that acted on the ball, right? This is exactly what Newton's first law says.
My question is - what force caused the ball to accelerate vertically so it could reach point 2? What source caused that additional force that allowed it to move upward, up the hill?
In position 1, the gravity force acting on the ball is cancelled out by the ground reaction force, so the net force is zero. In position 2, the net force isn't zero anymore, directed 'down' (because gravity force is greater than ground reaction force acting on the ball). So we have two cases - in both the net vertical force is either zero or it is directed down. But it had to be directed up, because the ball moved up!
I know it can be explained with conservation of energy law, but I think there has to be an explanation in terms of forces and momentum only as well (the laws of dynamics are always true).