I read that the derivative of kinetic energy=$F\cdot v$. I tried to differentiate (1/2) mv^2 with respect to time but each time I am getting $m*v$ and not $m*a*v$ which solves to $F*v$. My efforts are as follows:
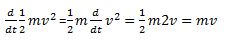
Required URL from where I found it: http://www.feynmanlectures.caltech.edu/I_13.html
Here is the procedure:
$KE = 0.5mv^2$
$\frac{d}{dt}KE = 0.5m\frac{d}{dt}v^2$
So the question becomes,how do we find the derivative of $v^2$ with respect to time?
One can easily see that $\frac{d}{dt} = \frac{dv}{dt}\frac{d}{dv}$ (Notice how the $dv$ cancels top and bottom)
Therefore,
$\frac{d}{dt}v^2 = \frac{dv}{dt}\frac{d}{dv}v^2 = \frac{dv}{dt}\times 2v$
Therefore, $\frac{d}{dt}KE = 0.5\times2mv\frac{dv}{dt} = vm\frac{dv}{dt} = vma = Fv$
Is this the correct way to find the derivative of kinetic energy?
$$ K=\frac{1}{2}m v^2 \\ $$ So: $$ \frac{dK}{dt} = \frac{1}{2} \left(\frac{dm}{dt} v^2 + 2mv \frac{dv}{dt} \right) $$ If the mass does not change over the time, then $$\frac{dm}{dt}=0$$
And finally $$ \frac{dK}{dt} = \frac{1}{2} \left(2mv \frac{dv}{dt} \right) $$
So simplifying: $$ \frac{dK}{dt} = mv \frac{dv}{dt}=mav=F.v $$
The time derivative of $v^2$ is $2v \frac{dv}{dt}$ not $2v$. You must use the chain rule.
