In Ch. 1 Derivation 1 of Goldstein's mechanics, we have
Show that for a single particle with constant mass the equation of motion implies $$ \frac{dT}{dt} = \vec{F}\cdot\vec{v} $$
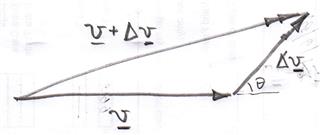
The first step seems straightforward $$ \frac{dT}{dt} = mv\dot{v} $$
But $$ \vec{F}\cdot\vec{v} = mv\dot{v}\cos\theta $$ where $\theta$ is the angle between force and velocity, so the relation that I'm trying to prove only holds if $\vec{F}\,||\,\vec{v}$. I come up against this same parallel requirement in the second part of the derivation trying to show $\frac{d(mT)}{dt} = \vec{F} \cdot \vec{p}$.
In my mind, counterexamples come to mind in the form of circular motion, where $\cos\theta = 0$ or a force against the velocity where $\cos\theta = -1$. Is there something that I'm missing in the problem or derivation here that causes the time derivative of kinetic energy to always be $\vec{F} \cdot \vec{v}$?
Just as a note, there do seem to be quite a few questions about this derivation around that I've found, but none of them seem to address the issue that I'm having.
I just thought of instead doing the derivation using $$ \frac{dT}{dt} = \frac{d}{dt} \frac{m}{2} \vec{v} \cdot \vec{v} = m \dot{\vec{v}} \cdot \vec{v} = \vec{F} \cdot \vec{v} $$ that the result seems to just pop out. I'm not sure of where any meaning about the angle comes out of using $v^2 = \vec{v} \cdot \vec{v}$. It does mean that the direction of $\vec{v}$ doesn't matter, but it isn't clear to me how that affects the dot product $\vec{F} \cdot \vec{v}$.
Out of curiosity, I also came up with the following that I think should also be valid starting with the definition of work: $$ dW = \vec{F} \cdot d\vec{l} = \vec{F} \cdot \vec{v} dt \implies \frac{dW}{dt} = \vec{F} \cdot \vec{v} $$ and by work-energy, $\frac{dW}{dt} = \frac{dT}{dt}$ (throwing away the delta since we're looking at a time derivative), so $\frac{dT}{dt} = \vec{F} \cdot \vec{v}$.
In addition to the accepted answer, I'll also point out that the correct calculation of $\frac{dv}{dt}$ seems to be $\frac{\vec{v} \cdot \dot{\vec{v}}}{v}$, which changes $\frac{dT}{dt}$ to the expected result.