10
$\begingroup$

This can be considered a follow-up turn-around question for this similar question.

I recently came across this interesting analysis of the game of tug of war.
One of the closing discussions is the following image:

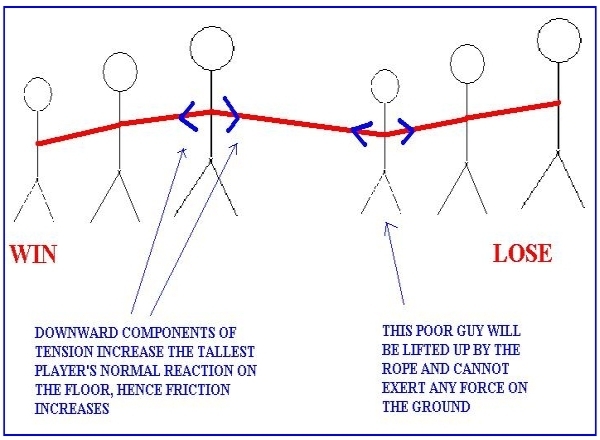
Sample image

However, the writer follows up that:

[Given there are multiple people on both teams,] the drawing above shows that the shorter players on the left team also experience an upward component of force, and therefore the friction on their feet is reduced. The shorter player on the right team certainly experiences decreased friction, but the other players on the right team have increased friction at their feet.

How do all the forces add up? Is it beneficial to put taller people first in a game of tug of war as shown in the diagram above, or do the forces cancel each other out?

Improve this question
$\endgroup$
2
  • $\begingroup$ Note: if you play the game properly (i.e. with a muddy pond between the teams) then the drawing is not to scale. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Jan 29, 2015 at 0:28
  • 2
    $\begingroup$ The participants don't stand up straight but instead lean at quite a shallow angle. By varying the angle of lean the players of different heights can keep the rope level. So I don't think the analysis you cite is correct. The requirement to vary the angle of lean to compensate for height differences may well have some effect, but offhand I'm not sure what that would be. $\endgroup$
    – John Rennie
    Commented Jan 29, 2015 at 9:58

2 Answers 2

Reset to default
2
$\begingroup$

For calculating the total force a team can generate, it is useful to consider one team as a single system. Note that the total normal force of a team acting on ground only depends on the total mass of the team and the vertical component of tension force of the rope between the teams. You can't do much to increase the team's mass, however you indeed can generate downforce (and upforce for the other team) by putting the tallest closest to the other team (perhaps also the tallest could try to lift the rope even higher, though I'm not sure it will help in practice. The friction force generated by real people is not purely proportional to the normal force).

Improve this answer
$\endgroup$
0
$\begingroup$

The drawing has it completely wrong, because it doesn't take into account the leaning that players do. Leaning is beneficial to the team, because it allows you to use gravitational force as well as your muscles to pull the rope and removes the height differences in the rope that would put shorter players at a greater disadvantage than they already have (taller players lean more, shorter ones lean less). Since taller people need a lot of space behind them to lean properly, it is beneficial to the team to have tall people in the back and short people in the front.

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ I doubt this approach is correct. There's a limit to how much you can lean before you slip so all in all taller people will still grip the rope at a greater height, even if at a smaller difference. As for spacing, you can space players out in any order so that point doesn't seem to make sense. $\endgroup$
    – Etheryte
    Commented Jul 1, 2015 at 16:28
  • $\begingroup$ @Nit the length of the rope limits the spacing you can use $\endgroup$
    – swapneils
    Commented Jul 1, 2015 at 16:42
  • 1
    $\begingroup$ In settings I've seen tug of war take place (I'm sure there are numerous different rule sets around the world), you're usually not that short on rope. $\endgroup$
    – Etheryte
    Commented Jul 1, 2015 at 16:44

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.