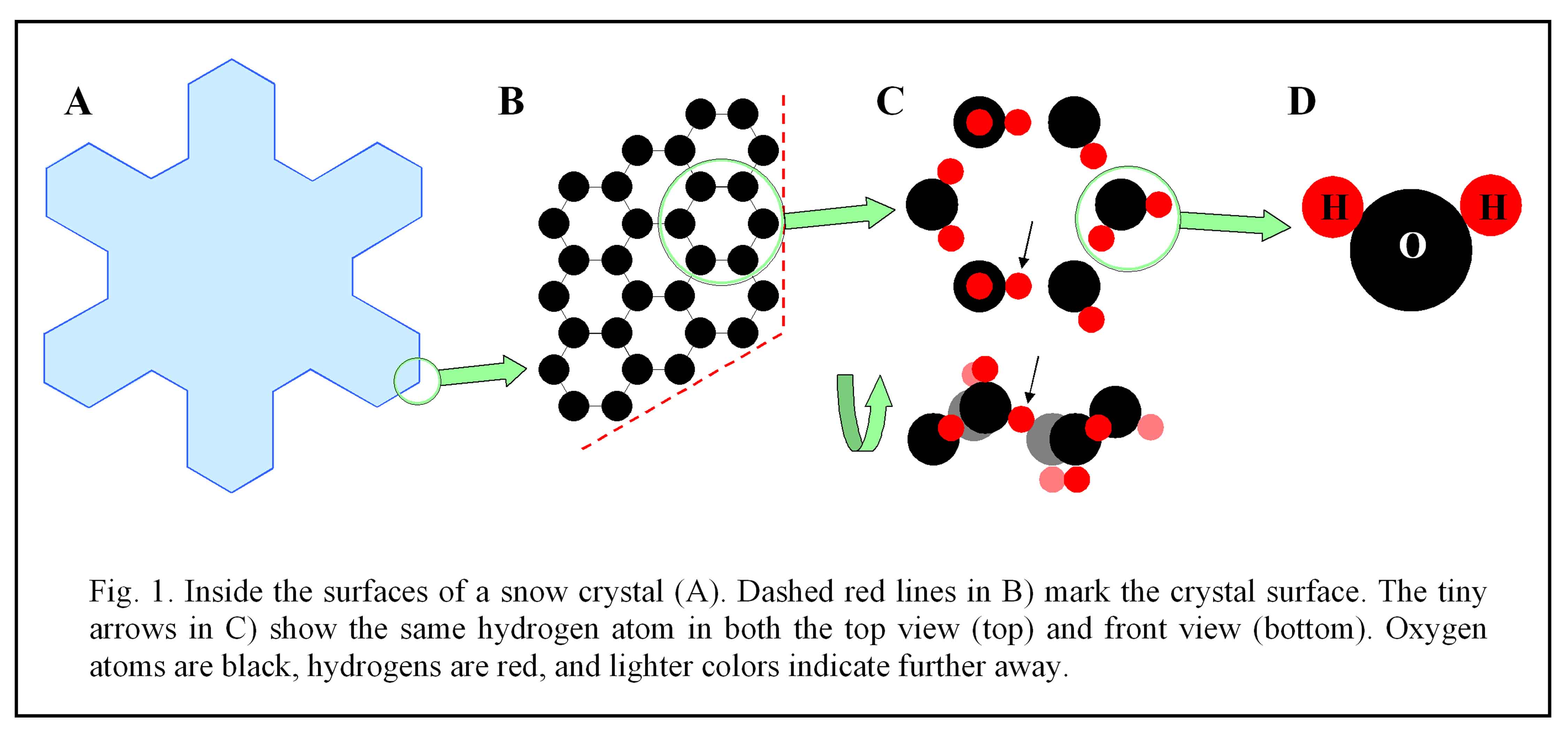
I want to know why snowflakes (and other crystals) grow symmetrically and I find the leading answer to the established question to be entirely unsatisfactory.
When water freezes, you get ice. Ice, like many solid materials, forms a crystalline structure. In the case of water, the crystalline structure may be attributed to the hydrogen bond, a special kind of an attractive interaction.
So a big chunk of ice will have a crystalline structure - preferred directions, translational symmetry, and some rotational symmetries.
Nature adds one water molecule at a time. The molecules always try to choose the most energetically favored position on the frozen body. Because these laws of creation of a snowflake are symmetric with respect to the rotational symmetries, it follows that any symmetry that exists at the beginning - a hexagonal symmetry of a small number of molecules in the initial crystal - will be preserved.
No, sorry, it doesn't follow at all. Something hugely important is missing from this answer -- causality.
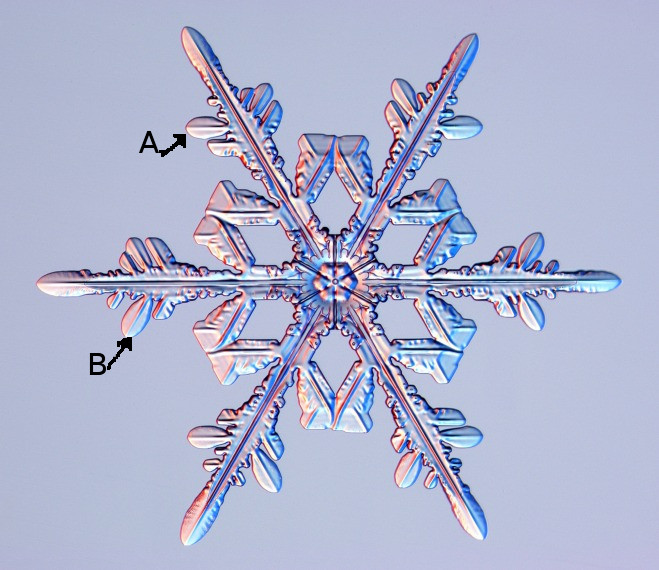
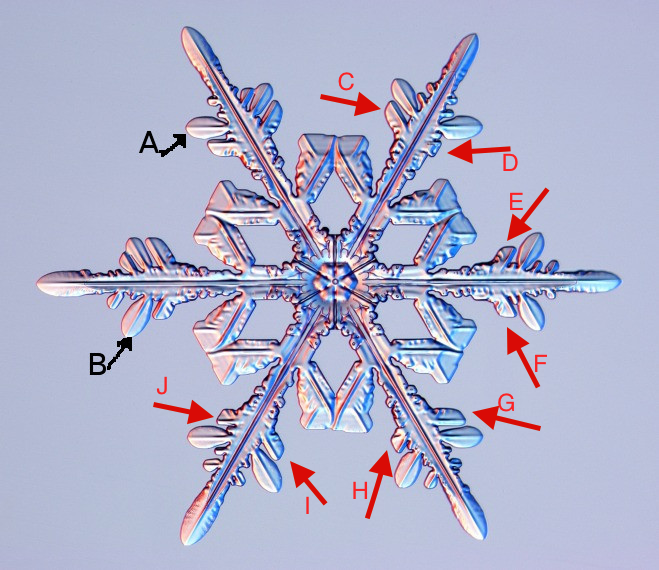
Suppose the snowflake at one moment is perfectly symmetrical. Then suppose a random molecule gets added at point A. To maintain the symmetry, later on a random molecule gets added at a corresponding point B (and four others). But the motion of the water molecule is random and there is no reason for it to end up at B or anywhere else in particular.
Even considering theo's remarks about dendrite growth, why don't we see this?
I see absolutely no reason why any given additional water molecule cannot land in an energetically unfavourable position. Besides, if low energy considerations were a driving force, snowflakes would be approximately spherical.
So, what's really going on? How can a bump at A create an attractive force at a distant B?