But we do see exactly the kinds of asymmetries that you're asking about, even in your sample image. Here it is again, with some more letters and arrows:

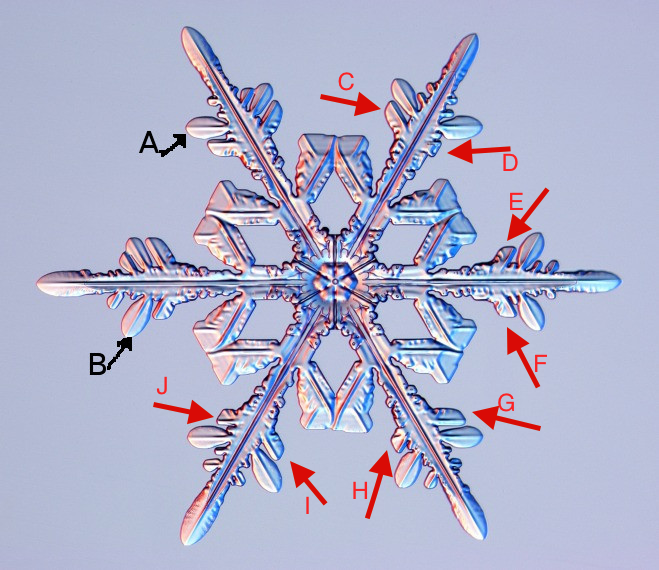
If the ice crystals are more or less confined to a plane, you expect the crystal to be symmetric under rotations of 60º (one-sixth of a turn) and reflections about six axes in the plane. That's clearly approximately true for this snowflake. But look at the second biggish lobe on each long arm.

• A reflection with lower-left to upper-right arm as its axis would swap $C\leftrightarrow D$ and $I \leftrightarrow J$, but those lobes are different sizes.

• A reflection about the orthogonal axis would swap $C\leftrightarrow J$ and $D\leftrightarrow I$, but those lobes are different sizes.

• A rotation by 60º clockwise would take $B\to A$, which as you point out are the same size, but would also take $(C,D)\to(E,F)$, which don't match.

If I had better image manipulation tools and a lot of free time I would overlay rotated and reflected images on each other to make the differences more obvious, but I think you should have the point. The asymmetries are there, but they are smaller than you expected.

Predicting the size of fluctuations in stochastic processes is hard, but fruitful; the most famous example is Einstein's analysis of fluctuations in Brownian motion, which was the deciding evidence in favor of the theory that matter is made of atoms.

But we do see exactly the kinds of asymmetries that you're asking about, even in your sample image. Here it is again, with some more letters and arrows:

If the ice crystals are more or less confined to a plane, you expect the crystal to be symmetric under rotations of 60º (one-sixth of a turn) and reflections about six axes in the plane. That's clearly approximately true for this snowflake. But look at the second biggish lobe on each long arm.

• A reflection with lower-left to upper-right arm as its axis would swap $C\leftrightarrow D$ and $I \leftrightarrow J$, but those lobes are different sizes.

• A reflection about the orthogonal axis would swap $C\leftrightarrow J$ and $D\leftrightarrow I$, but those lobes are different sizes.

• A rotation by 60º clockwise would take $B\to A$, which as you point out are the same size, but would also take $(C,D)\to(E,F)$, which don't match.

If I had better image manipulation tools and a lot of free time I would overlay rotated and reflected images on each other to make the differences more obvious, but I think you should have the point. The asymmetries are there, but they are smaller than you expected. And there's a strong bias towards publishing photographs of exceptionally symmetrical flakes like this one. Libbrecht, who may have taken that image, puts it nicely:

If you think this is hard to swallow, let me assure you that the vast majority of snow crystals are not very symmetrical. Don't be fooled by the pictures -- irregular crystals (see the Guide to Snowflakes) are by far the most common type. If you don't believe me, just take a look for yourself next time it snows. Near-perfect, symmetrical snow crystals are fun to look at, but they are not common.

Elsewhere:

Even on the best of days, I search for hours to find just a few beautifully symmetrical specimens. I typically glance over thousands of crystals on my collection board before selecting one to photograph, and the pictures you see in the Galleries are some of the best among over 7000 pictures I've taken.

That's been my personal experience looking at actual snowflakes as well.