0
$\begingroup$

An electron-positron annihilation can produce a pair of gamma rays.

In the reverse process, known as pair production,

  1. can the gamma rays carry enough information to determine the resulting particles, e.g. which one will be electron and which one positron,

  2. if so, then exactly how, and

  3. does an electron-positron annhilation produce gamma rays with such information.

Improve this question
$\endgroup$
5
  • 2
    $\begingroup$ What you mean by "which one" will be electron? Please explain. $\endgroup$
    – Sofia
    Commented Nov 24, 2014 at 0:10
  • $\begingroup$ @sofia: two particles are produced in a pair production. they fly off in opposite directions. when one of these is an electron, the other is a positron. if the gamma rays do not contain enough information to determine the general directions, then that is an answer (namely a "no", not enough info for exact reversion of annihilation). but if they do, then we have a pair of particles going off in known directions, and then, is it determined or arbitrary which is electron and which is positron. if arbitrary, then again there is an answer of "no", annihilation not reversible. $\endgroup$
    – Cheers and hth. - Alf
    Commented Nov 24, 2014 at 0:19
  • 2
    $\begingroup$ Don't forget about Heisenberg uncertainty principle. $\endgroup$
    – Ruslan
    Commented Nov 24, 2014 at 10:31
  • $\begingroup$ Do you believe that photons born by electron - positron annihilation contain info about the electron and positron wavefunctions ? $\endgroup$
    – kludg
    Commented Sep 22, 2022 at 11:38
  • $\begingroup$ @kludg: No, I don't. And I don't think anyone else do either. And if we're right about that, then pair annihilation is an example of a simple physical process that destroys information. Contrary to the belief that no physical process destroys information, which is central to e.g. the "black hole information paradox". So admitting this would open up a Pandora's box. E.g. consider that every photon interaction may destroy information, and how that can impact on the notion of time, etc. I believe it will not be admitted until a sufficient number of generations have passed by. $\endgroup$
    – Cheers and hth. - Alf
    Commented Sep 23, 2022 at 15:42

2 Answers 2

Reset to default
2
$\begingroup$

Once the gammas are produced, they do not carry particular information. There is just a certain probability, measured by a differential cross section, that, if they scatter again, they produce $e^+,\,e^-$ as in the initial pair.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thanks! I'm still wondering, are the gamma rays emitted in any particular direction relative to the original particle pair? And -- does this follow from the rules of the standard model, or is it plain observed facts not accounted for by the model? $\endgroup$
    – Cheers and hth. - Alf
    Commented Nov 25, 2014 at 3:19
  • $\begingroup$ @Cheers and hth: I don't know the standard model that you mention, but I can say that gamma rays are very energetic. So, I suppose the gamma photon can be described with a definite linear momentum. The laws of conservation (including total spin projection if the photon is polarized, because the photon has angular momentum +-ħ) should define in which directions with respect to the gamma linear momentum, are emitted the e^- and the e^+. So, it's not the photon that has memory, but the conservation laws. The only thing I am not sure, which conservation law is not symmetrical in e^- vs. e^+. $\endgroup$
    – Sofia
    Commented Nov 25, 2014 at 14:45
  • 1
    $\begingroup$ @Sofia: Thank you. The "standard model" I referred to is the Standard Model of particle physics, the prevalent theory (or theories) since the mid 1970's. Re conservation of momentum, I understand what that means for ordinary momentum, where in the rest frame of collision it's zero and allows any directions. But I don't understand your comment about angular momentum. As I understand it we're talking about at least or exactly two photons, since conservation of ordinary momentum in the rest frame of collision requires that. Is that wrong? $\endgroup$
    – Cheers and hth. - Alf
    Commented Nov 25, 2014 at 19:56
1
$\begingroup$

In particle physics we use Feynman diagrams to calculate the probabilities of interaction of two partilces. This is a Feynman diagram of electron positron annihilation , dominant for energies low enough so that more particles cannot appear. At high energies a lot more particles come out than two gammas.

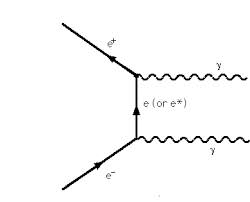
e+e-annihilation

Now the probabilities of interaction come from the prescription for Feynman diagrams and two photons are indistinguishable in this calculation. They do not have "memory" of the initial two particles other than the angular distribution, a probability plot, computable from the diagram. The diagram can be read and is valid for calculations in reverse time.

I cannot understand what you mean by reversible. If you mean can two photons scatter off each other and produce an electron positron pair, yes, one could compute the probability amplitude for that. Usually this happens often in detectors with the second gamma a virtual one from the field of an atom or a nucleus.

If you mean the two original gammas of e+e- annihilation, they will never meet again as they depart with the velocity of light, and the probability of meeting another one that has come from an e+e- annihilation is very very small unless it is two gamma beams designed to see this ( but why would one want to do that) . They are talking of gamma gamma colliders, but at much larger energies to generate a lot of particles.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thanks for answer! Re what I meant by "reversible" in the commentary, I used that as a short form to refer to "enough info for exact reversion" earlier in that sentence. Any process is reversible in the sense that we can imagine viewing it as a movie in reverse, but that "any" also means that that sense is meaningless. For classical (non-quantum) physics reversible is simply that a reversal of all velocities would play out the original system evolution in reverse. With probabilistic behavior it's less clear, but total information loss about some aspect necessarily means "not reversible". $\endgroup$
    – Cheers and hth. - Alf
    Commented Dec 28, 2014 at 15:26

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.