0
$\begingroup$

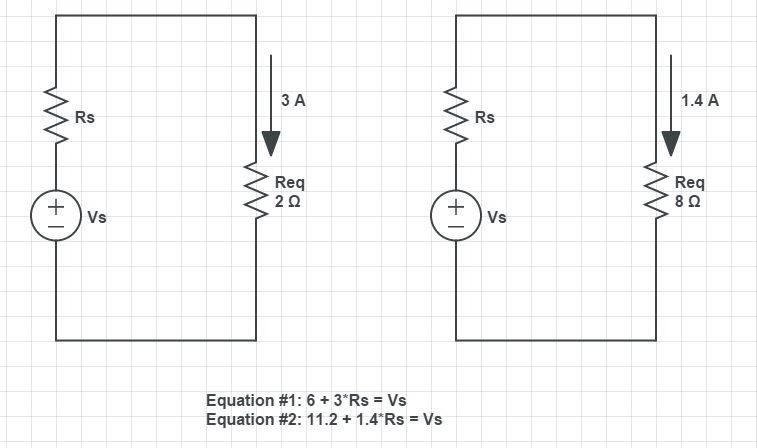
When two resistors, each of resistance 4.0 ohms, are connected in parallel with a battery, the current leaving the battery is 3.0 A. When the same two resistors are connected in series with the battery, the total current in the circuit is 1.4 A. Calculate the internal resistance of the battery. The solution started with

$1.4 = \frac{E}{8.0 + r}$ and $3.0= \frac{E}{2.0+r}$

I understand the first part because the current in series is always the same, and they are considering the total resistance. But for the second part, I'm confused on why 3 A is used. It's 3 A when it leaves the battery, but didn't it already go through the internal resistor so it's not an accurate representation of the total current in the parallel circuit?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
2
$\begingroup$

The 3 A that leaves the battery depends on two things: the amount of supply voltage, and the impedance (here resistance) that draws this current.

So the second part simply says:

  • The 3 A current that leaves the voltage source = The voltage source divided by the total resistance (which is the internal resistance, plus the equivalent of the parallel combination.)

Here is a schematic of the situation with the governing equations:

enter image description here

Improve this answer
$\endgroup$
1
  • $\begingroup$ Pretty close. Just a small clarification: the 3A goes entirely through the internal resistance of the voltage source, and is split 50-50 between the two parallel resistors. $\endgroup$
    – Floris
    Commented Sep 14, 2014 at 1:05
0
$\begingroup$

Since the internal resistance of a voltage source is modelled as a resistor in series with source, all of the source current is through the internal resistance.

If the open circuit voltage of the battery is denoted by $V_{OC}$ and the internal resistance is denoted by $R_S$, the voltage across the battery terminals is given by

$$V_{BAT} = V_{OC} - R_SI_S$$

Where $I_S$ is the current 'leaving' the battery. The current through the external resistance $R_L$ is, by Ohm's law

$$I_S = \frac{V_{BAT}}{R_L} = \frac{V_{OC} - R_SI_S}{R_L}$$

Thus,

$$I_S = \frac{V_{OC}}{R_L + R_S}$$

where $R_L$ is the equivalent resistance connected across the battery.

In other words, whether the external resistors are series connected, parallel connected, or combination series parallel connected is irrelevant. These resistors can always be combined ('lumped') into one equivalent resistor with resistance $R_L$.

Since you have two unknowns for this problem, $V_{OC}$ and $R_S$, you need two independent equations in order to solve for $R_S$.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.