4
$\begingroup$

Assume a hypothetical 3 media/ 1 layer structure with the following indices of refraction: $$n_1 = 2, n_2 = 2+i0.5, n_3 = 1$$ where the thickness of the layer is 100 nm and wavelength = 1000 nm.

Using Fresnel equations to derive the total transmittance and reflectance I get that about ~38% of incident power is absorbed in the layer.

If we now add another boundary, so that the structure now looks like: $$n_1 = 2, n_2 = 2+i0.5, n_3 = 1, n_4 = 3$$

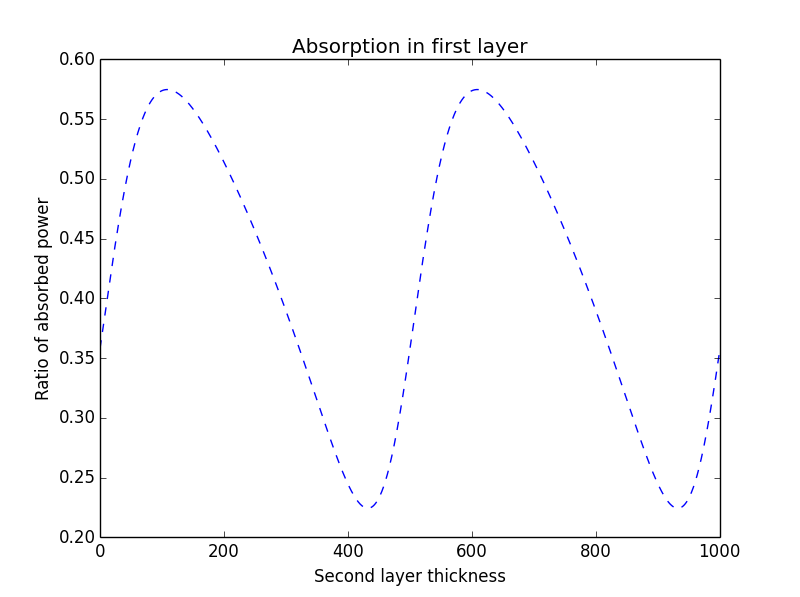
The absorption in the first layer as a function of the second layer thickness now looks like: enter image description here

My question is how is that the absorption now goes below the ~38%?

I understand that when deriving the transmission and reflectance I am summing up the electric field amplitude, some of which might cancel due to the reflections in the second layer but intuitively this still doesn't make sense.

Improve this question
$\endgroup$
8
  • 1
    $\begingroup$ I'm just venturing a guess here, but because your films are quite thin, could this be a thin-film interference phenomenon? $\endgroup$
    – Xcheckr
    Commented Aug 11, 2014 at 4:42
  • $\begingroup$ @Xcheckr yes it is $\endgroup$
    – user1830663
    Commented Aug 11, 2014 at 4:44
  • $\begingroup$ Looks like there's more reflection w/ the fourth layer, thus leading to a "second chance" for absorption. Perhaps you can trace the transmission and reflection at each interface to see what's actually happening? $\endgroup$
    – Carl Witthoft
    Commented Aug 11, 2014 at 13:44
  • $\begingroup$ @CarlWitthoft I get how there can be more absorption. Whats confusing is how can there be less than 38% $\endgroup$
    – user1830663
    Commented Aug 11, 2014 at 13:53
  • $\begingroup$ Oops - maybe the reverse of my previous comment: less reflection, leading to less reflected light to be absorbed? $\endgroup$
    – Carl Witthoft
    Commented Aug 11, 2014 at 14:25

1 Answer 1

Reset to default
1
$\begingroup$

I assume the light arrives from the n1 layer.

The second thin layer (n3=1) is sandwiched between two layers of greater refractive index, so it acts like a Fabry-Perot interferometer (etalon). As you change its thickness, it alternates between high transmission and high reflection. In its transmitting state, there is very little reflection back into the n2 layer, and so not much absorption. When the n4 material is absent, there is strong reflection at the n2/n3 boundary, putting light back into n2 to be absorbed a second time. When n4 is present, the reflection at n3/n4 can interfere destructively with that from n2/n3, reducing the energy that goes back into the absorbing n2 layer. I suggest that you study the simpler case of two interfaces - which is mathematically easy but still gives surprising results.

The problem in the question is sufficiently complicated that you don't get the right answer by just plugging in the conditions for a simple two-surface Fabry-Perot etalon (lambda/4) - you need a detailed calculation as the the questioner has done.

Improve this answer
$\endgroup$
2
  • $\begingroup$ In terms of electric field amplitude, the total reflection from the first 2 boundaries(n1/n2 and n2/n3) is the same regardless of what comes after, so I dont understand the "strong reflection of n2/n3". Also what do you mean by your last sentence? I am quite sure that my derivation is correct. It is equivalent to the transfer matrix method and matches several numerical packages $\endgroup$
    – user1830663
    Commented Aug 11, 2014 at 22:32
  • $\begingroup$ Ok. The destructive interference from the n3/n4 is what I was thinking. I guess my confusion came from thinking that all the reflection from n2/n3 happens before it has a chance of interfering with n3/n4. $\endgroup$
    – user1830663
    Commented Aug 15, 2014 at 0:32

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.