Compressibility of solids (quadratic approximation)

Question

(according to Steven H. Simon's "The Oxford Solid State Basics")

A well-known approach to describe a one-dimensional chain of atoms is to approximate the potential of each of the atoms quadratically:

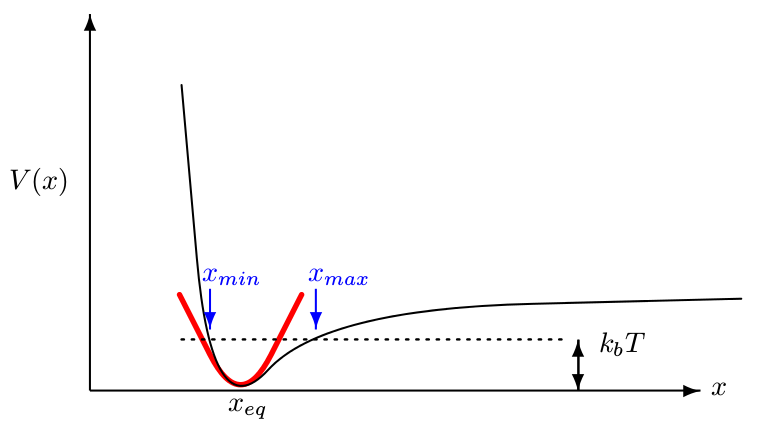
Therefore, $V(x) \simeq V(x_{eq}) + \frac{\kappa}{2}(x-x_{eq})^2$ and hence $F=-\kappa (x-x_{eq})$.

We can use this approximation to describe compressibility whose coefficient is defined as: $$\beta = -\frac{1}{V} \left (\frac{\partial V}{\partial P} \right )_T$$

In 1D this becomes (I'll skip the T subscript): $$\beta = -\frac{1}{L}\frac{\partial L}{\partial F}=\frac{1}{\kappa x_{eq}}$$ I don't understand how this result was obtained. Isn't $\frac{\partial F}{\partial x}$ simply equal to $-\kappa$? What exactly are we differentiating with respect to? Also, am I right in thinking that the $P \rightarrow F$ transition may be justified because pressure "becomes" force in 1D?

Answer 1

Since $F=\kappa(x-x_\text{eq})$, distance as a function of force becomes $$x(F)=x_\text{eq}-\frac{F}{\kappa}.$$ With $N$ atoms, the length $L$ is $$L(F)=Nx(F)$$ giving $$\beta(F)=-\frac{1}{L}\frac{\partial L}{\partial F}=\frac{1}{\kappa x_{\text{eq}}-F}\approx\frac{1}{\kappa x_\text{eq}}$$ whenever $F\ll\kappa x_\text{eq}$ (ie, in the small-distortion limit).

Since the model breaks down in the large-distortion limit anyways due to anharmonicity, this is a valid approximation.