5
$\begingroup$

It's not homework (I'm teacher). I would like to compute sum of forces on this study :

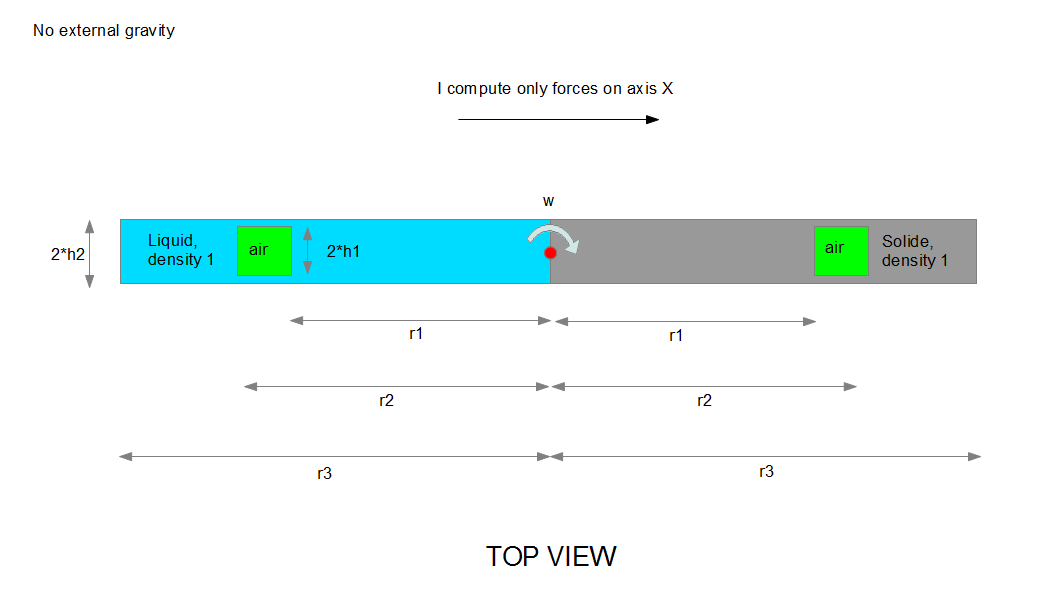
enter image description here

The shape is symmetrical like that I'm sure the center of gravity is in the center of the shape. I compute forces on axis $X$ only. No external gravity. Air container are fixed on big container. But air container has very low pressure, I don't want to compute it, consider it like vacuum.

I know force of pressure is like :

$\frac{1}{2}{\rho}h_{\epsilon}^2w^2R^2$ with R the distance to container from center of gravity of container, $h_{\epsilon}^2$ is a small area, true ?

I compute forces on water, depth is d, it's:

$$d\omega^2\rho*((\int_{0}^{h1}(r1^2+y^2)cos(atan(\frac{y}{r1}))dy)-(\int_{0}^{h1}(r2^2+y^2)cos(atan(\frac{y}{r2}))dy)+(\int_{0}^{h2}(r3^2+y^2)cos(atan(\frac{y}{r3}))dy))$$

so, it's:

$$d\omega^2\rho*((\int_{0}^{h1}\sqrt{(r1^2+y^2)}r1dy)-(\int_{0}^{h1}\sqrt{(r2^2+y^2)}r2dy)+(\int_{0}^{h2}\sqrt{(r3^2+y^2)}r3dy))$$

Is it ok ?

Now, if I want to compute forces on rectangle solid, I need to integrate 2 times ? Like that :

$$\frac{1}{2}d\rho\omega^2*(\int_{-h2}^{h2}\int_{0}^{r3}(x^2+y^2)^{0.5}cos(atan(\frac{y}{x}))dxdy-(\int_{-h1}^{h1}\int_{r1}^{r2}(x^2+y^2)^{0.5}cos(atan(\frac{y}{x}))dxdy)$$

So it's:

$$\frac{1}{2}d\rho\omega^2*(\int_{-h2}^{h2}\int_{0}^{r3}(x^2+y^2)^{0.25}xdxdy-\int_{-h1}^{h1}\int_{r1}^{r2}(x^2+y^2)^{0.25}xdxdy)$$

How to add mass inside integrales ?

I have a problem, I don't find $0$ for the sum of forces.

There are 2 additional forces $F_a$ that decrease forces from liquid:

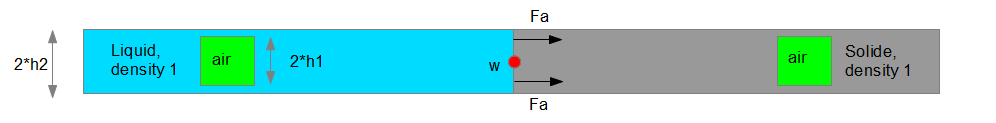
enter image description here

But the shape can be like that:

enter image description here

So in this case forces $F_a = 0$. In last case, $F_a$ are compensated by what forces ?

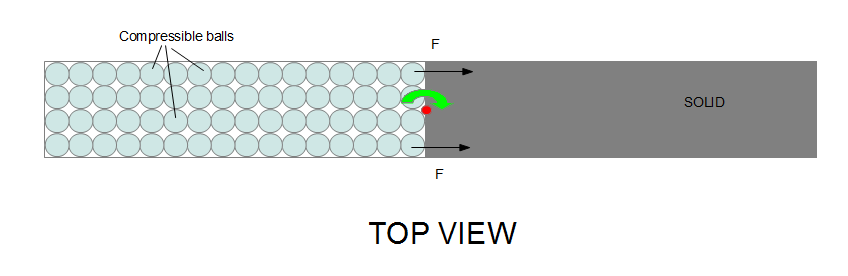
If I take a macroscopic model, with compressible balls for understand where F forces are compensated, consider the center of gravity like red point (balls have more density than solid, just enough for compensated):

enter image description here

And there is another force to take in account it's the red force :

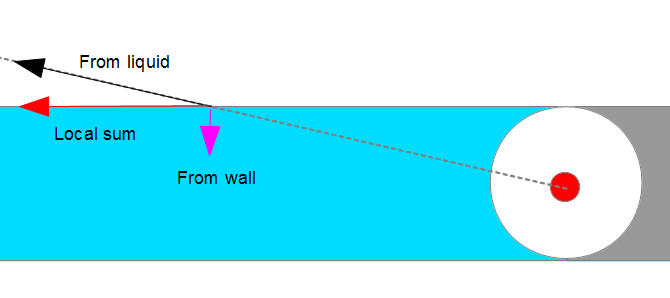
enter image description here

This red force is apply all along the surface of container ?

Improve this question
$\endgroup$
0

1 Answer 1

Reset to default
1
$\begingroup$

The question is confusing for several reasons.

First, it doesn't specify if the air is fixed in place somehow, or free to move. The air container would experience a force in the direction of the axis of rotation the magnitude of which would depend upon the density of the liquid, the density of the air, the rate or rotation, and distance of each point of the container from the axis.

Second, it introduces a variable or constant "k" without any explanation.

Third, it's not clear what force is being considered. If the water is not moving toward or away from the axis, there is no net force on the water. There will be a pressure at each point in the water that depends upon distance from the axis. The pressure of the water exerts a varying force on each infinitesimal area of the water container, and the container exerts an equal and opposite force.

The pressure at any point in the water (assume in air is in a fixed container) would be $\rho \omega ^2 r^2/2$, where "r" is distance from the axis of rotation.

See the following reference for more information: http://science.utcc.ac.th/lecturer/muanmai/AITdownload/Ch6_centrifuge.ppt

Improve this answer
$\endgroup$
14
  • $\begingroup$ Sorry. Forget k please. Air container is fixed. I changed formulas. I consider the sum of forces on axis X (no external gravity) for all the study: water+containers. Sure I need to find 0. I consider air like vacuum. Thanks for the link :) $\endgroup$
    – user43880
    Commented Apr 21, 2014 at 12:54
  • $\begingroup$ can't you use dx single integrals, consider the force in the x direction of each infinitesimal cross section. Have three intergals, one from 0 to x1, one from x1 to x2, and one from x2 to x3? $\endgroup$
    – DavePhD
    Commented Apr 21, 2014 at 13:28
  • $\begingroup$ For the right side (mass) ? I don't know how add mass inside integrals. I thought it was double integrals because it's a surface. If I use single integral how can I take mass in parameter ? $\endgroup$
    – user43880
    Commented Apr 21, 2014 at 13:33
  • $\begingroup$ $dM=\rho Adx$ where $dM$ is the mass of a cross section and $A$ is the area of the cross section. You haven't told us a 3-dimension shape for the container, so we can not say what $A$ is in terms of the variables provided. $\endgroup$
    – DavePhD
    Commented Apr 21, 2014 at 13:40
  • $\begingroup$ With d=depth, I can't write double integrales like I wrote (I changed formulas) ? I can't understand how I can integrate all forces on the solid only with one integrale. $\endgroup$
    – user43880
    Commented Apr 21, 2014 at 13:56

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.