Reading about electronic band structures, I came across the following:
Band gaps open at the edges of the Brillouin zone (BZ), since that is where the Bragg scattering occurs.
I am slightly confused, as most band structures show a gap opening at $\Gamma$ which is at the centre of the BZ. What am I missing?
They are shown at the $\Gamma$ point in special diagrams called the reduced zone scheme in which a band will be shown folded back on itself. This way of showing the band structure is convenient for a few reasons, one of which is that it saves space on the page. If you look at that band gap at $\Gamma$ and follow the lower band down to lower energies, you will find that at some other symmetry point the band will change direction and then continue down to the zero-energy state at the $\Gamma$ point.
There's a another scheme, the extended zone scheme, in which the band gap will appear more explicitly to be at a zone boundary. These diagrams take up space, and it's also difficult to tell which states share a single wave vector. In the reduced zone scheme, all states that line up vertically have the same wave vector.
Both schemes show the same information.
Details: The $\Gamma$ point has wave vector $k=0$. The edge of the 1st BZ occurs when $k=\pi/a$ where $a$ is the lattice period. The edge of the second BZ occurs at $k=2\pi /a$. But that means that the wave length of that state is equal to the lattice spacing. So for example if a wave peak occurs at a lattice site, every wave peak will occur at a lattice site. The wavefunction has the same value at every single lattice site. This is exactly the same thing that happens at when $k=0$ and the wavelength is infinite.
That is to say that the wave vector $k=2\pi / a$ is identical to the wave vector $k=0$. The second zone boundary occurs at $k=2\pi/a$ which is the same as saying that the second zone boundary occurs at $k=0$. The second zone boundary does indeed occur at the $\Gamma$ point. This seemingly peculiar state of affairs is a consequence of the mechanics of a periodic potential, such as we have in a perfect crystal.
This periodic unravelling of wave vectors is what's exploited in the reduced zone scheme.
Consider the illustration showing a nearly free electron in a 1-d periodic potential. Two views of the same dispersion relation: first in the extended zone scheme, then in the reduced zone scheme. The second zone is folded back onto the first, so that the 2-3 zone boundary occurs at $k=2\pi / a$ in the extended zone scheme, and $k=0$ in the reduced zone scheme. But in a periodic potential, $k=2\pi /a$ and $k=0$ are the same wave vector. Both diagrams contain exactly the same information.
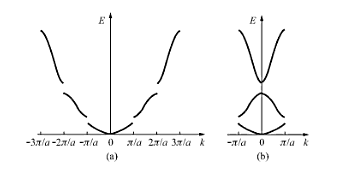
Figure credit: Introduction to Condensed Matter Physics, Vol I, Feng and Jin, World Scientific 2005
