when we use the nearly free electron approximations for electrons in a solid and get them as plane waves the energy becomes $E=\frac{\hbar^2k^2}{2m}$, which gives us a parabola. but when we see the band structure obtained from this model, there are several energies for one $k$.
what does that mean? how can we justify this as there is nothing in the $E(k)$ equation (parabola)?
I changed the photo and explained the question on it...
Your picture is correct, you have many different energies for electrons with the same quantum number $k$ (the crystal momentum). The reason for this behavior is the following: unlike the free electron case, electrons in a lattice require an additional quantum number to specify the state of an electron. This additional quantum number is the band index $n$. This band index is just a label for the periodic part of the Bloch wavefunction $u_n(x)$ of $\psi(x)=u_n(x)e^{ikx}$, where $u_n(x)$ is a function with the same periodicity as the lattice.
For example, one can sometimes label the bands by the atom/orbital that gives rise to it (e.g. Fe-$3d$ or Li-$2s$).
We can also understand this situation by appealing to an analogy with the Hydrogen atom. Think about the energy spectrum of Hydrogen as a function of angular momentum $l$. There, you have infinitely many states each with different energies for a single value of angular momentum $l$. But, is this actually a problem? No, it just means that we need more quantum numbers to specify a particular state in addition to $l$. In the Hydrogen case, what we need to consider is the principle quantum number $n$ which dictates the radial part of the wavefunction.
Most of the confusion this chart (v1) generates comes from the choice of the $x$-axis, which is puzzled togeter from lines connecting different high symmetry points in the (first) Brillouin zone.
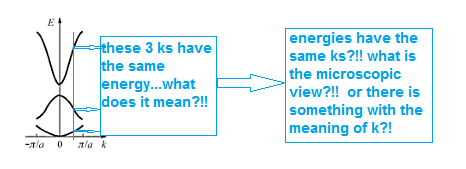
The free dispersion parabola is "folded" back into the first Brillioun zone (as points separated by reciprocal lattice vectors can be identified – the crystal momentum is only conserved up to inverse lattice vectors). This is especially easy to understand in the 1d case. (Just draw a parabola, the first Brillouin zone and shift back the parts of the parabola outside of the first Brillouin zone).
The low slope parts in the higher bands are due to the fact, that there the shifted back parabola is not necessarily shifted in such a way, that the lines chosen follow the direction of the highest slope.
In a real metal the first thing that happens is that the degeneracy at the high symmetry points is lifted (due to the lattice periodic potential due to the ions).
The new image does not correspond to $E = \hbar^2k^2/2m$ (but to the situation with non-vanishing a periodic potential) and does therefore not properly illustrate the question. But still, the point is, that crystals break translation symmetry to a discrete group of lattice translations! Therefore, in an infinite crystal the conserved quantity is not momentum (which is a quantity conserved due to the continuous translation invariance of a theory) but quasi-momentum, which is only conserved up to multiples of inverse lattice vectors. So the two vectors $\vec k$ and $\vec k + \vec G$ (with a inverse lattice vector $\vec G$ can be said to be "coordinates" for the same point in momentum space. In other words, the momentum space of a crystal is, topologically speaking, a $n$-torus. Therefore, we can "fold back" the free electron dispersion to the first Brillouin zone, the new states are then labelled by a "band index" $\nu$ and a vector from the first Brillouin zone $\vec k$, so there are multiple possible energies at each point of the first Brillouin zone! That is the possible energies at point $\vec k$ in the first Brillouin zone are the energies $E_{\vec k + \vec G}$ (for all $\vec G$ from the inverse lattice), because these different $\vec k$ are just coordinates for the same point in momentum space! So the bands arise (more than one energy at each point), because by breaking the continuous translation symmetry, the momentum space is effectively reduces to the first Brillouin zone in the described fashion.
Side note: The 1d case I mention above can be found in probably any introduction to solid state physics. Another useful keyword for finding more about this is Bloch theorem.
An electron having a momentum $k$ in a solid means that its wave function is given by $$ \psi(x) = u_k(x) {\rm e}^{i k x} $$ where $u_k(x)$ is some periodic function with period $a$. The exact form of $u_k(x)$ is given by the Schrödinger equation $$ -\frac{\hbar^2}{2m}\psi''(x) + V(x)\psi(x) = E\psi(x)$$ with periodic boundary conditions $$ \psi(a) = \psi(0), \qquad \psi'(a) = \psi'(0)$$ Even if there is no potential, ($V(x) = 0$, the electrons were truly free), the Schrödinger equation gives us many solutions for any given $k$: $$u_{k,n}(x) = {\rm e}^{i \frac{2\pi n}{a}x}, \qquad n\in\mathbb Z$$ with the corresponding energies being $$E_{k,n} = \frac{\hbar^2}{2m}\left(k+\frac{2\pi n}{a}\right)^2, \qquad n\in\mathbb Z$$ Usually instead of being seen as different solutions for the same $k$, the additional solutions are interpreted as solutions for a different $k'=k+\frac{2\pi n}{a}$ by allowing $|k'|>\frac{\pi}{a}$. However, if you plot different $E_{n,k}$ on one graph for $|k|<\frac{\pi}{a}$, you'll see a familiar band structure.
If the potential is non trivial, we still have a sequence of functions $u_{k,n}$ and sequence of energies $E_{k,n}$, and they are what creates the band structure, but they no longer have such simple form, which is why they cannot be clearly identified as the solutions for $k'=k+\frac{2\pi n}{a}$. However, if you prolong the graph of a band structure periodically, you can note that for small potential the different bands will together form something approximating a series of parabolas - this is a relic of the free case, where the parabolas are exact.
The key word is "nearly".
The electrons are not completely free, so their dispersion relationship is not necessarily parabolic. Furthermore, the potential is periodic. This results in the odd fact that different values of $k$ label exactly the same wave vector.
This answer provides the details, although for a different question.
