0
$\begingroup$

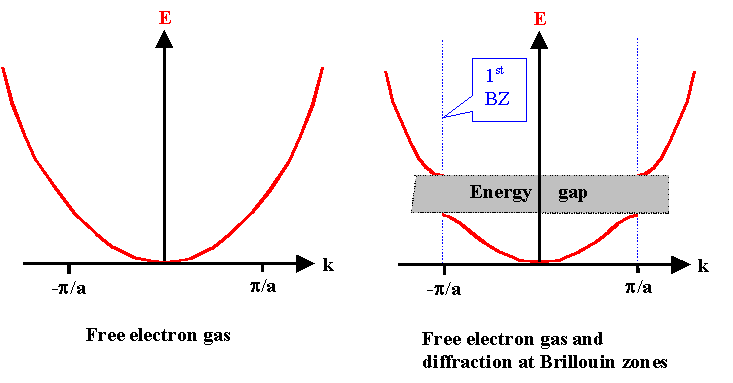
Many introductory books on solid state physics use images that I find confusing. For instance, one picture I see alot when reading about the band gap, is similar to this one:

Band gap

  1. I understand that the band gap originates due to diffraction near the BZ edges and that the standing wave can be "decomposed" into two waves, with an energy difference - an energy spectrum where the Schrödinger eq. has no solution (tell me if I am mistaking here!). Fine. But in pictures like these, the band with the lower energy stops and the band with the higher energy begins at the edge of the zone? Why is it drawn like that? Surely, the higher energy wavefunction also "exists" inside the 1st BZ and the lower energy wavefunction outside the 1st BZ? If so, could someone direct me to a more telling picture and if not, could you please explain why? As I understand it, the band structure pattern is simply repeated for the other zones.

    1. One a more general note, the energy bands are usually plotted against the k-vector. What good does it do to have the energy bands plotted vs. the k-vector? The k-vector is a mathematical tool and I understand its true purpose, but I have a difficulty making a physical interpretation of these plots. If someone could expain the logic behind this, I would appreciate it alot. Thanks.
Improve this question
$\endgroup$
3
  • $\begingroup$ The k vector is far more than a mathematical tool, so I don't think you quite see the true purpose of it. Now, I will also state that the image shown, while not uncommon, is pretty horrible, and the explanation using 'diffraction' is even worse. One should dive in to a better reference, such as Ashcroft and Mermin's Solid State Physics book. The 'gap' is caused by an attempt to overlap the bands at the edge - any electron-electron interaction will lift the degeneracy, lifting one band away from the other. This is just like the phonon-polariton splitting. $\endgroup$
    – Jon Custer
    Commented Jan 15, 2016 at 17:52
  • $\begingroup$ Here's an answer to a related question, which I think might answer this question as well. $\endgroup$
    – garyp
    Commented Jan 15, 2016 at 18:29
  • $\begingroup$ Hello and thank you for your answer! :) I don't have the book by Ashcroft and Mermin. Do you know a site which might explain the band gap in greater detail, as you put it? All the sites I find simply repeat the explanation which is found in Kittel's book etc. Regarding the E(k)-plots: I understand that the wave vector says something about the energy, because the impulse depends on it and also says something about the direction of the wave (from the solution to the wave equation). But I find it tricky to make a physical interpretation of the plots. $\endgroup$
    – user103984
    Commented Jan 16, 2016 at 12:24

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.