Why is work done by normal force 0 here?

Question

In solving this problem from Kleppner and Kolenkow, the (total) work done by the reaction force b/w the cube and the large block is taken to be 0 and I cannot seem to rationalise this.

Clearly, the "normal force is perpendicular to motion" argument doesn't work here as the reaction force is perpendicular to circular path and the cube doesn't follow the circular path in the rest frame. In fact, it is easy to see that the work done by $N$ on the block is positive as its kinetic energy increases and no other force is doing work (there is no motion vertically). It must be, then, that $N$ does an equal negative work on the cube for the total work by $N$ to be $0$. Why is this the case here, and is there some general principle dealing with this?

Answer 1

This answer states the same as @basics, just stated more simply.

Consider two frictionless blocks, $A$ and $B$, which happen to be moving at speeds $v_A$ and $v_B$ under gravity. See picture below. Block $B$ is resting on a frictionless surface as well, but we don't need that for our analysis right now. In this setup, we can see easily that the work per unit time is zero. Because $$\dot W_A=\mathbf {F}_A\cdot\mathbf {v}_A=0$$ and likewise for $B$. But this has to be true for any setup of two solid, touching objects. The reason is that the normal force is perpendicular to the relative velocity of the two bodies. If it weren't, the bodies would either start separating or penetrate each other.

To see this, we can calculate the distance between the two blocks. For this simple geometry, the distance is given by $$d=(\mathbf r_A-\mathbf r_B)\cdot\hat{\mathbf {n}}-d_0$$ where $\hat{\mathbf {n}}$ is the surface normal vector, $\mathbf r_i$ are the centers of mass of the blocks and $d_0$ is the minimal separation, i.e. when the blocks are just touching. If $\hat{\mathbf {n}}$ stays constant, the time derivative is given by \begin{align} \dot d&=(\mathbf v_A-\mathbf v_B)\cdot\hat{\mathbf {n}}\\ &=\mathbf v_{AB}\cdot \hat{\mathbf {n}} \end{align}

So, for the distance between the two blocks to stay constant, the relative velocity needs to be orthogonal to the normal vector. In your problem, the blocks are always touching so this needs to be the case. We can now easily show that the work between the two bodies needs to be zero: \begin{align} \frac{d}{dt}(W_A+W_B)&=\dot W_A+\dot W_b\\ &=\mathbf {F}_A\cdot\mathbf {v}_A+\mathbf {F}_B\cdot\mathbf {v}_B\\ &=\mathbf {F}_A\cdot\mathbf {v}_A-\mathbf {F}_A\cdot\mathbf {v}_B&\text{(Newton's third law)}\\ &=\mathbf F_A\cdot\mathbf v_{AB}\\ &=F_{A}^{n}\ \hat{\mathbf {n}}\cdot\mathbf v_{AB}+F_{A}^{t}\ \hat{\mathbf {t}}\cdot\mathbf v_{AB}\\ &=0 \end{align} where $\hat{\mathbf {t}}$ is a vector tangent to the surface. Because of what we just showed, the normal component is zero at all times. The tangent component is zero because friction is zero. Since the power (time derivative of work) is zero at all times, the total work done is also zero.

Answer 2

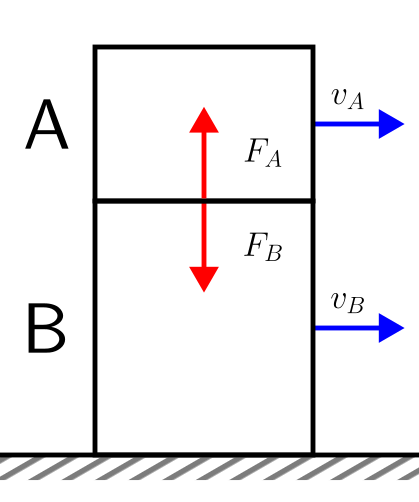
Short answer

No friction and thus ideal internal algebraic constraint provides no net power to the system.

Details

Let's ignore the rotational inertia of the small mass there, so that it's possible to deal only with momentum in deriving the equation for the kinetic energy of the system.

Being the system $S$ composed of several sub-systems (here two, the small mass and the large block) $S_j$.

For each sub-system:

• momentum equation reads: $$\dot{\boldsymbol{Q}}_j = \mathbf{F}^{ext,j} = \mathbf{F}^{ext}_j + \sum_{k\ne j} \mathbf{F}_{jk}$$

• if $K_j = \frac{1}{2} \mathbf{v}_{G,j} \cdot \mathbf{Q} = \frac{1}{2} m_j |\mathbf{v}_{G,j}|^2$ is the kinetic energy of the $j^{th}$ subsystem, the kinetic energy equation reads,

  $$\dot{K}_j = \mathbf{v}_{G,j} \cdot \dot{\mathbf{Q}}_j = \mathbf{v}_{G,j} \cdot \left( \mathbf{F}^{ext}_j + \sum_{k\ne j} \mathbf{F}_{jk} \right) \ .$$

Thus, equations for the whole system $S$ are derived by summing over all the sub-systems (both momentum and kinetic energy are defined to be additive quantities). The kinetic energy equation, using the third principle of Newton mechanics $\mathbf{F}_{jk} = - \mathbf{F}_{kj}$, reads

$$\begin{aligned} \dot{K} = \sum_j \dot{K}_j & = \sum_j \left\{ \mathbf{v}_{G,j} \cdot \left( \mathbf{F}^{ext}_j + \sum_{k\ne j} \mathbf{F}_{jk} \right) \right\} = \\ & = \sum_j \mathbf{v}_{G,j} \cdot \mathbf{F}^{ext}_j + \sum_{ \{j,k \} } \left( \mathbf{v}_{G,j} - \mathbf{v}_{G,k} \right) \cdot \mathbf{F}_{jk} \end{aligned}$$

The first term is the power of external forces, the second term is the power of the internal forces exchanged here between each pair of sub-systems $j$ and $k$, and working for the relative velocity (in general of the application point, here of the center of mass because of the assumption made before to avoid treating unnecessary complications to tread rigid motion here). This latter contribution is identically zero for algebraic constraints since the force is orthogonal to the relative velocity of the bodies.