Work done by friction

Question

Suppose we have a block of mass $M$ and we are moving it up a curve, very slowly ($a=0$). The surface is not smooth, and coefficient of friction is $\mu=\mu_s=\mu_k$.

To move the block we apply a force $F$ to the block tangential to the surface(on which the block is, at that time). If we take the angle of slope to be $\theta$ (of the surface), then the forces acting are friction$f$, gravity/weight $Mg$ and external force $F$.

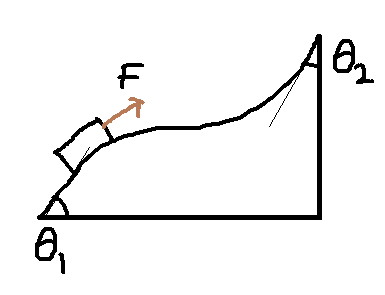
This is where the problem comes. If we want to find out the work done by friction, then $$W_{friction}=\int\vec{F_{friction}}.\vec{dr}=-\mu Mg\int dr cos\theta=-\mu Mg\int dx$$ But when I use the work-energy theorem, then $$W_{friction}+W_{gravity}+W_{ext}=0$$ $$W_{friction}=-W_{gravity}-W_{ext}$$ $$W_{friction}=Mgh-F\int dr$$ When I get the work done by friction using integration then it tells me it doesn't depend on the type of path and path length, but only horizontal $x$ covered. When I use the work-energy theorem, it tells me that it depends on height $h$ till which the block goes and path length $r$. What is going wrong? Could anyone hint me?

Thanks in advance.

Answer 1

Let at any angle the slope of path is $\phi$ :

So, $$-F\int dr =-\left[\int F\cdot\text dr\cos\phi +\int F\cdot\text dr\sin\phi\right];$$

Now as $\Delta K.E.=0;$ (moving slowly);

Net work by horizontal forces = Net work done by vertical forces =0;

Now , you can see vertical work = $\int F\sin\phi\cdot \text dr - Mgh =0$

and horizontal work =$\int F\cos\phi\cdot \text dr $ must be cancelled out by the frictional work , which shows work of friction just depends on horizontal distance. So,The first method was right and second one was not completed.