Is the normal force a conservative force?

Question

Most of the time the normal force doesn't do any work because it's perpendicular to the direction of motion but if it does do work, would it be conservative or non-conservative?

For example, consider the following block-incline system where the incline is on a friction-less surface. Now as the block moves down, the incline itself starts moving in the opposite direction. Here, the normal force acting on the incline by the block does do work.

This question was on some website (it asked something about the final velocities of the block and the incline) and they solved this using mechanical energy and momentum conservation which confused me as I couldn't understand why the normal force here is conservative.

So,

1. Is the normal force always conservative or is this only in some cases?
2. If yes, how do we deduce that like in the above example?
3. Also, if it is conservative, what would be its corresponding potential energy function?

Answer 1

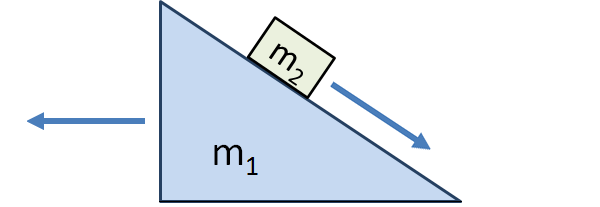
The normal force acting on the incline by the block does do work, but the normal force acting on the block by the incline does negative work, and the total work done by all normal forces in the system is zero (edit: see below for proof).
Therefore, the normal force can be considered a "constraint force", i.e. a force that does no work and is neither conservative nor non-conservative.
The work vanishes only when looking at all the normal forces in the system, since the normal force acts here as a mediating force, transferring the gravitational force from the block to the incline.

This example may be confusing since there are additional forces in different directions, consider the simpler setting of a force pushing two blocks on a horizontal plane:

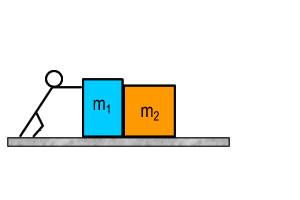
Here the left block applies a normal force to the right block and vice versa, and again the total work done by the two normal forces cancels, since the normal force mediates the pushing force between the left block and the right block.

Another interesting example is the tension force of a string holding two weights over a pulley:

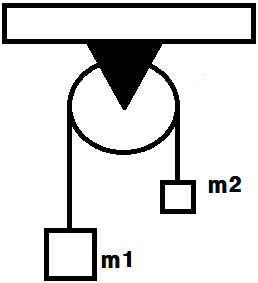
In this system the string pulls the lighter mass and does work on it, but it does negative work on the heavier mass and so the total work the tension forces do is zero. The string acts as a mediator that transfers the gravitational force between the two blocks.

Edit - corrected proof (credit to @DSinghvi for pointing out the error in the previous version of the proof in the comment below):
Here's how we can see the work done by the two normal forces cancel (and this proof can be easily generalized to any other problem with normal forces):
According to Newton's second law, the force acting on the incline by the block, $\mathbf{N}_{bi}$, is equal in size and opposite in direction to the force acting on the block by the incline, $\mathbf{N}_{ib}$, i.e.: $$ \mathbf{N}_{bi} = - \mathbf{N}_{ib}. $$ In the axis parallel to the normal force, the incline and the block move together, so if the incline travels an infinitesimal distance of $dx$, then the block at the same time travels the same distance $dx$. The total work done by both forces while this distance is traveled cancels: $$ N_{bi} dx = - N_{ib} dx ~~~\Rightarrow~~~ N_{bi} dx + N_{ib} dx = 0 $$

Answer 2

Normal force are reaction forces on solid things, like walls, and are perpendicular to this: If $dx$ has some perpendicular component to the surface, it must be outwards (it can't go through the surface), so the normal force dissapears as it stops touching the surface. If the normal always exists the object must move on the surface, then $dx$ is perpendicular to $N$ and the work made by that force is always 0. That means that: $$\oint_C Ndx=0$$ For every closed path $C$, as actually it will be 0 for every path, so it's conservative, but not very interesting as the work is always 0.

In moving reference systems, the normal is coupled to the force that keeps the object on the surface, so the work will be the same as the one by the force that keeps the object on the surface. It will be conservative if this force is.