1
$\begingroup$

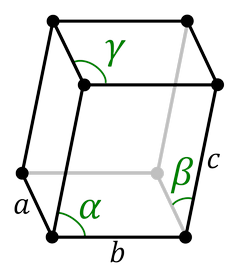
When classifying the Bravais lattices we have the triclinic (point group ${\rm C_i}$) and the monoclinic $({\rm C_{2h}})$ cases, but we do not see the "biclinic" case listed. Why not?

It seems that of the three angles involved (see image of triclinic case) there could quite well be just one right angle and two of them different from $90^\circ$. Does that not happen in nature? To be complete, we do have the "zero case" of orthorombic crystals, where none of the angles differs from $90^\circ$, but the case with two just seems to be missing...

Triclinic

Improve this question
$\endgroup$

1 Answer 1

Reset to default
3
$\begingroup$

Having a single right angle still makes it a triclinic crystal as all three angles are different. It does not increase the symmetry of the crystal.

Improve this answer
$\endgroup$
4
  • $\begingroup$ So it can occur, but the fact that one angle happens to be 90$^∘$ is not special enough to get its own name... Although we actually do have a special name for the case where all angles are of equal value when that value happens to be 90$^∘$, which is then called orthorombic instead of monoclinic. (Presumably because the supplementary angles are always present as well but in the orthorombic case they are no longer of a different value). $\endgroup$
    – Jos Bergervoet
    Commented Apr 13 at 10:47
  • $\begingroup$ @JosBergervoet Yes, because I believe it doesn't change the symmetry group and therefore isn't a different Bravais lattice type. Orthorhombic is genuinely a different crystal as it has more symmetries. We only give different names to lattices with different symmetries. $\endgroup$
    – Vincent Thacker
    Commented Apr 15 at 16:20
  • $\begingroup$ That's what we do in the classification of Bravais lattices. But even though we do this, the fact still remains that there can be special properties of a lattice that do not affect its symmetry, like having (as discussed) one right angle and two non-right angles in its basis. So I'm still wondering: in the (perhaps rare) cases when we're interested in that (relatively unimportant) property, would it be appropriate to call that case "biclinic", or is there already a different name in existence? $\endgroup$
    – Jos Bergervoet
    Commented Apr 16 at 7:00
  • $\begingroup$ @JosBergervoet I haven't seen that term being used. A Google search only brings up a couple relevant results. Maybe it's because it doesn't occur in nature. Because from the perspective of symmetry, there is nothing that makes having just one right angle more privileged than other triclinics. 90° is as good as any other angle and there is no reason for nature to prefer it more. Only when we have two right angles do we get more symmetry (monoclinic). $\endgroup$
    – Vincent Thacker
    Commented Apr 16 at 20:13

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.