In hadron spectroscopy, a structure may be interpreted as various "configurations", such as conventional quark-antiquark states, tetraquarks, hybrid states, dynamically generated states or molecular states, and so on.
What is the meaning of dynamically generated states here?
My rough understanding is that (in this context) "dynamically generated states" are those that cannot simply be described as simple quark model hadronic states, but whose existence and properties instead depend on dynamic interactions between those hadrons.
The theoretical framework is that these dynamically generated states are solutions to a scattering (e.g. Lippmann-Schwinger) equation for a given Hamiltonian $H_0+V$ where $H_0$ is the Hamiltonian of the free particles in the model and $V$ is the potential that determines the dynamics of the interactions between those particles. (See, for example, Dasiuke Jido's 2011 paper and slides on "Baryon resonances as dynamically generated states in chiral dynamics".)
In principle, all hadronic states are dynamically generated in Quantum Chromodynamics from quark and gluon fields, but since QCD is so hard to solve, the quark model used to define the hadrons that form $H_0$ and some effective theory (e.g. chiral perturbation) used for $V$. In such models, the interactions between hadrons produce additional "dynamically generated states" outside the quark model.
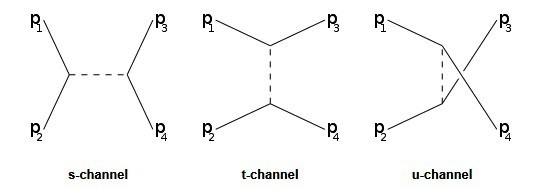
For example, in meson-baryon scattering, resonant $qqq$ states can be formed in the s channel where the initial state particles disappear forming a new meson which lives for $\sim10^{-23}$ seconds and then decays into two (or more particles). In the u- or t- channel, the particles interact over a similar timescale and can dynamically form states that depend on meson-baryon - not quark-quark - forces.
For example, the $\Lambda(1405)$ has confused people for over sixty years. It is too light to be understood as a simple quark model $uds$ excited state and can only be explained by including $KN$, $\pi\Sigma$, … dynamic interactions. Things are messy with quark-model states mixing with dynamically generated states.
