Physical interpretation of Mandelstam variables

Question

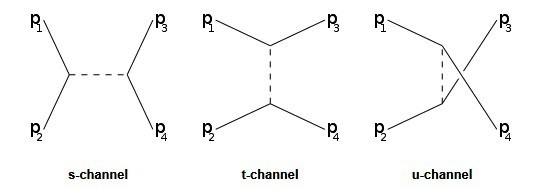
In $2-2$ scattering, the Mandelstam variables $s$, $t$ and $u$ encode the energy, momentum, and angles of particles in a scattering process in a Lorentz-invariant fashion.

$$s=(p_{1}+p_{2})^{2}=(p_{3}+p_{4})^{2}$$ $$t=(p_{1}-p_{3})^{2}=(p_{2}-p_{4})^{2}$$ $$u=(p_{1}-p_{4})^{2}=(p_{2}-p_{3})^{2}$$

where $p_1$ and $p_2$ are the four-momenta of the incoming particles and $p_3$ and $p_4$ are the four-momenta of the outgoing particles.

---

How is $s$ is the square of the center-of-mass energy?

How is $t$ the square of the four-momentum transfer?

What is the physical interpretation of $u$?

Are $s$, $t$ and $u$ related to the $s$-channel, $t$-channel and $u$-channel respectively?

Answer 1

Yes, they are related to the $s,t$ and $u$ channels as you say. Consider a scattering process where the incoming particles have four-momenta $p_1$ and $p_2$ while the particles that go out have momenta $p_3$ and $p_4$. Then, diagrammatically, there are three possible way the process can take place, described by the figure below.

• $s$-channel: The two particles "merge" into a virtual intermediate particle that finally splits into the two final particles. Note that since four-momentum is conserved at each vertex we have for the momentum $q$ of the intermediate particle $$q^2=(p_1+p_2)^2=s$$ Besides, since the four-momenta are $p_i=(E_i,\vec{p}_i)$ and the center of mass frame is defined by the constraint $\vec{p}_1=-\vec{p}_2$ one has $$s=(p_1+p_2)^2=|(E_1,\vec{p}_1)+(E_2,\vec{p}_2)|^2=|(E_1,\vec{p}_1)+(E_2,-\vec{p}_1)|^2=|(E_1+E_2,\vec{0})|^2=E^2$$ where $E$ is the total energy of the two particles in the c.o.m. frame.

• $t$-channel: The particle $1$ emits a virtual particle and in doing so it turns into particle $3$. The virtual particle is absorbed by the particle $2$, that as a consequence of this interaction turns into particle $4$. Since the first particle had momentum $p_1$ before the emission and particle $3$ has momentum $p_3$, the difference of these must have gone into the emitted virtual particle, that thus has (squared) momentum $$q^2=(p_1-p_3)^2=t$$ Note that by reasoning in the same way about the absorption by the particle $2$ we find also that $$q^2=(p_4-p_2)^2=t$$ We can say then that the particle $1$ has "lost" the momentum $q$, that has been transferred to the particle $2$.

• $u$-channel: As you can see from the image, the $u$ channel is analogous to the $t$ channel with the roles of $p_3$ and $p_4$ interchanged. Therefore we can still interpret $u$ as the momentum transfer from particle $1$ to particle $2$.