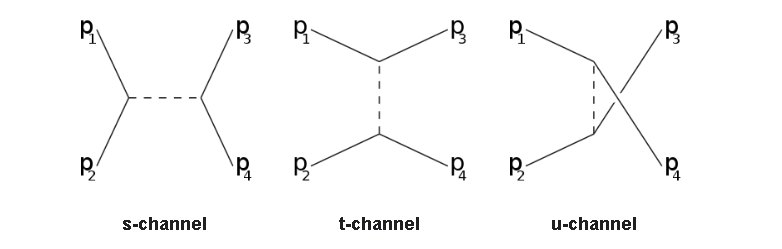
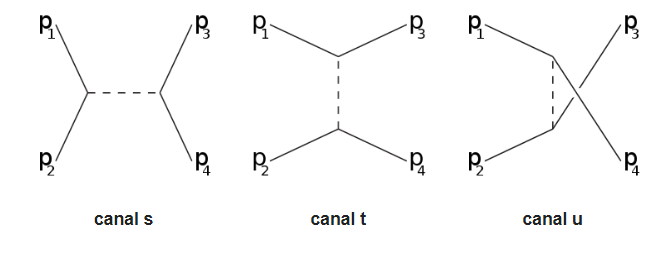
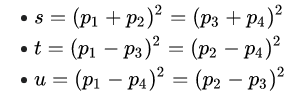As you said they name is totally related with the diagram, since the i-variable is simple the sum (+) of the momentum that go to an annihilation minus (-) the momentum that are created from a vertex the i-diagram, as we can easily see from:
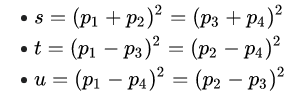
From where wee see that for those concrete diagrams:
s-channel: The two particles "merge" into a virtual intermediate particle that finally splits into the two final particles. Note that since four-momentum is conserved at each vertex we have for the momentum q of the intermediate particle $q^2=(p_1+p_2)^2=s=E^2$ where E is the total energy of the virtual particle interchanged (doted lines).
t-channel: The particle 1 emits a virtual particle and in doing so it turns into particle 3. The virtual particle is absorbed by the particle 2, that as a consequence of this interaction turns into particle 4. Since the first particle had momentum p1 before the emission and particle 3 has momentum p3, the difference of these must have gone into the emitted virtual particle, that thus has (squared) momentum $q^2=(p_1−p_3)^2=t$. We can say then that the particle 1 has "lost" the momentum q, that has been transferred to the particle 2.
u-channel: As you can see from the image, the u channel is analogous to the t channel with the roles of p3 and p4 interchanged. Therefore we can still interpret u as the momentum transfer from particle 1 to particle 2.
But, and here goes what you are asking, once you defined them in the way we said above, they remain the same for all the other cases, and each one gets a physical general intuition that works for any diagram, even when the doted line from where we originally defined it is not there.
Taking this general picture we can see it clearly:
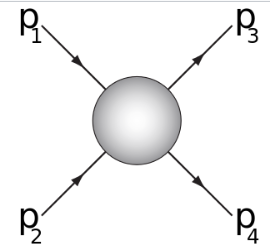
The s-variable will be the total energy of the interaction in the mass center, which means that for any reference frame you compute it with different $p_1$ and $p_2$ the result will be the same (Actually this is the principal motivation to use them, that they are Lorentz invariant), and concretely the result will be the energy of the CM frame, to show it let's start with:
\begin{equation}
(p_1+p_2)^2 = p_1^2+p_2^2 + 2p_1p_2= E_1^2 - |\vec{p}_1|^2 + E_2^2 - |\vec{p}_2|^2 + 2(E_1E_2 - \vec{p}_1\vec{p}_2)
\end{equation} and now since in that reference frame the $\vec{p}_1= -\vec{p}_2$, we get:
\begin{equation}
(E_1^2+E_2^2+2E_1E_2) -|\vec{p}_1|^2 - |\vec{p}_2|^2 - 2 \vec{p}_1(-\vec{p}_1) = (E_1+E_2)^2
\end{equation} where $E_1+E_2$ is the total energy in the CM frame, which also can be thought of as the "mass" of the interchanged particle since in the CM frame would be still, but this also works for diagrams where there is not a dotted line in the middle, so its better to think of it more genereally as the total energy of the system in the CM, you see?
The t and u variables diagrams are less intuitive (they both are the same changing the $p_3$ leg with $p_4$), but also you can see that they represent the four-momentum$^2$ that has been interchanged in the interaction, even for diagrams where there is not a direct interaction as in the originals where they were defined, generally they will be the momentum that goes from $p_1$ into $p_2$ during the diagram ocurs! (Also it is the same for any reference frame).