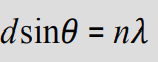Where does the diffraction equations work and why?

Question

w = fringe spacing
$\lambda$ = wavelength
D = horizontal distance between slits and screen
s = slit spacing

d = slit spacing
$\theta$ = angle between central maximum and the ray at the nth maximum
$\lambda$ = wavelength

I am an A Level physics student in the UK. The book I am referring to later on is the 'Advanced PHYSICS' 2nd edition by Steve Adams and Jonathan Allday.

I am confused about where the above equations work? Any number of slits or only double slits or some combination? What about theta? Does it have to be small, or can it be large (greater than 10 degrees)? I know that in both cases the slit spacing should be a lot smaller than the horizontal distance, but I am confused because the bottom equation is used for diffraction gratings, but the derivation was using the young's double slit experiment. However, the top equation was also derived using double slits in the book, yet I have never seen it being used for diffraction gratings. I tried to look for similar answers, but they were too detailed and a bit difficult to comprehend. So, I would appreciate a concise answer.

Answer 1

Answering in order, The fringe width equation works when:

• $d \ll D$
• $\theta$ is also small

The second works when:

• $d \ll D$

Both are derived for the double slit experiment but can be modified for the single slit experiment.

The diffraction equation is not the same as the second, it has a minor difference, that being that the path difference between the topmost and bottommost rays being $n \lambda$ is the condition for minima, not maxima. The only exception is the central maxima, which as a consequence is twice as wide.

For diffraction, according to wave theory, every point on the wavefront acts as a source. For illustration purposes, consider the first minimum and consider $300$ rays. While solving, $d$ is considered to be the width of the slit itself. Now we can apply the double slit equation on each pair of secondary sources along the slit. The resultant intensity is the sum of all these intensities.

If the path difference for ray $1$ and ray $300$ is $\lambda$, these two interfere constructively, but this is the only maximum. Ray $1$ and $151$ form a minimum, so do $2$ and $152$ and so on. Overall, this shows as a minimum since an overwhelming majority of the pairs result in destructive interference.

Now for a maximum, it occurs at path difference $1.5 \lambda$ between ray $1$ and $300$. In this case, ray $1$ and $100$ form a maximum and so on. However note that due to there being a large number of rays destructively interfering, overall intensity is low. Due to this, as $n$ increases, overall intensity also decreases.

Now for the last question about number of slits, any number can be used, just take them pairwise and solve as if it is a double slit. The resultant pattern will be due to their sum.

Just a disclaimer: I'm not saying there are actually $300$ rays but it helps to visualize.