How does the "York time" measure the expansion of space; why is it equal to the divergence of the comoving observer's four velocity for warp drive?

Question

The mysterious York time, θ is important in warp drive topic. It is plotted on the famous diagrams and is considered the measure of the mechanism that "drives" the warp drive bubble at superluminal speed. However, the basic articles do not explain in detail what it is derived from and how it measures the expansion and contraction of space. In reading another question I came across:

1. on the one hand, the expansion and contraction of space is expressed by a rather complicated expansion tensor. This can be derived from the Raychaudhuri equation which requires a deep knowledge of the mathematics of general relativity. https://en.wikipedia.org/wiki/Raychaudhuri_equation
2. On the other hand, for warp drive, the expansion and contraction are given by a relatively simple formula called "York time". In addition, the papers state that York time = the covariant divergence of the Eulerian observers four velocity. So it is extremely important; in the end, the formula only includes the velocity and shape functions. Alcubierre-Lobo

My questions:

• can someone explain how the two are related? I am thinking that maybe York time is equal to the trace of the expansion tensor. But I don't see exactly the relationship between them, and it is not clear from the basic articles on warp drive why it is equal to the divergence of the Eulerian observers four velocity. No more detailed derivation is given, unfortunately, although it is astonishing: the four velocity is a feature of a worldline, a tangent vector. And the expansion and contraction of space seems to be another matter entirely.
• Am I correctly assuming that it is not just the U four velocity of a particular worldline, but all such worldlines that pass through the points of the bubble, as well as, before and after it?

Notes: Alcubierre introduces the measure of expansion in a slightly different way in his first article opening the warp drive research. Here as a trace of the extrinsic curvature tensor. It is a slightly more understandable starting point, but how does the later derivation based on four velocity lead to the same formula? And would it make any difference whether we work with the trace of the extrinsic curvature tensor or the expansion tensor?

Alcubierre

Thank you very much if someone can give me some clarification on this fascinating issue.

Answer 1

Let $U^{\mu}$ be the tangent vector field to a four-dimensional timelike geodesic congruence; for instance the four-velocity field of some pressureless fluid, with an observer in the center. To observe how this congruence evolves we set up a set of three normal vectors orthogonal to our timelike geodesics. The failure of this set of vectors to be parallel-transported will tell us how nearby geodesics in the congruence are evolving. Equivalently, we can imagine a small sphere of test particles centered at some point, and we want to describe quantitatively the evolution of these particles with respect to their central geodesic. Defining an operator projecting to the space normal to $U^{\mu}$ it is natural to introduce the quantity

$$\theta = \nabla_{\mu} U^{\mu}$$

which measured the rate at which the volume of a small ball of matter changes with respect to time as measured by a central comoving observer. This is the most generic definition. It is well covered in Carroll general relativity book Appendix F, which you should check for more background.

Equivalently, if we are interested in Eulerian observers (moving only trought time), which is our case, we can use the 3+1 formalism and look at the extrinsic curvature of the 3-space. The latter gives you the rate of change of the projection tensor as we move along the normal vector field, so you recover

$$\theta \propto K $$

where $K$ is the trace of the extrinsic curvature tensor $K_{ij}$.

To my understanding the York time is another name for the expansion scalar $\theta$, but it is a rarely used terminology overall (I believe it was introduced by H White), so I would advise to simply use the expansion scalar terminology instead.

Answer 2

I will write an answer to my question. @Rexcirus 's answer and the source he provides are excellent clarifications of the mathematical implications of my original question, the characterization of expansion and York time. I will briefly summarize this based on the book, please comment if I understand it correctly. And although the mathematical details of the thought process are clear enough, there are important questions about their meaning, their physical content, which I will then address - mainly that the expansion and contraction of 3d space is a common, general phenomenon in the analysis of spacetime, but is not usually addressed? A RELATIVIST’S TOOLKIT

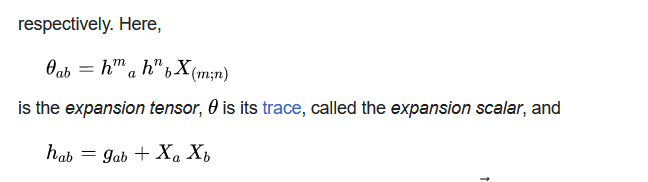
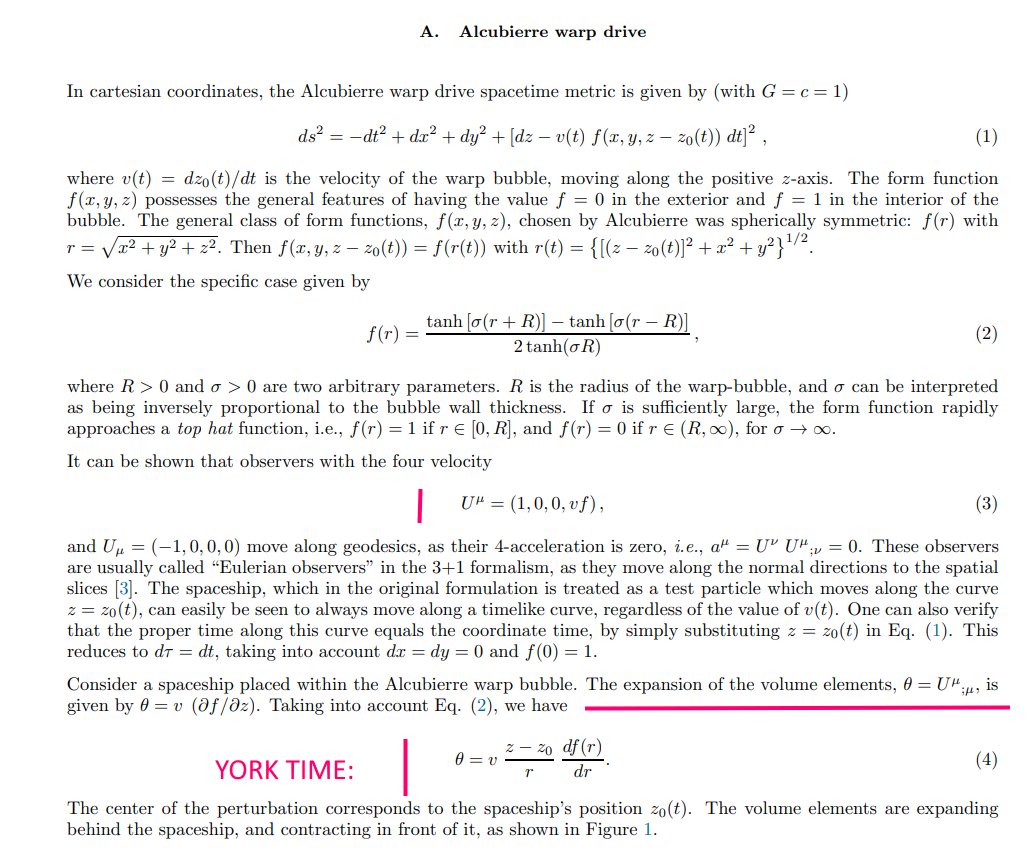
So: A) To characterize the expansion, we indeed start from the obvious formula for the change of the 3d volume (the first formula with red line in the picture). For this we form the expansion tensor 2.17, which characterizes it in several ways, the most important one being perhaps the expansion scalar θ. The tensor is the covariant derivative of the four velocity vector field associated with the geodesic congruence, and the θ is its trace. This, as the next line states, is the same as the divergence of the four velocity - so this is where the formula used in the warp drive articles appears. That the θ expansion scalar does indeed accurately measure the expansion of space, and not just intuitively introduced its formula, is deduced later in a subsection, but I have not put it on the picture. So that's the answer to my original question, and that's how the York time formula I asked about comes about. (The strange name "York time" for the expansion scalar is rare, but it is applied to it by others for that reason, not just Harold White.) If the formula for the Uμ four velocity vector field is relatively simple, and the metric tensor is not too complicated, then the formula for θ will be a relatively simple expression, as it is for the Alcubierre warp drive. The extrinsic curvature tensor, K, comes into play when a spacetime is handled with the 3+1 ADM foliation; in this case, the θ will be proportional to its trace, but so will the divergence of the four velocity. Alcubierre and other researchers have developed warp drive models in this way, of course we are not bound to apply 3+1 fromalism to them. Two important examples are presented in the book, the well-known cosmological spacetime and the outer part of Scwarzschild spacetime; in both of them it is nicely shown what kind of θ is derived from the four velocity of the geodesic.

So the short point is that θ is indeed a measure of the volume change of 3d space and can be calculated from the four velocity of the geodesics. And the focusing theorem states that if the strong energy condition holds for a part of a spacetime, then the θ so calculated will be negative,ergo the geodesics converge and 3d space contracts. (It cannot be positive, it may be 0, then it remains constant.) This is consistent with the usual gravitational effects being attractive.

But thinking about the above, the picture is not so reassuring, since the question naturally arises: all spacetimes have geodesics, including local ones, and even the much-analysed spacetimes, such as those used to describe black holes or stars. And for every geodetic congruence there is a four velocity field, ergo at every point in all spacetimes one can calculate the θ described above. Which measures the expansion of 3d space. So why don't we see this often, in known metrics, solutions, models? But it is a very important question, whether the space expands or contracts. And I almost only encountered it in cosmological FLRW spacetime and warp drive. As a special phenomenon - and it should be as general as the geodesic field is general. Or is it not calculated and described for the other spacetimes because it equals 0 for some reason? Only in these two is it not zero? But what would cause this?

Let's take a look at the local case, Schrwarzschild spacetime, also shown in the picture. It computes a special case of geodesics: radial, timelike and marginal bounded geodesics. These will be of two types, outgoing and ingoing, both with non-zero θ, given by the formula at the bottom of the picture.
Excellent, this proves the previous question right, since the expansion rate, θ, can be calculated in the most studied local spacetime, and will not be 0. So for this and all other known spacetimes, we should calculate and analyze what happens to the volume of space. One could say, if I am right, that well, but in this Schwarzschild case you can see that the outgoing geodesics and the ingoing geodesics show the same θ but with opposite signs, so you still end up with 0 for the two together. Okay, but this is far from being all geodesics in Schwarzschild spacetime, it's just a pretty special kind. And if we looked at all of them, not just the radial and marginally bound geodesics, what would we get? What is the space doing, is it expanding to stay the same volume, or is it contracting? Does observing geodesics that are not so specific also give two types , which cancel each other out? It would take a lot of luck, I think. Also, within the event horizon, there are no longer two types of timelike geodesics, but only ingoing. There, the previous cancellation with opposite sign certainly no longer works. Then why is it not part of the standard analysis and body of knowledge that the volume of the Schwarzschild black hole is contracted by θ = …..(some formula)?