3
$\begingroup$

I'm trying to understand how to calculate proper spacelike volumes of metric tensors that have off-diagonal terms. Right now I'm considering a slower-than-light Alcubierre metric:

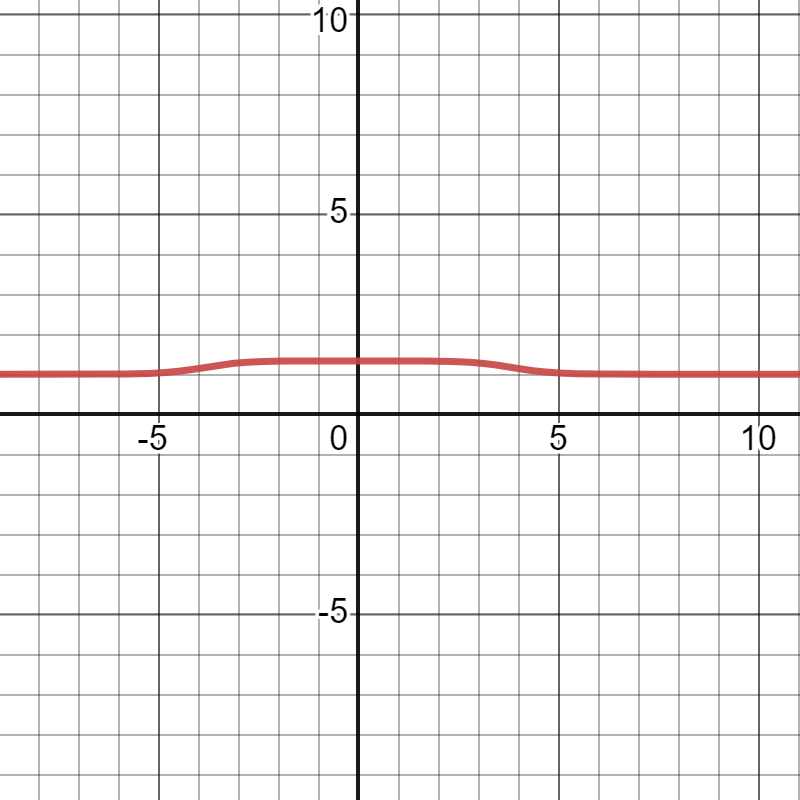
$ds^2=(v^2f^2-1)dt^2-2vfdtdx+dx^2$, where I'm making $v=0.5$. Also, I'm considering $t=0$, so $f=\frac{tanh(x+4)-tanh(x-4)}{2tanh(4)}$.

Switching to a new time coordinate $d\tau=dt+\frac{vf}{1-v^2f^2}dx $ makes the determinant of the 3-metric $\frac{1}{1-v^2f^2}$, which has this graph:

enter image description here

However I'm not sure about this because I would have expected the volume elements inside the bubble to be smaller than those out, since they are contracted on one side and restored to normal size on the other end. Would this assumption be correct?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

Usually inside the bubble $f = 1$ and the space is Minkowskian, so space is completely flat.

Also, perhaps you could be more interested in a coordinate invariant statement such as the expansion $θ = \nabla_{μ} U^{\mu}$ which gives $$ θ=v \frac{x−x_s}{r} \frac{df(r)}{dr} $$

where $x_s$ is the center of the bubble or position of a ship. That is what is drawn in the original paper.

Improve this answer
$\endgroup$
12
  • $\begingroup$ But wouldn't it still be different from the minkowskian space outside the bubble, where f is 0? I would assume so because this would make the metric different. $\endgroup$
    – user345249
    Commented Sep 6, 2022 at 5:00
  • $\begingroup$ Also, are you saying that taking the integral of $\theta$ would yield a better answer to the question than a new time coordinate would? $\endgroup$
    – user345249
    Commented Sep 6, 2022 at 5:06
  • $\begingroup$ Regarding the first comment, f=1 is minkowski, you just need a change of coordinates to redefine a new X = x - x_s(t) to see that, and using the fact that v = dx_s/dt. $\endgroup$
    – Rexcirus
    Commented Sep 6, 2022 at 9:52
  • $\begingroup$ 𝜃 is the expansion (scalar), not a coordinate. See en.wikipedia.org/wiki/Raychaudhuri_equation $\endgroup$
    – Rexcirus
    Commented Sep 6, 2022 at 9:53
  • $\begingroup$ Yes indeed, not a coordinate. I was just thinking that that if $\theta$ is the rate of change of the curvature per coordinate time (although I'm not sure that's what it always is), integrating it could somehow give the curvature at a single moment. $\endgroup$
    – user345249
    Commented Sep 8, 2022 at 19:05

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.