I've wondered about this for ages. If we create a pair of flywheels that rotate in the opposite direction with the same angular momentum, but are co-located and have the same mass and inertial moment (one can imagine various ways to accomplish this, at least approximately) -- it is clear to me that there cannot be any precession forces, but, if we try to rotate the entire assembly around an axis perpendicular to the axis of flywheel rotation, will the force needed to produce this secondary rotation be the same as if the flywheels were stationary, or will it require a proportionally greater force to rotate in this way, as it does with a single flywheel?
2 Answers
two concentric and counterrotating flywheels preclude all precession forces regardless of which plane the axis is rotated in. this is assuming the connection between the two flywheels is sufficiently strong--it make break from tension/compression due to each flywheel experiencing its own forces.
refer to the diagram i just drew up.
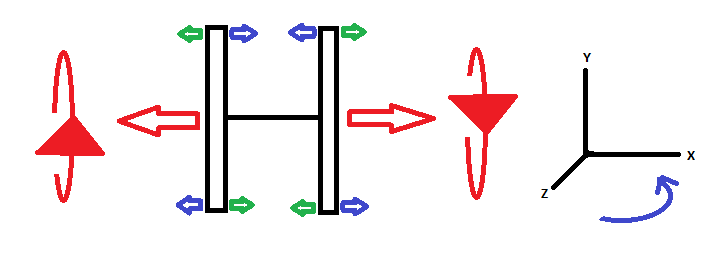
the black rectangles are the two flywheels, the connecting line is the physical connection and also the axle which both flywheels are concentric. the red arrows show the direction of angular momentum (along x axis), while the red circles indicate the direction of rotation (around x axis).
the blue arrows indicate the precession forces experienced by both flywheels when the whole system is rotated in the direction indicated by the curved blue arrow. this is what causes tension/compression in the connecting bar, but otherwise zero torque on the system as a whole.
the green arrows indicate the same forces if the system was rotated the other way (counter to the blue curved arrow).
the situation is similar for rotation of the system in any other plane.
-
1$\begingroup$ Thanks for the diagram! I agree as in my post that there will be no precessional forces, as they cancel each other out in the two wheels. My question is different -- I suspect that it will still require more force to rotate the entire assembly in orthogonal directions than it would take if the flywheels were at rest. I believe the flywheels' motion increases the angular inertia of the system overall, and I'm looking for some explanation of why that is or is not the case. $\endgroup$ Commented Oct 5, 2013 at 16:26
-
1$\begingroup$ hmm doubt it. i forgot to add that since the flywheels are counter rotating, their angular momentum vectors cancel nicely. ie, the system has 0 angular momentum. it will still take more force to torque the whole bit as a system, but only because it has more mass. $\endgroup$– gregsanCommented Oct 5, 2013 at 16:43
-
1$\begingroup$ Well, there is a relativistic correction: the whole assembly is effectively more massive than it was with the wheels at rest, but that enters at the level of $m(\omega r/c)^2$. An effect more likely to be detected is that the frame may flex slightly when you first begin turning the device: be sure to build it strong because otherwise you are constructing a bomb powered by the rotational kinetic energy of the wheels. $\endgroup$ Commented Oct 5, 2013 at 17:15
-
2$\begingroup$ Not looking for X2 because 2 flywheels, or anything close to relativistic. I'm talking about how, when you have a single gyroscope, and you attempt to rotate it around an axis 90 degrees from its rotational axis, and you resist the precession force that occurs, you STILL find that it is as if you were trying to rotate something much more massive than the wheel. I am pretty sure that my counter-rotating assembly will show the same effect, without the precession force. But to date, no one has satisfactorily addressed this issue (as far as I have seen) $\endgroup$ Commented Oct 7, 2013 at 9:02
-
1$\begingroup$ that's why you use a second, counter rotating flywheel so the both precession forces cancel each other on the system level. this precession force doesnt oppose your applied torque (because it is orthogonal). it changes its direction--that gives it the illusion that it is harder to torque, a phenomenon that disappears when there are two flywheels. any perceived increased difficulty to torque the system would simply be due to there being twice as much mass and therefore moment of inertia. $\endgroup$– gregsanCommented Oct 8, 2013 at 7:08
I think that in case of two flywheels the angular momenta point in opposite directions and thus cancel each other. Therefore no nett torque will be required to change the angular momentum of the pair which is not the case if there is only one flywheel.
-
3$\begingroup$ "Therefore no torque will be required" Well, the frame of the device is required to supply quite significant torques to the two wheels, it is just that these cancel out. Better to say "no net torque". $\endgroup$ Commented Oct 5, 2013 at 17:11
